Home Page Textbooks Physics Page 105: Assessment

Physics

1st Edition

Walker

ISBN: 9780133256925

Textbook solutions

All Solutions

Page 105: Assessment

Exercise 63

Step 1

1 of 2

In this problem, we explain why the units of acceleration is $mathrm{m/s^{2}}$.

Step 2

2 of 2

First, we find the units for velocity. Velocity is the rate of change of displacement, so its units is in $textbf{m/s}$. Acceleration is the rate of change of the velocity, so its units must be $dfrac{mathrm{m/s}}{mathrm{s}} = dfrac{mathbf{m}}{mathbf{s^{2}}}$

Exercise 64

Step 1

1 of 2

In this problem, we see if rounding a corner can be done with constant speed and with constant velocity.

Step 2

2 of 2

For the constant speed, it is $textbf{possible}$, since rounding a corner only needs to change the direction of motion. For the constant velocity, it is $textbf{not possible}$, since rounding a corner means that the direction of motion is always, so the velocity is not constant.

Exercise 65

Step 1

1 of 2

In this problem, the breaks of a train create a constant deceleration regardless of the initial speed. If the speed is doubled, we find what happens to the time required to stop.

Step 2

2 of 2

The acceleration is constant. The final velocity must be $0$, so we have

$$
begin{align*}
v_text{f} &= v_text{i} + at \
0 &= v_text{i} + at \
t &= left( -frac{1}{a} right)v_text{i} \
implies t &propto v_text{i}
end{align*}
$$

The time before stopping is proportional to the speed before hitting the breaks. Since the speed is doubled, the time must also be $textbf{doubled}$.

Exercise 66

Step 1

1 of 2

In this problem, we see if a ball with zero velocity can have a positive acceleration.

Step 2

2 of 2

The answer is $textbf{yes}$. Consider the downward direction to be positive, and a ball was initially thrown upward. At the peak of the trajectory, the velocity is zero, but the acceleration is downward (positive). The scenario is possible.

Exercise 67

Step 1

1 of 2

In this problem, we see if a ball can have positive velocity but negative acceleration.

Step 2

2 of 2

The answer is $textbf{yes}$. Consider the upward direction to be positive. A ball thrown upward has a positive velocity, but its acceleration is negative because the acceleration is downwards.

Exercise 68

Step 1

1 of 2

In this problem, an object has a position-time graph that is a straight line with negative slope. We find if its acceleration is positive, zero, or negative.

Step 2

2 of 2

The position-time graph has a constant slope, so the velocity must be constant. The acceleration is the rate of change of velocity. The rate of change of a constant is zero, so the acceleration must be $textbf{zero}$.

Exercise 69

Step 1

1 of 2

In this problem, we sketch the velocity-time graph of an object with negative initial velocity but positive acceleration.

Step 2

2 of 2

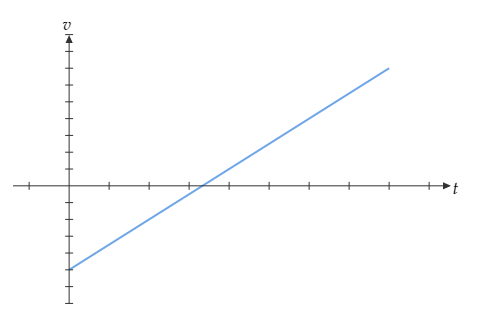
The initial value is negative, but the slope is positive. The graph is Exercise scan

Exercise 70

Solution 1

Solution 2

Step 1

1 of 2

Assume east as a positive direction and west as negative direction. The jet comes to rest in $t=13:s$, so it’s final velocity is: $v_f=0$. Write the velocity equation:
$$v_f=v_0+at$$
Substitute the values given in the task (along with $v_0=115:frac{text{m}}{text{s}}$):
$$0=115+acdot13$$
Solve for $a$:
$$a=-frac{115}{13}$$
$$boxed{a=-8.85:frac{text{m}}{text{stextsuperscript{2}}}}$$
Since the sign of the acceleration is negative, it’s direction is west (opposite of jet’s direction of movement).

Result

2 of 2

$a=-8.85:frac{text{m}}{text{stextsuperscript{2}}}$ westward.

Step 1

1 of 2

$$
textbf{Concept:}
$$

Average acceleration is calculated by dividing velocity by the elapsed time. Assume that east is in the positive direction

$$
textbf{Solution:}
$$

$$
a_{av}=frac{v_f-v_i}{Delta t}=frac{0-115m/s}{13.0s}=color{#4257b2}boxed{bf -8.85m/s^2}
$$

Result

2 of 2

$$
a_{av}=-8.85m/s^2;towards,west
$$

Exercise 71

Step 1

1 of 3

In this problem, a runner accelerates from rest ($v_text{i} = 0$) at $a = 1.9~mathrm{m/s^{2}}$ for $2.2mathrm{s}$. We calculate the runner’s speed after $t = 2.0~mathrm{s}$ after she starts running.

Step 2

2 of 3

We have the following equation

$$
begin{align*}
v_text{f} &= v_text{i} + at \
&= 0 + left( 1.9~mathrm{m/s^{2}} right) left( 2.0~mathrm{s} right) \
v_text{f} &= boxed{ 3.8~mathrm{m/s} }
end{align*}
$$

Result

3 of 3

$$
v_text{f} = 3.8~mathrm{m/s}
$$

Exercise 72

Step 1

1 of 3

In this problem, a skier starts from rest ($v_text{i} = 0$) and accelerates down a slope at $a = 1.2~mathrm{m/s^{2}}$. We calculate the time for the skier to to reach the speed of $v_text{f} = 7.3~mathrm{m/s}$.

Step 2

2 of 3

We have

$$
begin{align*}
v_text{f} &= v_text{i} + at \
implies t &= frac{v_text{f} – v_text{i}}{a} \
&= frac{7.3~mathrm{m/s} – 0}{1.2~mathrm{m/s^{2}}} \
&= 6.08333~mathrm{s} \
t &= boxed{ 6.1~mathrm{s} }
end{align*}
$$

Result

3 of 3

$$
t = 6.1~mathrm{s}
$$

Exercise 73

Step 1

1 of 3

In this problem, an object initially moving with velocity $v_text{i} = +5.6~mathrm{m/s}$. After $Delta t = 3.1~mathrm{s}$, the velocity is $v_text{f} = -2.7~mathrm{m/s}$. We calculate the object’ average acceleration.

Step 2

2 of 3

The average acceleration is the ratio of the change in velocity and the time interval.

$$
begin{align*}
a_text{av} &= frac{Delta v}{Delta t} \
&= frac{-2.7~mathrm{m/s} – left( +5.6~mathrm{m/s} right)}{3.1~mathrm{s}} \
&= -2.67742~mathrm{m/s^{2}} \
a_text{v} &= boxed{ -2.7~mathrm{m/s^{2}} }
end{align*}
$$

Result

3 of 3

$$
a_text{av} = -2.7~mathrm{m/s^{2}}
$$

Exercise 74

Step 1

1 of 3

In this problem, a car has initial velocity $v_text{i} = +12~mathrm{m/s}$ comes to rest ($v_text{f} = 0$) after $Delta t = 3.5~mathrm{s}$. We calculate its average acceleration.

Step 2

2 of 3

The average acceleration is the ratio of the change in velocity and the time interval.

$$
begin{align*}
a_text{av} &= frac{Delta v}{Delta t} \
&= frac{0 – left( +12~mathrm{m/s} right)}{3.5~mathrm{s}} \
&= -3.42857~mathrm{m/s^{2}} \
a_text{v} &= boxed{ -3.4~mathrm{m/s^{2}} }
end{align*}
$$

Result

3 of 3

$$
a_text{av} = -3.4~mathrm{m/s^{2}}
$$

Exercise 75

Step 1

1 of 6

In this problem, the velocity-time graph of a motorcycle is given. We calculate the average acceleration of the given time segments.

Step 2

2 of 6

The average acceleration is the ratio of the change in velocity and the time interval.

$$
begin{align*}
a_text{av} &= frac{Delta v}{Delta t} tag{1}
end{align*}
$$

Step 3

3 of 6

Part A.
For this part, $v_text{f} = 10~mathrm{m/s}$, $v_text{i} = 0$, and $Delta t = 10~mathrm{s}$.

$$
begin{align*}
a_text{av, A} &= frac{Delta v}{Delta t} \
&= frac{10~mathrm{m/s} – 0}{10~mathrm{s}} \
a_text{av, A} &= boxed{ +1~mathrm{m/s^{2}} }
end{align*}
$$

Step 4

4 of 6

Part B.
For this part, $v_text{f} = 5~mathrm{m/s}$, $v_text{i} = 10~mathrm{m/s}$, and $Delta t = 5~mathrm{s}$.

$$
begin{align*}
a_text{av, B} &= frac{Delta v}{Delta t} \
&= frac{5~mathrm{m/s} – 10~mathrm{m/s}}{5~mathrm{s}} \
a_text{av, B} &= boxed{ -1~mathrm{m/s^{2}} }
end{align*}
$$

Step 5

5 of 6

Part C.
For this part, $v_text{f} = 10~mathrm{m/s}$, $v_text{i} = 10~mathrm{m/s}$, and $Delta t = 10~mathrm{s}$.

$$
begin{align*}
a_text{av, C} &= frac{Delta v}{Delta t} \
&= frac{10~mathrm{m/s} – 10~mathrm{m/s}}{10~mathrm{s}} \
a_text{av, C} &= boxed{ 0 }
end{align*}
$$

Result

6 of 6

begin{enumerate}
item [(a)] $a_text{av, A} = +1~mathrm{m/s^{2}}$
item [(b)] $a_text{av, B} = -1~mathrm{m/s^{2}}$
item [(c)] $a_text{av, C} = 0~mathrm{m/s^{2}}$
end{enumerate}

Exercise 76

Step 1

1 of 6

In this problem, we are given the velocity-time graph of a person on horseback. We find the average acceleration of the horse and rider in the given segments of motion.

Step 2

2 of 6

The average acceleration is the ratio of the change in velocity and the time interval.

$$
begin{align*}
a_text{av} &= frac{Delta v}{Delta t} tag{1}
end{align*}
$$

Step 3

3 of 6

Part A.
For this part, $v_text{f} = 2~mathrm{m/s}$, $v_text{i} = 0$, and $Delta t = 10~mathrm{s}$.

$$
begin{align*}
a_text{av, A} &= frac{Delta v}{Delta t} \
&= frac{2~mathrm{m/s} – 0}{10~mathrm{s}} \
a_text{av, A} &= boxed{ +0.2~mathrm{m/s^{2}} }
end{align*}
$$

Step 4

4 of 6

Part B.
For this part, $v_text{f} = 6~mathrm{m/s}$, $v_text{i} = 2~mathrm{m/s}$, and $Delta t = 5~mathrm{s}$.

$$
begin{align*}
a_text{av, B} &= frac{Delta v}{Delta t} \
&= frac{6~mathrm{m/s} – 2~mathrm{m/s}}{5~mathrm{s}} \
a_text{av, B} &= boxed{ +0.8~mathrm{m/s^{2}} }
end{align*}
$$

Step 5

5 of 6

Part C.
For this part, $v_text{f} = 2~mathrm{m/s}$, $v_text{i} = 6~mathrm{m/s}$, and $Delta t = 10~mathrm{s}$.

$$
begin{align*}
a_text{av, C} &= frac{Delta v}{Delta t} \
&= frac{2~mathrm{m/s} – 6~mathrm{m/s}}{10~mathrm{s}} \
a_text{av, C} &= boxed{ -0.4~mathrm{m/s^{2}} }
end{align*}
$$

Result

6 of 6

begin{enumerate}
item [(a)] $a_text{av, A} = +0.2~mathrm{m/s^{2}}$
item [(b)] $a_text{av, B} = +0.8~mathrm{m/s^{2}}$
item [(c)] $a_text{av, C} = -0.4~mathrm{m/s^{2}}$
end{enumerate}

Exercise 77

Step 1

1 of 4

In this problem, we assume that the brakes in the car create a constant deceleration of $a = -4.2~mathrm{m/s^{2}}$ regardless of the speed. If the driving speed is doubled from $v_text{i, 1} = 16~mathrm{m/s}$ to $v_text{i, 2} = 32~mathrm{m/s}$. We verify the relationship by calculating the exact time.

Step 2

2 of 4

Part A.

The acceleration is constant, and $v_text{f} = 0$. We have

$$
begin{align*}
v_text{f} &= v_text{i} + at \
0 &= v_text{i} + at \
implies t &= left( – frac{1}{a} right)v_text{i} tag{1} \
t &propto v_text{i}
end{align*}
$$

We see that the stopping time is directly proportional to the driving speed. Since the driving speed is doubled, the stopping time also $textbf{doubles}$.

Step 3

3 of 4

Part B.

Calculating the initial stopping time, we use equation (1)

$$
begin{align*}
t_{1} &= left( – frac{1}{a} right)v_text{i, 1} \
&= left( – frac{1}{-4.2~mathrm{m/s^{2}}} right) left( 16~mathrm{m/s} right) \
&= 3.80952~mathrm{s} \
t_{1} &= boxed{ 3.8~mathrm{s} }
end{align*}
$$

Step 4

4 of 4

Part C.

Calculating the final stopping time, we use equation (1)

$$
begin{align*}
t_{2} &= left( – frac{1}{a} right)v_text{i, 2} \
&= left( – frac{1}{-4.2~mathrm{m/s^{2}}} right) left( 32~mathrm{m/s} right) \
&= 7.61905~mathrm{s} \
t_{2} &= boxed{ 7.6~mathrm{s} }
end{align*}
$$

We indeed see that $t_{2} = 2t_{1}$.

Exercise 78

Step 1

1 of 2

In this problem, we differentiate the average acceleration and instantaneous acceleration.

Step 2

2 of 2

The average acceleration of an object is the ratio of the change in velocity $Delta v$ and the time interval $Delta t$. The time interval must be finite and non-zero. The instantaneous acceleration is the acceleration of an object at a point in time, meaning that the time interval $Delta t$ is very close to 0. The instantaneous acceleration is the slope of the tangent line at a certain point in time.

Exercise 79

Step 1

1 of 4

In this problem, Truck 1 accelerates from $v_text{i, 1} = 5~mathrm{m/s}$ to $v_text{f, 1} = 10~mathrm{m/s}$ in $Delta t_{1} = 10~mathrm{s}$. Truck 2, on the other hand, accelerates from $v_text{i, 2} = 10~mathrm{m/s}$ to $v_text{f, 2} = 25~mathrm{m/s}$ in $Delta t_{2} = 15~mathrm{s}$.

Step 2

2 of 4

The acceleration of the trucks are given by

$$
begin{align*}
a &= dfrac{v_text{f} – v_text{i}}{Delta t} tag{1}
end{align*}
$$

Step 3

3 of 4

For truck 2, the acceleration is

$$
begin{align*}
a_{2} &= dfrac{v_text{f, 2} – v_text{i, 2}}{Delta t_{2}} \
&= dfrac{25~mathrm{m/s} – left( 10~mathrm{m/s} right)}{15~mathrm{s}} \
a_{2} &= 1~mathrm{m/s^{2}}
end{align*}
$$

We see that
$$
boxed{ a_{2} > a_{1} }
$$

Result

4 of 4

$$
a_{2} > a_{1}
$$

Exercise 80

Step 1

1 of 5

In this problem, we compare the acceleration of two truck. Truck 1 accelerates from $v_text{i, 1} = 5~mathrm{m/s}$ to $v_text{f, 1} = 10~mathrm{m/s}$ in $Delta x_{1} = 10~mathrm{m}$. Truck 2, on the other hand, accelerates from $v_text{i, 2} = 15~mathrm{m/s}$ to $v_text{f, 2} = 20~mathrm{m/s}$ in $Delta x_{2} = 10~mathrm{m}$.

Step 2

2 of 5

The acceleration can be calculate using

$$
begin{align*}
v_text{f}^{2} &= v_text{i}^{2} + 2a Delta x \
implies a &= frac{v_text{f}^{2} – v_text{i}^{2}}{2 Delta x} tag{1}
end{align*}
$$

Step 3

3 of 5

For truck 1, the acceleration is

$$
begin{align*}
a_{1} &= frac{v_text{f, 1}^{2} – v_text{i, 1}^{2}}{2 Delta x_{1}} \
&= frac{ left( 10~mathrm{m/s} right)^{2} – left( 5~mathrm{m/s} right)^{2}}{2 left( 10~mathrm{m} right)} \
a_{1} &= 3.75~mathrm{m/s^{2}}
end{align*}
$$

Step 4

4 of 5

For truck 2, the acceleration is

$$
begin{align*}
a_{2} &= frac{v_text{f, 2}^{2} – v_text{i, 2}^{2}}{2 Delta x_{2}} \
&= frac{ left( 20~mathrm{m/s} right)^{2} – left( 15~mathrm{m/s} right)^{2}}{2 left( 10~mathrm{m} right)} \
a_{2} &= 8.75~mathrm{m/s^{2}}
end{align*}
$$

We see that
$$
boxed{ a_{1} < a_{2} }
$$

Result

5 of 5

$$
a_{1} < a_{2}
$$

Exercise 81

Step 1

1 of 2

In this problem, an object moves with constant acceleration. We describe the shape of the velocity-time graph.

Step 2

2 of 2

The acceleration is the slope of the velocity-time graph. The acceleration is constant, so the slope is constant. The graph of constant slope is $textbf{linear}$.

Exercise 82

Step 1

1 of 7

In this problem, the cars start from rest ($v_text{i} = 0$). The acceleration distances and final speeds are given in the following table. We rank the accelerations in increasing order.
begin{center}
begin{tabular} {|c|c|c|}
hline
Car & $Delta x$ (in m) & $v_text{f}$ in (m/s)\ hline
1 & 10 & 5 \ hline
2 & 20 & 10 \ hline
3 & 1 & 3 \ hline
4 & 100 & 20 \ hline
end{tabular}
end{center}

Step 2

2 of 7

With $v_text{i} = 0$, we can calculate the acceleration to be

$$
begin{align*}
v_text{f}^{2} &= v_text{i}^{2} + 2a Delta x \
implies a &= frac{v_text{f}^{2} – v_text{i}^{2}}{2 Delta x} \
a &= frac{v_text{f}^{2}}{2 Delta x} tag{1}
end{align*}
$$

Step 3

3 of 7

We use equation (1) to find the acceleration. For Car 1

$$
begin{align*}
a_{1} &= frac{v_text{f, 1}^{2}}{2 Delta x_{1}} \
&= frac{left( 5~mathrm{m/s} right)^{2}}{2 left( 10~mathrm{m} right)} \
a_{1} &= 1.25~mathrm{m/s^{2}}
end{align*}
$$

Step 4

4 of 7

For Car 2

$$
begin{align*}
a_{2} &= frac{v_text{f, 2}^{2}}{2 Delta x_{2}} \
&= frac{left( 10~mathrm{m/s} right)^{2}}{20 left( 10~mathrm{m} right)} \
a_{2} &= 2.5~mathrm{m/s^{2}}
end{align*}
$$

Step 5

5 of 7

For Car 3

$$
begin{align*}
a_{3} &= frac{v_text{f, 3}^{2}}{2 Delta x_{3}} \
&= frac{left( 3~mathrm{m/s} right)^{2}}{2 left( 1~mathrm{m} right)} \
a_{3} &= 4.5~mathrm{m/s^{2}}
end{align*}
$$

Step 6

6 of 7

For Car 4

$$
begin{align*}
a_{4} &= frac{v_text{f, 4}^{2}}{2 Delta x_{4}} \
&= frac{left( 20~mathrm{m/s} right)^{2}}{2 left( 100~mathrm{m} right)} \
a_{4} &= 2~mathrm{m/s^{2}}
end{align*}
$$

We see that
$$
boxed{ a_{1} < a_{4} < a_{2} < a_{3} }
$$

Result

7 of 7

$$
a_{1} < a_{4} < a_{2} < a_{3}
$$

Exercise 83

Step 1

1 of 3

In this problem, two bows shoot identical arrows with the same initial speed. The string of bow 1 must be pulled back farther than the string in bow 2. We compare the accelerations and explain the relationship.

Step 2

2 of 3

Part A.

The arrows start from rest, and with the final speed being equal, we have

$$
begin{align*}
v_text{f}^{2} &= v_text{i}^{2} + 2a Delta x \
v_text{f}^{2} &= 0 + 2a Delta x \
a &= left( frac{v_text{f}^{2}}{2} right) left( frac{1}{Delta x} right) \
a &propto frac{1}{Delta x}
end{align*}
$$

The acceleration is inversely proportional to the acceleration distance, since the final speed is equal (constant in the situation). The acceleration distance of arrow 1 is greater, so its acceleration must be $textbf{less than}$ the acceleration of bow 2.

Step 3

3 of 3

Part B.

From the previous part, we see that this happens because $textbf{(C)}$ the arrow from bow 1 accelerates over a greater distance.

Exercise 84

Step 1

1 of 3

In this problem, an airplane lands with speed $v_text{i} = 81.9~mathrm{m/s}$ due south. It comes to rest in $Delta x = 949~mathrm{m}$. Assuming that the acceleration i constant, we calculate the magnitude and direction of this acceleration.

Step 2

2 of 3

Let southwards be the positive direction. The final velocity is $v_text{i} = 0$.
With $v_text{i} = 0$, we can calculate the acceleration to be

$$
begin{align*}
v_text{f}^{2} &= v_text{i}^{2} + 2a Delta x \
implies a &= frac{v_text{f}^{2} – v_text{i}^{2}}{2 Delta x} \
&= frac{ left( 0 right)^{2} – left( 81.9~mathrm{m/s} right)^{2} }{2 left( 949~mathrm{m} right)} \
&= -3.53404~mathrm{m/s^{2}} \
a &= boxed{ -3.53~mathrm{m/s^{2}} }
end{align*}
$$

Step 3

3 of 3

The acceleration has magnitude
$$
boxed{ a = 3.53~mathrm{m/s^{2}} }
$$
and because the sign is negative, it must be $textbf{due north}$.

Exercise 85

Step 1

1 of 3

In this problem, a park ride accelerates from $v_text{i} = 0$ to $v_text{f} = 20.1~mathrm{m/s}$ in $Delta t = 2.2~mathrm{s}$. We calculate the average acceleration.

Step 2

2 of 3

The average acceleration is given by

$$
begin{align*}
a_text{av} &= frac{v_text{f} – v_text{i}}{Delta t} \
&= frac{20.1~mathrm{m/s} – 0}{2.2~mathrm{s}} \
&= 9.13636~mathrm{m/s^{2}} \
a_text{av} &= boxed{ 9.1~mathrm{m/s^{2}} }
end{align*}
$$

Result

3 of 3

$$
a_text{av} = 9.1~mathrm{m/s^{2}}
$$

Exercise 86

Step 1

1 of 3

In this problem, a spaceship is blasted out of a cannon with speed about $v_text{f} = 11000~mathrm{m/s}$ and accelerates over a distance of $Delta x = 213~mathrm{m}$. We calculate the acceleration.

Step 2

2 of 3

The spaceship starts from rest $v_text{i} = 0$. The acceleration can be calculated from

$$
begin{align*}
v_text{f}^{2} &= v_text{i}^{2} + 2a Delta x \
implies a &= frac{v_text{f}^{2} – v_text{i}^{2}}{2 Delta x} \
&= frac{ left( 11000~mathrm{m/s} right)^{2} – left( 0 right) }{2 left( 213~mathrm{m} right)} \
&= 2.84037 times 10^{5}~mathrm{m/s^{2}} \
a &= boxed{ 2.8 times 10^{5}~mathrm{m/s^{2}} }
end{align*}
$$

Result

3 of 3

$$
a = 2.8 times 10^{5}~mathrm{m/s^{2}}
$$

Exercise 87

Step 1

1 of 3

In this problem, the tongue of a chameleon can extend to $x = 0.16~mathrm{m}$ in $t = 0.10~mathrm{s}$. We calculate the acceleration, assuming it to be constant.

Step 2

2 of 3

The equation we can use

$$
begin{align*}
x &= x_text{i} + v_text{i}t + frac{1}{2}at^{2} \
x &= 0 + 0 + frac{1}{2}at^{2} \
implies a &= frac{2x}{t^{2}} \
&= frac{2 left( 0.16~mathrm{m} right)}{left( 0.10~mathrm{s} right)^{2}} \
a &= boxed{ 32~mathrm{m/s^{2}} }
end{align*}
$$

Result

3 of 3

$$
a = 32~mathrm{m/s^{2}}
$$

Exercise 88

Step 1

1 of 3

In this problem, an air bag expand by $x = 0.20~mathrm{m}$ in $t = 10 times 10^{-3}~mathrm{s}$. We calculate its acceleration and express it in terms of $g = 9.81~mathrm{m/s^{2}}$.

Step 2

2 of 3

We use the following equation

$$
begin{align*}
x &= x_text{i} + v_text{i}t + frac{1}{2}at^{2} \
x &= 0 + 0 + frac{1}{2}at^{2} \
implies a &= frac{2x}{t^{2}} \
&= frac{2 left( 0.20~mathrm{m} right)}{left( 10 times 10^{-3}~mathrm{s} right)^{2}} times frac{g}{9.81~mathrm{m/s^{2}}} \
&= 4.07747 times 10^{2}~g\
a &= boxed{ 4.1 times 10^{2}~g }
end{align*}
$$

Result

3 of 3

$$
a = 4.1 times 10^{2}~g
$$

Exercise 89

Step 1

1 of 6

In this problem, we are given the velocity-time graph of a person on horse back. We calculate the displacement of the horse and rider on the given segments.

Step 2

2 of 6

In each segment, the acceleration is constant, so we can use the following equation

$$
begin{align*}
Delta x &= left( frac{v_text{f} + v_text{i}}{2} right)Delta t
end{align*}
$$

Step 3

3 of 6

Part A.

For this segment, $v_text{i, A} = 0~mathrm{m/s}$m, $v_text{f, A} = 2~mathrm{m/s}$, and $Delta t_{A} = 10~mathrm{s}$.

$$
begin{align*}
Delta x_{A} &= left( frac{v_text{f, A} + v_text{i, A}}{2} right)Delta t_{A} \
&= left( frac{2~mathrm{m/s} + 0}{2} right) left( 10~mathrm{s} right) \
Delta x_{A} &= boxed{10~mathrm{m}}
end{align*}
$$

Step 4

4 of 6

Part B.

For this segment, $v_text{i, B} = 2~mathrm{m/s}$m, $v_text{f, B} = 6~mathrm{m/s}$, and $Delta t_{B} = 5~mathrm{s}$.

$$
begin{align*}
Delta x_{B} &= left( frac{v_text{f, B} + v_text{i, B}}{2} right)Delta t_{B} \
&= left( frac{6~mathrm{m/s} + 2~mathrm{m/s}}{2} right) left( 5~mathrm{s} right) \
Delta x_{B} &= boxed{20~mathrm{m}}
end{align*}
$$

Step 5

5 of 6

Part C.

For this segment, $v_text{i, C} = 6~mathrm{m/s}$m, $v_text{f, C} = 2~mathrm{m/s}$, and $Delta t_{C} = 10~mathrm{s}$.

$$
begin{align*}
Delta x_{C} &= left( frac{v_text{f, C} + v_text{i, C}}{2} right)Delta t_{C} \
&= left( frac{2~mathrm{m/s} + 6~mathrm{m/s}}{2} right) left( 10~mathrm{s} right) \
Delta x_{C} &= boxed{40~mathrm{m}}
end{align*}
$$

Result

6 of 6

begin{enumerate}
item [(a)] $Delta x_{A} = 10~mathrm{m}$
item [(b)] $Delta x_{B} = 20~mathrm{m}$
item [(c)] $Delta x_{C} = 40~mathrm{m}$
end{enumerate}

Exercise 90

Step 1

1 of 4

In this problem, it is given that the brakes of a car create a constant acceleration of $a = -3.7~mathrm{m/s^{2}}$ regardless of the driving speed. In this problem, the driving speed is doubled from $v_text{i, 1} = 11~mathrm{m/s}$ to $v_text{i, 2} = 22~mathrm{m/s}$. We see what happens to the stopping distance, if increases by a factor of 2 or 4.

Step 2

2 of 4

Part A.

First, we see how the stopping distance is related to the driving speed. Since the car stops, $v_text{f} = 0$. We have

$$
begin{align*}
v_text{f}^{2} &= v_text{i}^{2} + 2a Delta x \
0 &= v_text{i}^{2} + 2a Delta x \
implies Delta x &= left( – frac{1}{2a} right)v_text{i}^{2} tag{1} \
Delta x &propto v_text{i}^{2}
end{align*}
$$

The stopping distance is proportional to the square of the driving speed. Since the driving speed is doubled, the distance required to stop must be increased by a factor of
$$
boxed{4}
$$

Step 3

3 of 4

Part B.

To find the actual distances, we use equation (1). For $v_text{i, 1} = 11~mathrm{m/s}$, we have

$$
begin{align*}
Delta x_{1} &= left( – frac{1}{2a} right)v_text{i, 1}^{2}\
&= left( – frac{1}{2left( -3.7~mathrm{m/s^{2}} right)} right) left( 11~mathrm{m/s} right)^{2} \
&= 16.35135~mathrm{m} \
Delta x_{1} &= boxed{ 16.4~mathrm{m} }
end{align*}
$$

Step 4

4 of 4

Part C.

For $v_text{i, 2} = 22~mathrm{m/s}$, we have

$$
begin{align*}
Delta x_{2} &= left( – frac{1}{2a} right)v_text{i, 2}^{2}\
&= left( – frac{1}{2left( -3.7~mathrm{m/s^{2}} right)} right) left( 22~mathrm{m/s} right)^{2} \
&= 65.40541~mathrm{m} \
Delta x_{2} &= boxed{ 65.4~mathrm{m} }
end{align*}
$$

We see that indeed
$$
boxed{ Delta x_{2} = 4Delta x_{1} }
$$

Exercise 91

Step 1

1 of 4

In this problem, a driver is traveling with speed $v_text{i} = 12.0~mathrm{m/s}$ when a ball roll out. The driver hits the brakes, and the vehicle experiences an acceleration of $a = -3.5~mathrm{m/s^{2}}$. We find the distance before the vehicle stop, and the speed when the vehicle has traveled half of this total distance.

Step 2

2 of 4

Part A.

For this part $v_text{f} = 0$ since the vehicle has stopped. We have

$$
begin{align*}
v_text{f}^{2} &= v_text{i}^{2} + 2a Delta x tag{1} \
0 &= v_text{i}^{2} + 2a Delta x \
implies Delta x &= -frac{v_text{i}^{2}}{2a} tag{2} \
&= – frac{left( 12~mathrm{m/s} right)^{2}}{2 left( -3.5~mathrm{m/s^{2}} right)} \
&= 20.57143~mathrm{m} \
Delta x &= boxed{ 21~mathrm{m} }
end{align*}
$$

Step 3

3 of 4

Part B.

Notice that the decrease in velocity squared is proportional to the distance traveled, so the decrease velocity must follow a square root relation with respect to the distance. The square root function is less than the direct proportion, so the $textit{decrease}$ in speed is less than in the decrease direct proportion (if the distance is halved, the speed is also halved). Since the decrease is less, the speed is $textbf{greater than}$ $6.0~mathrm{m/s}$.

Step 4

4 of 4

Part C.

We use again equations (1) and (2). The displacement this time is $Delta x’ = frac{1}{2}Delta x = frac{1}{2} left( -frac{v_text{i}^{2}}{2a} right)$ from equation (2). Using equation (1),we have

$$
begin{align*}
v_text{f}^{2} &= v_text{i}^{2} + 2a Delta x’ \
&= v_text{i}^{2} + 2a left[ frac{1}{2} left( -frac{v_text{i}^{2}}{2a} right) right] \
&= v_text{i}^{2} – frac{v_text{i}^{2}}{2} \
v_text{f}^{2} &= frac{v_text{i}^{2}}{2} \
v_text{f} &= sqrt{frac{v_text{i}^{2}}{2}} \
&=frac{v_text{i}}{sqrt{2}} \
&=frac{12.0~mathrm{m/s}}{sqrt{2}}\
&= 8.48528~mathrm{m/s} \
v_text{f} &= boxed{ 8.5~mathrm{m/s} }
end{align*}
$$

The speed is indeed greater than $6.0~mathrm{m/s}$

Exercise 92

Step 1

1 of 4

In this problem, David Purley decelerated from $v_text{i} = 173~mathrm{km/h}$ to $v_text{f} = 0$ in a distance of $Delta x = 0.66~mathrm{m}$. We calculate the magnitude of his acceleration in terms of $g = 9.81~mathrm{m/s^{2}}$.

Step 2

2 of 4

First, we convert the given speed into $mathrm{m/s}$. We have

$$
begin{align}
v_text{i} &= 173~mathrm{km/h} times frac{1000~mathrm{m}}{1~mathrm{km}} times frac{1~mathrm{h}}{3600~mathrm{s}} \
v_text{i} &= frac{865}{18}~mathrm{m/s}
end{align}
$$

Step 3

3 of 4

We now calculate the magnitude of the acceleration

$$
begin{align*}
v_text{f}^{2} &= v_text{i}^{2} + 2a Delta x’ \
implies a &= leftvert frac{v_text{f}^{2} – v_text{i}^{2}}{2Delta x} rightvert frac{g}{9.81~mathrm{m/s^{2}}} \
&= leftvert frac{ left( 0 right)^{2} – left( frac{865}{18}~mathrm{m/s} right)^{2}}{2left( 0.66~mathrm{m} right)} rightvert frac{g}{9.81~mathrm{m/s^{2}}} \
&= 178.33815g \
a &= boxed{180g}
end{align*}
$$

Result

4 of 4

$$
a = 180g
$$

Exercise 93

Step 1

1 of 2

In this problem, an object moves with a constant, negative acceleration. We describe the shape of its position-time graph.

Step 2

2 of 2

An object with constant acceleration has a parabolic position-time graph. The parabola opens downward if the sign of the acceleration is negative. For this given, the position-time graph is a $textbf{downward parabola}$.

Exercise 94

Step 1

1 of 2

In this problem, we are given the position-time graph of an object with constant acceleration.

Step 2

2 of 2

The intercept represents the $textbf{initial position}$ of the object. The initial slope of a position-time graph corresponds to the $textbf{initial velocity.}$

Exercise 95

Step 1

1 of 2

In this problem, we are given that the position-time graph of an object is a straight line.

Step 2

2 of 2

The slope is constant, so the velocity must also be constant. Since the velocity is constant, the acceleration must be $textbf{zero}$.

Exercise 96

Step 1

1 of 3

In this problem, the position-time graph of an object with constant acceleration starts off with a positive slope. Later on, the slope becomes negative. We find the signs of the initial velocity and acceleration.

Step 2

2 of 3

The initial slope is the initial velocity. Since the initial slope is positive, the initial velocity must be $textbf{positive}$.

Step 3

3 of 3

The velocity changes from positive to negative. The net change of a negative minus positive is a negative, so the acceleration must be $textbf{negative}$.

Exercise 97

Step 1

1 of 3

In this problem, the position-time graph of an object with constant acceleration starts off with zero slope. Later on, the slop becomes positive. We find the signs of the initial velocity and acceleration.

Step 2

2 of 3

The initial slope is the initial velocity. Since the initial slope is zero, the initial velocity must be $textbf{zero}$.

Step 3

3 of 3

The net change of velocity, which is positive minus zero, is positive, so the acceleration must be $textbf{positive}$.

Exercise 98

Step 1

1 of 3

In this problem, a car has initial position $x_text{i} = 5.5~mathrm{m}$, initial velocity of $v_text{i} = +2.1~mathrm{m/s}$, and constant acceleration of $a = +0.75~mathrm{m/s^{2}}$. We find the position at time $t = 2.5~mathrm{s}$.

Step 2

2 of 3

The position-time equation is

$$
begin{align*}
x_text{f} &= x_text{i} + v_text{i}t + frac{1}{2}at^{2} \
&= 5.5~mathrm{m} + left( +2.1~mathrm{m/s} right) left( 2.5~mathrm{s} right) + frac{1}{2} left( +0.75~mathrm{m/s^{2}} right) left( 2.5~mathrm{s} right)^{2} \
&= 13.09375~mathrm{m} \
x_text{f} &= boxed{ 13~mathrm{m} }
end{align*}
$$

Result

3 of 3

$$
x_text{f} = 13~mathrm{m}
$$

Exercise 99

Step 1

1 of 3

In this problem, a car has initial position $x_text{i} = 3.2~mathrm{m}$, initial velocity of $v_text{i} = -8.4~mathrm{m/s}$, and constant acceleration of $a = +1.1~mathrm{m/s^{2}}$. We find the position at time $t = 1.5~mathrm{s}$.

Step 2

2 of 3

The position-time equation is

$$
begin{align*}
x_text{f} &= x_text{i} + v_text{i}t + frac{1}{2}at^{2} \
&= 3.2~mathrm{m} + left( -8.4~mathrm{m/s} right) left( 1.5~mathrm{s} right) + frac{1}{2} left( +1.1~mathrm{m/s^{2}} right) left( 1.5~mathrm{s} right)^{2} \
&= -8.16250~mathrm{m} \
x_text{f} &= boxed{ -8.2~mathrm{m} }
end{align*}
$$

Result

3 of 3

$$
x_text{f} = -8.2~mathrm{m}
$$

Exercise 100

Step 1

1 of 5

In this problem, we are given the position-time equation of a train.

$$
begin{align*}
x_text{f} &= 2.1~mathrm{m} + left( 8.3~mathrm{m/s} right)t + left( 2.6~mathrm{m/s^{2}} right)t^{2}
end{align*}
$$

We find its initial velocity and acceleration.

Step 2

2 of 5

The position-time equation is given by

$$
begin{align*}
x_text{f} &= x_text{i} + v_text{i}t + frac{1}{2}at^{2}
end{align*}
$$

We compare this general equation to the given equation.

Step 3

3 of 5

Part A.

For this part, we find te initial velocity of the train. Comparing the general equation to the given equation, we see that

$$
begin{align*}
v_text{i} &= boxed{ 8.3~mathrm{m/s} }
end{align*}
$$

Step 4

4 of 5

Part B.

For this part, we find the acceleration. We have

$$
begin{align*}
frac{1}{2}a &= 2.6~mathrm{m/s^{2}} \
implies a &= boxed{ 5.2~mathrm{m/s^{2}} }
end{align*}
$$

Result

5 of 5

begin{enumerate}
item [(a)] $v_text{i} = 8.3~mathrm{m/s}$
item [(b)] $a = 5.2~mathrm{m/s^{2}}$
end{enumerate}

Exercise 101

Step 1

1 of 3

In this problem, we find the position of the train in Problem 101 at time $t = 4.1~mathrm{s}$. The position-time equation is

$$
begin{align*}
x_text{f} &= 2.1~mathrm{m} + left( 8.3~mathrm{m/s} right)t + left( 2.6~mathrm{m/s^{2}} right)t^{2}
end{align*}
$$

Step 2

2 of 3

We simply need to substitute the given time to the position-time equation.

$$
begin{align*}
x_text{f} &= 2.1~mathrm{m} + left( 8.3~mathrm{m/s} right) left( 4.1~mathrm{s} right) + left( 2.6~mathrm{m/s^{2}} right) left( 4.1~mathrm{s} right)^{2} \
&= 79.83600~mathrm{m} \
x_text{f} &= boxed{ 80.~mathrm{m} }
end{align*}
$$

Result

3 of 3

$$
x_text{f} = 80.~mathrm{m}
$$

Exercise 102

Step 1

1 of 7

In this problem, the position-time equation for a cheetah chasing an antelope is

$$
begin{align*}
x_text{f} &= 1.6~mathrm{m} + left( 1.7~mathrm{m/s^{2}} right)t^{2} tag{1}
end{align*}
$$

We find its initial position, initial velocity, acceleration, and position at time $t = 4.4~mathrm{s}$.

Step 2

2 of 7

The position-time equation is given by

$$
begin{align*}
x_text{f} &= x_text{i} + v_text{i}t + frac{1}{2}at^{2}
end{align*}
$$

We compare this general equation to the given equation.

Step 3

3 of 7

Part A.

We first find the initial position. From the position-time equation, we have

$$
begin{align*}
x_text{i} &= boxed{ 1.6~mathrm{m} }
end{align*}
$$

Step 4

4 of 7

Part B.

For this part, we find the initial velocity. We have

$$
begin{align*}
v_text{i} &= boxed{0}
end{align*}
$$

Step 5

5 of 7

Part C.

For this part, we find the acceleration. We have

$$
begin{align*}
frac{1}{2}a &= 1.7~mathrm{m/s^{2}} \
implies a &= boxed{ 3.4~mathrm{m/s^{2}} }
end{align*}
$$

Step 6

6 of 7

Part D.

For this part, we simply substitute $t = 4.4~mathrm{s}$ to equation (1). We have

$$
begin{align*}
x_text{f} &= 1.6~mathrm{m} + left( 1.7~mathrm{m/s^{2}} right) left( 4.4~mathrm{s} right)^{2} \
&= 34.51200~mathrm{m} \
x_text{f} &= boxed{ 35~mathrm{m}}
end{align*}
$$

Result

7 of 7

begin{enumerate}
item [(a)] $x_text{i} = 1.6~mathrm{m}$
item [(b)] $v_text{i} = 0$
item [(c)] $a = 3.4~mathrm{m/s^{2}}$
item [(d)] $x_text{f} = 35~mathrm{m}$
end{enumerate}

Exercise 103

Step 1

1 of 5

In this problem, we are given that the balls start from rest at $x_text{i} = 0$ with $v_text{i} = 0$. It has a constant acceleration of $a = 2.4~mathrm{m/s^{2}}$. We find its position-time equation, and position at times $t = 1.0~mathrm{s}$ and $t = 2.0~mathrm{s}$.

Step 2

2 of 5

Part A.

The position-time equation is

$$
begin{align*}
x_text{f} &= x_text{i} + v_text{i} + frac{1}{2}at^{2} \
&= 0 + 0 + frac{1}{2} left( 2.4~mathrm{m/s^{2}} right)t^{2} \
x_text{f} &= boxed{ left( 1.2~mathrm{m/s^{2}} right)t^{2} }
end{align*}
$$

Step 3

3 of 5

Part B.

For this part, we ue the position, time equation. We substitute $t = 1.0~mathrm{s}$.

$$
begin{align*}
x_text{f} &= left( 1.2~mathrm{m/s^{2}} right) left( 1.0~mathrm{s} right)^{2} \
x_text{f} &= boxed{ 1.2~mathrm{m} }
end{align*}
$$

Step 4

4 of 5

Part C.

For this part, we substitute $t = 2.0~mathrm{s}$ into the position-time equation.

$$
begin{align*}
x_text{f} &= left( 1.2~mathrm{m/s^{2}} right) left( 2.0~mathrm{s} right)^{2} \
x_text{f} &= boxed{ 4.8~mathrm{m} }
end{align*}
$$

Result

5 of 5

begin{enumerate}
item [(a)] $x_text{f} = left( 1.2~mathrm{m/s^{2}} right)t^{2}$
item [(b)] $x_text{f} = 1.2~mathrm{m}$
item [(c)] $x_text{f} = 4.8~mathrm{m}$
end{enumerate}

Exercise 104

Step 1

1 of 6

In this problem, we are given that fishing boat leaves a marina with a constant speed of $v_text{i, fb} = 3.4~mathrm{m/s}$. A speedboat leaves the marina after a time $t’ = 12~mathrm{s}$ with initial speed $v_text{i, sp} = 2.8~mathrm{m/s}$ and constant acceleration of $a = 1.7~mathrm{m/s^{2}}$. Let $t = 0$ be the time the speedboat leaves the marina. We write the position-time equation for the two boards, and find the time when the speedboat catches up with the fishing boat.

Step 2

2 of 6

Part A.

The fishing boat has a time lead of $t’ = 12~mathrm{s}$. The position time graph is

$$
begin{align*}
x_text{f, fb} &= x_text{i, fb} + v_text{i, fb}left( t + t’ right) + frac{1}{2}a_text{fb} left( t + t’ right)^{2} \
&= 0 + left( 3.4~mathrm{m/s} right) left( t + 12~mathrm{s} right) + 0 \
x_text{f, fb} &= boxed{ left( 3.4~mathrm{m/s} right) left( t + 12~mathrm{s} right) }
end{align*}
$$

Step 3

3 of 6

For the speedboat, we have

$$
begin{align*}
x_text{f, sb} &= x_text{i, sb} + v_text{i, sb}t + frac{1}{2}a_text{sb} t^{2} \
&= 0 + left( 2.8~mathrm{m/s} right)t + frac{1.7~mathrm{m/s^{2}}}{2}t^{2} \
x_text{f, sb} &= boxed{ left( 2.8~mathrm{m/s} right) t + left( 0.85~mathrm{m/s^{2}} right)t^{2} }
end{align*}
$$

Step 4

4 of 6

Part B.

The speedboat catches up with the fishing boat when $x_text{f, fb} = x_text{f, b}$. We have

$$
begin{align*}
x_text{f, fb} &= x_text{f, sb} \
left( 3.4~mathrm{m/s} right) left( t + 12~mathrm{s} right) &= left( 2.8~mathrm{m/s} right) t + left( 0.85~mathrm{m/s^{2}} right)t^{2} \
implies 0 &= left( 0.85~mathrm{m/s^{2}} right)t^{2} + left( 2.8~mathrm{m/s} – 3.4~mathrm{m/s} right) t – 40.8~mathrm{m} \
0 &= left( 0.85~mathrm{m/s^{2}} right)t^{2} – left( 0.60~mathrm{m/s} right) t – 40.8~mathrm{m}tag{1}
end{align*}
$$

Step 5

5 of 6

Equation (1) is a quadratic with roots

$$
begin{align*}
t &= – frac{-0.60~mathrm{m/s}}{2 left( 0.85~mathrm{m/s^{2}} right)} pm frac{ sqrt{left( -0.60~mathrm{m/s} right)^{2} – 4left( 0.85~mathrm{m/s^{2}} right)left( -40.8~mathrm{m} right)}}{2 left( 0.85~mathrm{m/s^{2}} right)} \
t &= -6.58425~mathrm{s},~7.29013~mathrm{s}
end{align*}
$$

We take the positive root for time, since the speedboat only starts running at time $t = 0$. The time they meet is at
$$
boxed{ t = 7.3~mathrm{s} }
$$

Result

6 of 6

begin{enumerate}
item [(a)] $x_text{f, fb} = left( 3.4~mathrm{m/s} right) left( t + 12~mathrm{s} right)$, $x_text{f, sb} = left( 2.8~mathrm{m/s} right) t + left( 0.85~mathrm{m/s^{2}} right)t^{2}$
item [(b)] $t = 7.3~mathrm{s}$
end{enumerate}

Exercise 105

Step 1

1 of 2

In this problem, we explain what it means to say that an object is in free-fall.

Step 2

2 of 2

An object in free-fall is an object that travels such that its only acceleration is the acceleration due to gravity. The other forces on the object are negligible.

Exercise 106

Step 1

1 of 2

$$
text{color{#4257b2}boxed{bf Yes}, color{default} a ball that is thrown upward, under conditions where air friction is negligible, is still described as being in free fall because its motion is influenced only by gravity.}
$$

Result

2 of 2

Yes

Exercise 107

Step 1

1 of 2

In this problem, an object dropped at time $t = 0$ is allowed to fall freely toward the ground. In this problem, we compare the distance traveled in the time interval $t = 0$ to $t = 1~mathrm{s}$ to the distance traveled in the time interval $t = 1~mathrm{s}$ to $t = 2~mathrm{s}$.

Step 2

2 of 2

The distance traveled is proportional to the $textbf{square}$ of the time of travel. Hence, the distance traveled in a time interval is proportional to the difference of the squares of the time points. The difference in square for the first case is $1^{2} – 0^{2} = 1$ and for the second case, it is $2^{2} – 1^{2} = 3$. We see that the distance traveled in the time interval $t = 0$ to $t = 1~mathrm{s}$ is $textbf{less than}$ the distance traveled in the time interval $t = 1~mathrm{s}$ to $t = 2~mathrm{s}$.

Exercise 108

Step 1

1 of 3

In this problem, a batter hits a pop fly straight up. We compare the acceleration on the way up and on the way down, and at the top of the flight and right before it lands.

Step 2

2 of 3

Part A.

The object is in free-fall. Its acceleration is constant, and is always pointing toward the ground. Hence, for this part, the acceleration is $textbf{not different}$ in the given two segments of its trajectory.

Step 3

3 of 3

Part B.

Exercise 109

Step 1

1 of 2

Since the second ball is thrown downward with a certain initial speed, it will spend less time in the air than the first one.
Both balls are accelerated at the same rate ($g=9.81:m/s^2$), so the ball $1$, which spends more time in the air, will have it’s speed be increased more than the ball $2$.

Result

2 of 2

Greater than.

Exercise 110

Step 1

1 of 3

In this problem, we calculate the distance in which a free falling apple travels in $t = 5.0~mathrm{s}$ after being released from rest. We use $g = 9.81~mathrm{m/s^{2}}$.

Step 2

2 of 3

Let downwards be the positive direction, since we are calculating how far an object has fallen. The initial velocity must be $v_text{i} = 0$ since the apple starts from rest.

$$
begin{align*}
x_text{f} &= x_text{i} + v_text{i}t + frac{1}{2}at^{2} \
&= 0 + 0 + frac{9.81~mathrm{m/s^{2}}}{2}left( 5.0~mathrm{s} right)^{2} \
&= 122.62500~mathrm{m} \
x_text{f} &= boxed{ 120~mathrm{m} }
end{align*}
$$

Result

3 of 3

$$
x_text{f} = 120~mathrm{m}
$$

Exercise 111

Step 1

1 of 3

In this problem, we calculate the speed of a free-falling baseball after a time $t = 6.0~mathrm{s}$ after being dropped from rest. We use $g = 9.81~mathrm{m/s^{2}}$.

Step 2

2 of 3

We let the downward direction be positive. The initial velocity $v_text{i} = 0$. The velocity-time equation is

$$
begin{align*}
v_text{f} &= v_text{i} + at \
&= 0 + left( 9.81~mathrm{m/s^{2}} right) left( 6.0~mathrm{s} right) \
&= 58.80000~mathrm{s} \
v_text{f} &= boxed{ 59~mathrm{m/s} }
end{align*}
$$

Result

3 of 3

$$
v_text{f} = 59~mathrm{m/s}
$$

Exercise 112

Step 1

1 of 2

In this problem, a friend claim that a free falling car goes from $v_text{i} = 0$ to $v_text{f} = 26.8~mathrm{m/s}$ in about $t = 3.00~mathrm{s}$. We verify the statement.

Step 2

2 of 2

We calculate time in which a free-falling object reaches $v_text{f} = 26.8~mathrm{m/s}$.

$$
begin{align*}
v_text{f} &= v_text{i} + at \
implies t &= frac{v_text{f} – v_text{i}}{g} \
&= frac{26.8~mathrm{m/s} – 0}{9.81~mathrm{m/s^{2}}} \
t &= 2.73419~mathrm{s}
end{align*}
$$

The calculated time is approximately $3.0~mathrm{s}$, so the statement is $textbf{true}$.

Exercise 113

Step 1

1 of 4

In this problem, a grapefruit falls from a tree and hits the ground after a time $t = 0.75~mathrm{s}$. We calculate the distance traveled and its speed as it hits the ground. We use $g = 9.81~mathrm{m/s^{2}}$.

Step 2

2 of 4

Part A.

Let downwards be the positive direction. The position-time equation of the grapefruit is

$$
begin{align*}
x_text{f} &= x_text{i} + v_text{i}t + frac{1}{2}at^{2} \
&= 0 + 0 + frac{9.81~mathrm{m/s^{2}}}{2} left( 0.75~mathrm{s} right)^{2} \
&= 2.75906~mathrm{m} \
x_text{f} &= boxed{ 2.8~mathrm{m} }
end{align*}
$$

Step 3

3 of 4

Part B.

The velocity-time equation is

$$
begin{align*}
v_text{f} &= v_text{i} + at \
&= 0 + left( 9.81~mathrm{m/s^{2}} right)left( 0.75~mathrm{s} right) \
&= 7.35750~mathrm{m/s} \
v_text{f} &= boxed{ 7.4~mathrm{m/s} }
end{align*}
$$

Result

4 of 4

begin{enumerate}
item [(a)] $x_text{f} = 2.8~mathrm{m}$
item [(b)] $v_text{f} = 7.4~mathrm{m/s}$
end{enumerate}

Exercise 114

Step 1

1 of 3

In this problem, an astronaut drops a rock on the surface of an asteroid. It is released from rest from height $h = 0.95~mathrm{m}$ and hits the ground after time $t = 1.39~mathrm{s}$. We calculate the acceleration due to gravity.

Step 2

2 of 3

The equation we can ue is

$$
begin{align*}
h &= frac{1}{2}at^{2} \
implies a &= frac{2h}{t^{2}} \
&= frac{2 left( 0.95~mathrm{m} right) }{left( 1.39~mathrm{s} right)^{2}} \
&= 0.98339~mathrm{m/s^{2}} \
a &= boxed{ 0.98~mathrm{m/s^{2}} }
end{align*}
$$

Result

3 of 3

$$
a = 0.98~mathrm{m/s^{2}}
$$

Exercise 115

Step 1

1 of 3

In this problem, a person catches a ruler after it has traveled $d = 5.2 times 10^{-2}~mathrm{m}$. We calculate the reaction time. We use $g = 9.81~mathrm{m/s^{2}}$.

Step 2

2 of 3

We have

$$
begin{align*}
d &= frac{1}{2}at^{2} \
implies t &= sqrt{ frac{2d}{g} } \
&= sqrt{ frac{2 left( 5.2 times 10^{-2}~mathrm{m} right)}{9.81~mathrm{m/s^{2}}} } \
&= 0.10296~mathrm{s} \
t &= boxed{ 0.10~mathrm{s} }
end{align*}
$$

Result

3 of 3

$$
t = 0.10~mathrm{s}
$$

Exercise 116

Step 1

1 of 4

In this problem, water rises to a height of $h = 171~mathrm{m}$. We calculate its initial speed and the time it takes for the water to reach the highest point. We use $g = 9.81~mathrm{m/s^{2}}$.

Step 2

2 of 4

Part A.

The final speed must be $v_text{f} = 0$. We have

$$
begin{align*}
v_text{f}^{2} &= v_text{i}^{2} + 2a Delta x \
v_text{f}^{2} &= v_text{i}^{2} – 2gh \
implies v_text{i}^{2} &= 2gh \
v_text{i} &= sqrt{2gh} \
&= sqrt{2 left( 9.81~mathrm{m/s^{2}} right) left( 171~mathrm{m} right)} \
&= 57.92253~mathrm{m/s} \
v_text{i} &= boxed{ 57.9~mathrm{m/s} }
end{align*}
$$

Step 3

3 of 4

Part B.

To find the time it takes to reach the peak, we use

$$
begin{align*}
h &= frac{1}{2}at^{2} \
implies t &= sqrt{ frac{2h}{g} } \
&= sqrt{ frac{2 left( 171~mathrm{m} right)}{9.81~mathrm{m/s^{2}}} } \
&= 5.90444~mathrm{s} \
t &= boxed{ 5.90~mathrm{s} }
end{align*}
$$

Result

4 of 4

begin{enumerate}
item [(a)] $v_text{i} = 57.9~mathrm{m/s}$
item [(b)] $t = 5.90~mathrm{s}$
end{enumerate}

Exercise 117

Step 1

1 of 6

In this problem, a person and a friend step off a bridge. The total travel time to hit the water is $t = 1.6~mathrm{s}$. The friend goes first, and when the friend is at $x_text{1} = 2.0~mathrm{m}$, the observer steps off the bridge. We calculate their separation when the friend hits the water. We use $g = 9.81~mathrm{m/s^{2}}$.

Step 2

2 of 6

Part A.

Notice that the distance traveled is proportional to the square of the time. This means that the separation of the two people are getting longer since the difference the squares of the time is increasing. Hence, their separation when the friend hits the water is $textbf{more than}$ 2.0 m.

Step 3

3 of 6

Part B.

We let the time $t = 0$ be the time when the friend steps off the bridge. The time $t_{1}$ taken to reach the depth $x_text{1}$ is

$$
begin{align*}
x_{1} &= frac{1}{2}gt_{1}^{2} \
implies t_{1} &= sqrt{frac{2x_{1}}{g}} tag{1}
end{align*}
$$

Step 4

4 of 6

The position-time equation for the friend is given below. We find the depth of the fall when we substitute $t = 1.6~mathrm{s}$

$$
begin{align*}
x_text{f, fr} &= frac{1}{2}gt^{2} \
&= frac{1}{2} left( 9.81~mathrm{m/s^{2}} right) left( 1.6~mathrm{s} right)^{2} \
x_text{f, fr} &= 12.55680~mathrm{m}
end{align*}
$$

Step 5

5 of 6

For the observer, the same position-time equation can be used but with a time shift of $t_{1} = sqrt{frac{2x_{1}}{g}}$ from equation (1). We have

$$
begin{align*}
x_text{f, obs} &= frac{1}{2}gleft( t – t_{1} right)^{2} \
&= frac{1}{2}gleft( t – sqrt{frac{2x_{1}}{g}} right)^{2} \
&= frac{1}{2} left( 9.81~mathrm{m/s^{2}} right) left( 1.6~mathrm{s} – sqrt{ frac{2 left( 2.0~mathrm{m} right)}{9.81~mathrm{m/s^{2}}} } right)^{2} \
x_text{f, obs} &= 4.53101~mathrm{m}
end{align*}
$$

Step 6

6 of 6

The separation is given by

$$
begin{align*}
Delta x &= x_text{f, fr} – x_text{f, obs} \
&= 12.55680~mathrm{m} – 4.53101~mathrm{m} \
&= 8.02269~mathrm{m} \
Delta x &= boxed{ 8.0~mathrm{m} }
end{align*}
$$

The separation is indeed more than 2.0 m.

Exercise 118

Step 1

1 of 5

In this problem, a hot-air balloon is ascending at a rate $v_text{i} = +7.5~mathrm{s}$ when a passenger drops a camera. The camera is at $x_text{i} = +25~mathrm{m}$ above the ground when it was dropped. We calculate the time taken to reach the ground, and the velocity of the camera jut before it lands. We use $g = 9.81~mathrm{m/s^{2}}$, and let upwards be the positive direction.

Step 2

2 of 5

Part A.

The position-time equation of the camera is

$$
begin{align*}
x_text{f} &= x_text{i} + v_text{i}t + frac{1}{2}at^{2} \
0 &= 25~mathrm{m} + left( 7.5~mathrm{m/s} right)t – frac{9.81~mathrm{m/s^{2}}}{2}t^{2} \
implies 0 &= left( 4.905~mathrm{m/s^{2}} right) t^{2} – left( 7.5~mathrm{m/s} right)t – left( 25~mathrm{m} right) tag{1}
end{align*}
$$

Step 3

3 of 5

Equation (1) is a quadratic with roots

$$
begin{align*}
t &= -frac{1}{2left( 4.905~mathrm{m/s^{2}} right)} left[ -left(-7.5~mathrm{m/s} right) pm sqrt{ left( -7.5~mathrm{m/s} right)^{2} – 4left( 4.905~mathrm{m/s^{2}} right)left( -25~mathrm{m} right) } right] \
t &= -1.61903~mathrm{s},~3.14808~mathrm{s}
end{align*}
$$

We take the positive time, so
$$
boxed{ t = 3.1~mathrm{s} }
$$

Step 4

4 of 5

Part B.

The velocity-time equation is

$$
begin{align*}
v_text{f} &= v_text{i} + at \
&= 7.5~mathrm{m/s} – left( 9.81~mathrm{m/s^{2}} right) left( 3.14808~mathrm{s} right) \
&= -23.35118~mathrm{m/s} \
v_text{f} &= boxed{ -23~mathrm{m/s} }
end{align*}
$$

Result

5 of 5

begin{enumerate}
item [(a)] $t = 3.1~mathrm{s}$
item [(b)] $v_text{f} = -23~mathrm{m/s}$
end{enumerate}

Exercise 119

Solution 1

Solution 2

Step 1

1 of 5

In this problem, a hot-air balloon is descending at rate $v_text{i} = -2.0~mathrm{m/s}$ when a passenger drops a camera. The camera is at $x_text{i} = +45~mathrm{m}$ above the ground when it was dropped. We calculate the time taken to reach the ground, and the velocity of the camera jut before it lands. We use $g = 9.81~mathrm{m/s^{2}}$, and let upwards be the positive direction.

Step 2

2 of 5

Step 3

3 of 5

Equation (1) is a quadratic with roots

$$
begin{align*}
t &= -frac{1}{2left( 4.905~mathrm{m/s^{2}} right)} left[ -left(2.0~mathrm{m/s} right) pm sqrt{ left( 2.0~mathrm{m/s} right)^{2} – 4left( 4.905~mathrm{m/s^{2}} right)left( -45~mathrm{m} right) } right] \
t &= -3.23964~mathrm{s},~2.83189~mathrm{s}
end{align*}
$$

We take the positive time, so
$$
boxed{ t = 2.8~mathrm{s} }
$$

Step 4

4 of 5

Part B.

The velocity-time equation is

$$
begin{align*}
v_text{f} &= v_text{i} + at \
&= -2.0~mathrm{m/s} – left( 9.81~mathrm{m/s^{2}} right) left( 2.83189~mathrm{s} right) \
&= -29.78084~mathrm{m/s} \
v_text{f} &= boxed{ -30.~mathrm{m/s} }
end{align*}
$$

Result

5 of 5

begin{enumerate}
item [(a)] $t = 2.8~mathrm{s}$
item [(b)] $v_text{f} = -30.~mathrm{m/s}$
end{enumerate}

Step 1

1 of 5

To compute the time of the camera to reach the ground, its velocity before hitting the ground should first be computed.
Also, since the upward direction is to be the positive direction

$$
a = -g = -9.81m/s^2
$$

Step 2

2 of 5

To solve for the final velocity, we use

$$
v_{f}^{2} = v_{i}^{2} + 2gDelta x
$$

$$
v_{f}^{2} = 2^{2} + 2(-9.81m/s^2)(-45m)
$$

$$
v_{f} = sqrt{884.9m^2/s^2}
$$

$$
v_{f} = 29.75m/s
$$

Step 3

3 of 5

Next, to compute for the time, we use

$$
v_{f} = v_{i} + at
$$

Rearranging,

$$
t = dfrac{v_{f} – v_{i}}{a}
$$

Step 4

4 of 5

Since upward is the positive direction, we will use $v_{f} = 29.75m/s$ and $v_{i} = -2m/s$ since they are in downward motion.

$$
t = dfrac{-29.75m/s – (-2m/s)}{-9.81m/s^2} = 2.8 s
$$

Result

5 of 5

$$
t = 2.8 s
$$

Exercise 120

Step 1

1 of 5

In this problem, a car starts from rest and moves with acceleration $a_{0}$ for a time $t_{0}$. It covers a distance $x = 5~mathrm{m}$. We find the distance covered with the acceleration and time are changed to (a) $2a_{0}$ and $2t_{0}$, and (b) $4a_{0}$ and $0.5t_{0}$.

Step 2

2 of 5

The distance covered is

$$
begin{align*}
x &= frac{1}{2}a_{0}t_{0}^{2} \
implies frac{1}{2}a_{0}t_{0}^{2} &= 5~mathrm{m} tag{1}
end{align*}
$$

Step 3

3 of 5

Part A.

For this part, the distance covered is

$$
begin{align*}
x_{a} &= frac{1}{2} left( 2a_{0} right) left( 2t_{0} right)^{2} \
&= left[ frac{1}{2}a_{0}t_{0}^{2} right] left(2right) left( 2 right)^{2} \
&= left( 5~mathrm{m} right) left( 8 right) \
x_{a} &= boxed{ 40~mathrm{m} }
end{align*}
$$

Step 4

4 of 5

Part B.

For this part, the distance covered is

$$
begin{align*}
x_{b} &= frac{1}{2} left( 4a_{0} right) left( 0.5t_{0} right)^{2} \
&= left[ frac{1}{2}a_{0}t_{0}^{2} right] left(4right) left( 0.5 right)^{2} \
&= left( 5~mathrm{m} right) left( 1 right) \
x_{b} &= boxed{ 5~mathrm{m} }
end{align*}
$$

Result

5 of 5

begin{enumerate}
item [(a)] $x_{a} = 40~mathrm{m}$
item [(b)] $x_{b} = 5~mathrm{m}$
end{enumerate}

Exercise 121

Step 1

1 of 3

In this problem, a lava bomb that is projected straight upward took $t = 2.38~mathrm{s}$ to reach the peak. Its acceleration is $g = 9.81~mathrm{m/s^{2}}$ downward. We calculate its initial speed.

Step 2

2 of 3

We let upward be the positive direction. Its velocity at the peak is $v_text{f} = 0$. We have

$$
begin{align*}
v_text{f} &= v_text{i} + at \
implies v_text{i} &= v_text{f} – at \
&= 0 – left( -9.81~mathrm{m/^{2}} right) left( 2.38~mathrm{s} right) \
&= 23.34780~mathrm{m/s} \
v_text{i} &= boxed{ 23.3~mathrm{m/s} }
end{align*}
$$

Result

3 of 3

$$
v_text{i} = 23.3~mathrm{m/s^{2}}
$$

Exercise 122

Step 1

1 of 3

In this problem, ball $A$ is dropped from rest and ball $B$ is thrown upward with initial velocity $v_text{i}$. In the given velocity-time graphs, we choose which represents balls $A$ and $B$.

Step 2

2 of 3

Part A.

Ball $A$ has 0 initial velocity. Since downward direction is positive and it has a constant downward acceleration, its graph must have a positive slope. The only graph that follow this is $textbf{graph 3}$.

Step 3

3 of 3

Part B.

Ball $B$ has initial velocity $v_text{i}$, so its velocity at $t = 0$ is $v_text{i}$. It has the same acceleration as ball $A$, so the graph must have the same slope as graph 3. The only graph that satisfies these conditions is $textbf{graph 2}$.

Exercise 123

Step 1

1 of 4

In this problem, a pitcher throws her glove straight upward with velocity $v_text{i} = 6.0~mathrm{s}$. We calculate the time it takes to reach the maximum height, and the time taken to return to the pitcher. We use $g = 9.81~mathrm{m/s^{2}}$.

Step 2

2 of 4

Part A.

The velocity at the peak is $v_text{f} = 0$. With upwards begin positive, we have

$$
begin{align*}
v_text{f} &= v_text{i} + at \
implies t &= frac{v_text{f} – v_t{i}}{a} \
&= frac{0 – 6.0~mathrm{m/s}}{-9.81~mathrm{m/s^{2}}} \
&= 0.61162~mathrm{s} \
t &= boxed{ 0.61~mathrm{s} }
end{align*}
$$

Step 3

3 of 4

Part B.

With the symmetry of the problem, the time traveling upwards must be equal to the time traveling downwards. Hence, the time to return to the pitcher is twice the time moving up, so we have
$$
boxed{ t = 1.2~mathrm{s} }
$$

Result

4 of 4

begin{enumerate}
item [(a)] $t = 0.61~mathrm{s}$
item [(b)] $t = 1.2~mathrm{s}$
end{enumerate}

Exercise 124

Step 1

1 of 3

In this problem, a carpenter accidentally drops a hammer from the roof. As the hammer falls, it passes two windows of equal height. We compare the $textit{increase}$ in speed of the hammer in the two windows.

Step 2

2 of 3

Part A.

As the hammer falls down, it speeds up. This means that it takes a shorter amount of time for the hammer to pass window 2 (the one at the bottom) than window 1. Since the hammer accelerates in a shorter amount of time, the increase in speed in window 2 is less than in window 1. Hence, the increase in speed of the hammer as it passes window 1 is $textbf{greater than}$ the increase in speed as it drops past window 2.

Step 3

3 of 3

Part B.

From the previous part, we see that the correct answer in this part is $textbf{C.}$ “the hammer pends more time dropping past window 1.”

Exercise 125

Step 1

1 of 3

In this problem, we are given the velocity-time graph of the hammer in the problem 124. Shaded are the regions corresponding to the time intervals in which the hammer passes the two windows. We compare the areas.

Step 2

2 of 3

Part A.

The area under the curve of a velocity-time graph is the $textbf{displacement}$. The two windows have the same height, so the displacement of the hammer must be equal for the two hammers. Hence, the area of the shaded region corresponding to window 1 is $textbf{equal}$ to the area of the shaded region corresponding to window 2.

Step 3

3 of 3

Part B.

From the previous part, the best explanation is $textbf{B.}$ “the windows are equally tall.”

Exercise 126

Step 1

1 of 4

In this problem, an elevator is descending with constant velocity $v_text{i} = 3.0~mathrm{m/s}$ when the observer drops the book. We calculate the time for the book to hit the floor, which is $h = 1.2~mathrm{m}$ below the drop off point, and the speed of the book relative to the observer. We use $g = 9.81~mathrm{m/s^{2}}$.

Step 2

2 of 4

Part A.

Initially, the observer, elevator, and the book are all traveling with the same velocity. When we view them from the perspective of the same velocity, the book is free-falling from rest with respect to the elevator. Relative to the elevator and the observer, the book has 0 initial velocity. Hence, we can use the following equation

$$
begin{align*}
h &= frac{1}{2}gt^{2} \
implies t &= sqrt{frac{2h}{g}} \
&= sqrt{frac{2 left( 1.2~mathrm{m} right)}{9.81~mathrm{m/s^{2}}}} \
&= 0.49462~mathrm{s} \
t &= boxed{ 0.49~mathrm{s} }
end{align*}
$$

Step 3

3 of 4

Part B.

The velocity-time equation relative to the observer is

$$
begin{align*}
v_text{f} &= v_text{i} + at \
&= 0 + left( 9.81~mathrm{m/s^{2}} right) left( 0.49462~mathrm{s} right) \
&= 4.85222~mathrm{m/s} \
v_text{f} &= boxed{ 4.9~mathrm{m/s} }
end{align*}
$$

Result

4 of 4

begin{enumerate}
item [(a)] $t = 0.49~mathrm{s}$
item [(b)] $v_text{f} = 4.9~mathrm{m/s}$
end{enumerate}

Exercise 127

Step 1

1 of 4

In this problem, the observer is driving through town at $v_text{i} = 16~mathrm{m/s}$ applies the brakes and begin decelerating with $a = -3.2~mathrm{m/s^{2}}$. We calculate the time for the vehicle to stop, and the speed after half the stopping time.

Step 2

2 of 4

Part A.

We use the velocity-time equation of the vehicle. When the vehicle stops, the velocity is $v_text{f} = 0$.

$$
begin{align*}
v_text{f} &= v_text{i} + at \
implies t &= frac{v_text{f} – v_text{i}}{a} \
&= frac{0 – 16~mathrm{m/s}}{-3.2~mathrm{m/s^{2}}} \
t &= boxed{ 5.0~mathrm{s} }
end{align*}
$$

Step 3

3 of 4

Part B.

The velocity of a constantly accelerating object changes $textit{linearly}$ with time. Hence, when the time is halved, the change in velocity must be half of the total change. The final velocity is $0$, so at halfway the stopping time, the speed must be half the initial speed. Hence, the speed at half the stopping time is $textbf{equal}$ to $8.0~mathrm{m/s}$.

Step 4

4 of 4

Part C.

We use the velocity-time equation, with $t = 0.5left( 5.0~mathrm{s} right) = 2.5~mathrm{m/s}$.

$$
begin{align*}
v_text{f} &= v_text{i} + at \
&= 16~mathrm{m/s} + left( -3.2~mathrm{m/s^{2}} right) left( 2.5~mathrm{s} right) \
v_text{f} &= boxed{ 8.0~mathrm{m/s} }
end{align*}
$$

The speed is indeed equal to $8.0~mathrm{m/s}$

Exercise 128

Step 1

1 of 4

In this problem, a boat is cruising in a straight line at constant speed $v_text{i} = 2.6~mathrm{m/s}$ when it shifted into neutral (with constant acceleration). It coasts $x_text{f} = 12~mathrm{m}$, then it is resumes cruising at constant speed $v_text{f} = 1.6~mathrm{m/s}$. Assuming that the acceleration is constant, we calculate the time taken to coast the $x_text{f} = 12~mathrm{m}$, the acceleration of the boat, and the speed of the boat at halfway the distance.

Step 2

2 of 4

Part A.

Since the acceleration is constant, we can use the average velocity of the boat. We have

$$
begin{align}
x_text{f} &= x_text{i} + v_text{av}t \
implies t &= frac{x_text{f} – x_text{i}}{v_text{av}} \
&= frac{12~mathrm{m} – 0}{frac{2.6~mathrm{m/s} + 1.6~mathrm{m/s}}{2}} \
&= 5.71429~mathrm{s} \
t &= boxed{ 5.7~mathrm{s} }
end{align}
$$

Step 3

3 of 4

Part B.

The acceleration is given by

$$
begin{align*}
a &= frac{Delta v}{Delta t} \
&= frac{1.6~mathrm{m/s} – 2.6~mathrm{m/s}}{5.71429~mathrm{s}} \
&= -0.17500~mathrm{m/s^{2}} \
a &= boxed{ -0.18~mathrm{m/s^{2}} }
end{align*}
$$

Step 4

4 of 4

Part C.

Since the boat is decelerating, it must be faster in the first half of the distance coasted than in the second half. It should have coasted $6.0~mathrm{m}$ before half the total time of the travel. The speed changes linearly, and since the time of travel for this part is less than half the total time, the speed must still be $textbf{greater than}$ the average speed of $2.1~mathrm{m/s}$. Notice that the speed is greater than because it starts from higher to lower, but it has not yet reached the midpoint.

Exercise 129

Step 1

1 of 3

In this problem, the velocity-time graph of the a car is given. It is also given that the displacement of the car in the given time period is $13~mathrm{m}$ in $t_{1} = 8.0~mathrm{s}$. We find the speed $V$ labeled in the graph. The constant speed happens for a time $t_{2} = 6 – 4 = 2.0~mathrm{s}$.

Step 2

2 of 3

The displacement of an object is equal to the area bounded by the velocity-time graph. The graph is a trapezoid, and we know its area must be

$$
begin{align*}
Delta x &= V frac{t_{1} + t_{2}}{2} \
implies V &= frac{2Delta x}{t_{1} + t_{2}} \
&= frac{2 left( 13~mathrm{m} right)}{8.0~mathrm{s} + 2.0~mathrm{s}} \
V &= boxed{ 2.6~mathrm{m/s} }
end{align*}
$$

Result

3 of 3

$$
V = 2.6~mathrm{m/s}
$$

Exercise 130

Step 1

1 of 6

In this problem, we are given the same velocity-time graph as Item 129, but with $V = 1.5~mathrm{m/s}$. We find the distance covered in the time intervals (a) $t = 0$ to $t = 4~mathrm{s}$, (b) $t = 4~mathrm{s}$ to $t = 6~mathrm{s}$, and (c) $t = 6~mathrm{s}$ to $t = 8~mathrm{s}$.

Step 2

2 of 6

In each segment, the acceleration is constant because the velocity-time graphs are all linear. We can use the following equation to determine the distance

$$
begin{align*}
Delta x &= frac{v_text{i} + v_text{f}}{2} Delta t tag{1}
end{align*}
$$

Step 3

3 of 6

Part A.

For this part, $v_text{i, a} = 0~mathrm{m/s}$, $v_text{f, a} = 1.5~mathrm{m/s}$, and $Delta t_{a} = 4~mathrm{s}$. Using equation (1), we have

$$
begin{align*}
Delta x_{a} &= frac{v_text{i, a} + v_text{f, a}}{2} Delta t_{a} \
&= frac{0~mathrm{m/s} + 1.5~mathrm{m/s}}{2} left( 4~mathrm{s} right) \
Delta x_{a} &= boxed{ 3.0~mathrm{m} }
end{align*}
$$

Step 4

4 of 6

Part B.

For this part, $v_text{i, b} = 1.5~mathrm{m/s}$, $v_text{f, b} = 1.5~mathrm{m/s}$, and $Delta t_{b} = 2~mathrm{s}$. Using equation (1), we have

$$
begin{align*}
Delta x_{b} &= frac{v_text{i, b} + v_text{f, b}}{2} Delta t_{b} \
&= frac{1.5~mathrm{m/s} + 1.5~mathrm{m/s}}{2} left( 2~mathrm{s} right) \
Delta x_{b} &= boxed{ 3.0~mathrm{m} }
end{align*}
$$

Step 5

5 of 6

Part C.

For this part, $v_text{i, c} = 1.5~mathrm{m/s}$, $v_text{f, c} = 0~mathrm{m/s}$, and $Delta t_{c} = 2~mathrm{s}$. Using equation (1), we have

$$
begin{align*}
Delta x_{c} &= frac{v_text{i, c} + v_text{f, c}}{2} Delta t_{c} \
&= frac{1.5~mathrm{m/s} + 0~mathrm{m/s}}{2} left( 2~mathrm{s} right) \
Delta x_{c} &= boxed{ 1.5~mathrm{m} }
end{align*}
$$

Result

6 of 6

begin{enumerate}
item [(a)] $Delta x_{a} = 3.0~mathrm{m}$
item [(b)] $Delta x_{b} = 3.0~mathrm{m}$
item [(c)] $Delta x_{c} = 1.5~mathrm{m}$
end{enumerate}

Exercise 131

Step 1

1 of 4

In this problem, astronauts from a distant planet throw a rock straight upward and record its height-time graph. We calculate the acceleration due to gravity $a$, and the initial speed $v_text{i}$ of the rock.

Step 2

2 of 4

Part A.

Consider the motion from $t = 4~mathrm{s}$ to $t = 8~mathrm{s}$. The velocity at the top must be $v_text{i} = 0$. The rock is in free fall, and the cover the height $h = 30~mathrm{m}$ in $t = 4~mathrm{s}$. We use the following equation

$$
begin{align*}
h &= frac{1}{2}at^{2} \
implies a &= frac{2h}{t^{2}} \
&= frac{2 left( 30~mathrm{m} right)}{left( 4~mathrm{s} right)^{2}} \
a &= boxed{ 3.75~mathrm{m/s^{2}} }
end{align*}
$$

Step 3

3 of 4

Part B.

Consider the motion from $t = 0~mathrm{s}$ to $t = 4~mathrm{s}$. The ball is thrown initially upward with velocity $v_text{i}$. At the top, its velocity must be $v_text{f} = 0$, and the constant acceleration due to gravity is downward. We let upwards be the positive direction. The velocity-time equation must be

$$
begin{align*}
v_text{f} &= v_text{i} + at \
implies v_text{i} &= v_text{f} – at \
&= 0 – left( -3.75~mathrm{m/s^{2}} right) left( 4~mathrm{s} right) \
v_text{i} &= boxed{ 15.0~mathrm{m/s} }
end{align*}
$$

Result

4 of 4

begin{enumerate}
item [(a)] $a = 3.75~mathrm{m/s^{2}}$
item [(b)] $v_text{i} = 15.0~mathrm{m/s}$
end{enumerate}

Exercise 132

Step 1

1 of 6

In this problem, a car and truck are heading directly toward one another on a straight line. They avoid head-on collision by hitting the brakes at $t = 0$ until they stop. The velocity-time graph are given. Initially, the car is at $x_text{i, car} = 15~mathrm{m}$ and the truck is at $x_text{i, tr} = -35~mathrm{m}$.

Step 2

2 of 6

Based on the given positions, the object on the left must have a positive initial velocity and the object on the right has a negative initial velocity for collision to happen. From the graph, $v_text{i, car} = -15~mathrm{m/s}$ and $Delta t_text{car} = 3.5~mathrm{s}$, and for the truck we have $v_text{i, tr} = +10~mathrm{m/s}$ and $Delta t_text{tr} = 2.5~mathrm{s}$.

Step 3

3 of 6

First, we find the final position of the car. The position-time equation is

$$
begin{align*}
x_text{f, car} &= x_text{i, car} + frac{v_text{i, car} + v_text{f, car}}{2}Delta t_text{car} \
&= 15~mathrm{m} + frac{-15~mathrm{m/s} + 0}{2} left( 3.5~mathrm{s} right) \
x_text{f, car} &= -11.25~mathrm{m}
end{align*}
$$

Step 4

4 of 6

The final position of the truck is given by

$$
begin{align*}
x_text{f, tr} &= x_text{i, tr} + frac{v_text{i, tr} + v_text{f, tr}}{2}Delta t_text{tr} \
&= -35~mathrm{m} + frac{10~mathrm{m/s} + 0}{2} left( 2.5~mathrm{s} right) \
x_text{f, tr} &= -22.50~mathrm{m}
end{align*}
$$

Step 5

5 of 6

The separation of the car and truck is

$$
begin{align*}
Delta x &= x_text{f, car} – x_text{f, tr} \
&= -11.25~mathrm{m} – left( -22.50~mathrm{m} right) \
&= 11.25~mathrm{m} \
Delta x &= boxed{ 11~mathrm{m} }
end{align*}
$$

Result

6 of 6

$$
Delta x = 11~mathrm{m}
$$

Exercise 133

Step 1

1 of 2

In this problem, we report the acceleration due to gravity of various objects in the Solar system, and we compare them with the acceleration due to gravity on the Earth.

Step 2

2 of 2

Some of the acceleration due to gravity on some planets, moons, and asteroids are
begin{center}
begin{tabular} {|c|c|c|}
hline
Object & $a~mathrm{in~m/s^{2}}$ & Relative to Earth’s $g$ \ hline
Moon & $1.62$ & $0.166g$ \ hline
Titan & $1.40$ & $0.143g$ \ hline
Mars & $3.70$ & $0.377g$ \ hline
Jupiter & $24.5$ & $2.50g$ \ hline
Vesta & $0.220$ & $0.0224g$ \ hline
end{tabular}
end{center}

Exercise 134

Step 1

1 of 3

In this problem, we compare the free-fall of astronauts in orbit and of an elevator that drops after the cable breaks.

Step 2

2 of 3

For the astronauts, both the astronaut and the spaceship are free falling with the same acceleration. Hence, relative to the spaceship, the astronaut has 0 acceleration and feels “weightless.”

Step 3

3 of 3

For the elevator, both the person and elevator are accelerating with the same acceleration. Relative to the elevator, the observer has 0 acceleration and thus feels “weightless.”

Exercise 135

Step 1

1 of 3

In this problem, we calculate the time taken for the lander to drop the final 1.31 m to the moon surface.

Step 2

2 of 3

From the graph, the initial position happens at $t_{1} = 8.6~mathrm{s}$ and the final position at time $t_{2} = 9.8~mathrm{s}$. The time interval is

$$
begin{align*}
Delta t &= t_{2}- t_{1} \
&= 9.8~mathrm{s} – 8.6~mathrm{s} \
Delta t &= 1.2~mathrm{s}
end{align*}
$$

Among the choices, the closest value is
$$
boxed{ mathrm{A.~1.18~s} }
$$

Result

3 of 3

$$
mathrm{A.~1.18~s}
$$

Exercise 136

Step 1

1 of 3

In this problem, we calculate the impact speed of the lander. It has initial speed $v_text{i} = 0.152~mathrm{m/s}$ and accelerating at a rate $a = 1.62~mathrm{m/s^{2}}$. The displacement is $Delta x = 1.31~mathrm{m}$.

Step 2

2 of 3

The velocity-time equation must be

$$
begin{align*}
v_text{f}^{2} &= v_text{i}^{2} + aDelta x \
v_text{f} &= sqrt{v_text{i}^{2} + aDelta x} \
&= sqrt{ left( 0.152~mathrm{m/s} right)^{2} + 2 left( 1.62~mathrm{m/s^{2}} right) left( 1.31~mathrm{m} right) } \
&= 2.06579~mathrm{m/s} \
v_text{f} &= 2.07~mathrm{m/s}
end{align*}
$$

The impact speed is
$$
boxed{ v_text{f} = 2.07~mathrm{m/s} }
$$

Result

3 of 3

$$
text{B}. 2.07~mathrm{m/s}
$$

unlock

All Solutions

Page 105: Assessment

Haven't found what you were looking for?

Search for samples, answers to your questions and flashcards