Range is $left{ 2,3,4,5right}$

The value 2 of the independent variable maps
to two different values 3 and 4 of the dependent variable
This relation is not a function.

Range is $left{ -1,1,3,4right}$

This relation is a function because each element of the domain corresponds with only one element in the range

For example, if $x=4$, then $y=-(4-3)^{2}+5=-1^{2}+5=-1+5=4$

For example, if $x=13$, then $y=sqrt{13-4}=sqrt{9}=3$

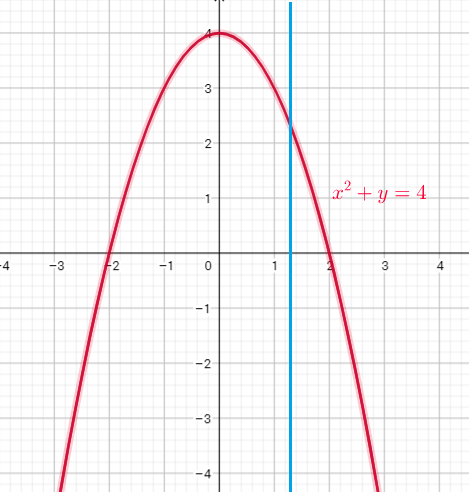
Substitute $x=0$ into equation $x^{2}+y=4$, now we get:

$0^{2}+y=4$

$0+y=4$

$y=4$

Substitute $x=-2$ into equation $x^{2}+y=4$, now we get:

$(-2)^{2}+y=4$

$4+y=4$

$y=4-4$

$y=0$

This is a function because for each value $x$ we get only one value of $y$.

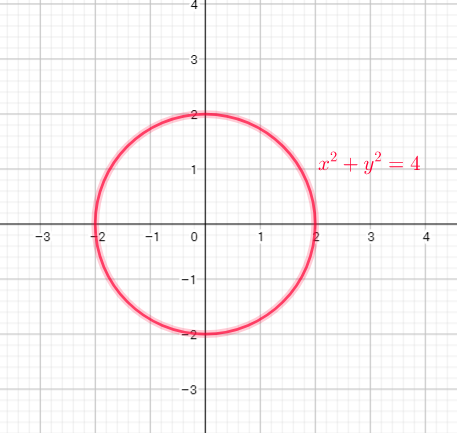
Substitute $x=0$ into equation $x^{2}+y^{2}=4$, now we get:

$0^{2}+y^{2}=4$

$y^{2}=4$

$y=pm sqrt{4}$

$y=pm2$

so, if $x=0$, then , $y=-2$ or $y=2$

Substitute $x=-2$ into equation $x^{2}+y^{2}=4$, now we get:

$(-2)^{2}+y^{2}=4$

$4+y^{2}=4$

$y^{2}=0$

$y=0$

This is a not a function because for $x=0$ we get two values of $y$

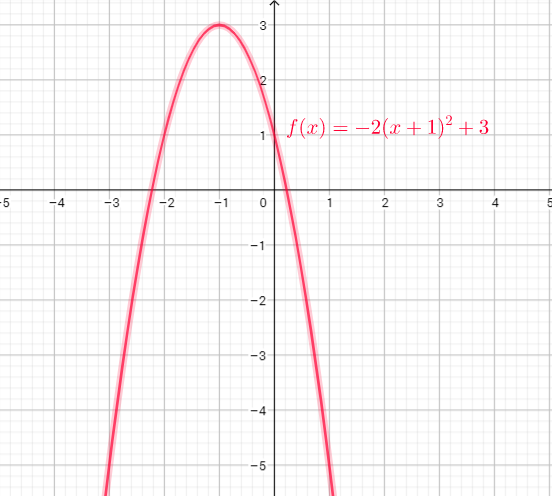
If $x=-3$, then
$f(-3)=-2(-3+1)^{2}+3=-2(-2)^{2}+3=-2cdot4+3=-8+3=-5$

That we can see from the graph: function passes through a point $(-3,-5)$

i) The first , we need determine $f(1)$ and $f(0)$

If $x=0$, then $f(0)=-2(0+1)^{2}+3=-2cdot1^{2}+3=-2+3=1$

If $x=1$, then $f(1)=-2(1+1)^{2}+3=-2cdot2^{2}+3=-8+3=-5$

Now, can determine:

$f(1)-f(0)=-5-1=-6$

ii) We need determine $f(2)$

If $x=2$, then $f(2)=-2(2+1)^{2}+3=-2cdot3^{2}+3=-18+3=-15$

Now can calculate:

$3cdot f(2)-5=3cdot(-15)-5=-45-5=-50$

iii) $f(2-x)=-2(2-x+1)^{2}+3=-2cdot(3-x)^{2}+3$

b) $-5$

c) $y$-coordinate on the graph corresponding to $x=-3$

d) i) $-6$ ii) $-50$ iii) $-2(3-x)^2+3$

Function is $f(x)=(20-5x)x$

$f(1)=(20-5cdot1)cdot1=(20-5)cdot1=15cdot1=15$

To find $f(-1)$ , plug in -1 wherever $x$ occures in the equation of $f(x)$ and simplify to get $f(-1)$

$f(-1)=(20-5cdot(-1))cdot(-1)=(20+5)cdot(-1)=25cdot(-1)=-25$

To find $f(7)$ , plug in 7 wherever $x$ occures in the equation of $f(x)$ and simplify to get $f(7)$

$f(7)=(20-5cdot7)cdot7=(20-35)cdot7=-15cdot7=-105$

Our quadratic function is $f(x)=(20-5x)x$ or $f(x)=-5x^{2}+20x$

There is $a=-5,$ and $b=20$. Now can calculate:

$dfrac{-b}{2a}=dfrac{-20}{2(-5)}=dfrac{-20}{-10}=2$

Now we need calculate $f(-dfrac{b}{2a})$:

$f(dfrac{-b}{2a})=f(2)=(20-5cdot2)cdot2=(20-10)cdot2=10cdot2=20$

Now can conclude: Quadratic function
$f(x)=(20-5x)x$ has maximum in the point $(2,20)$. That means, the maximum result possible is 20.

b) 15, $-25$, $-105$

c) 20

Domain =$left{xin R right}$

Range=$left{ yin R| ygeq0right}$

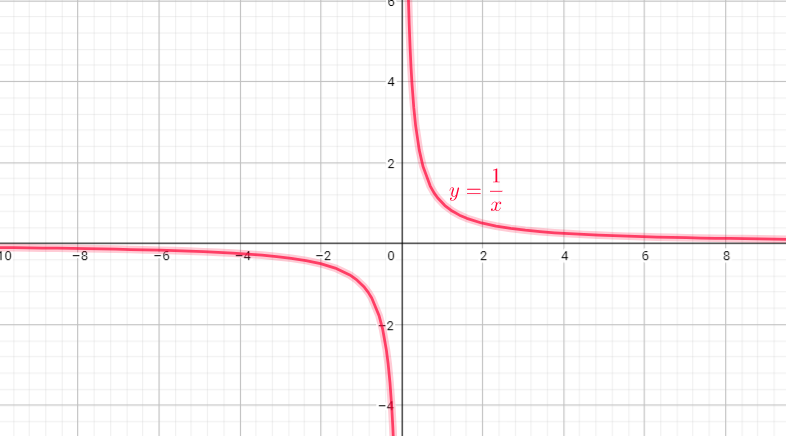
Domain =$left{xin R | xne 0right}$

Range=$left{ yin R| yne0 right}$

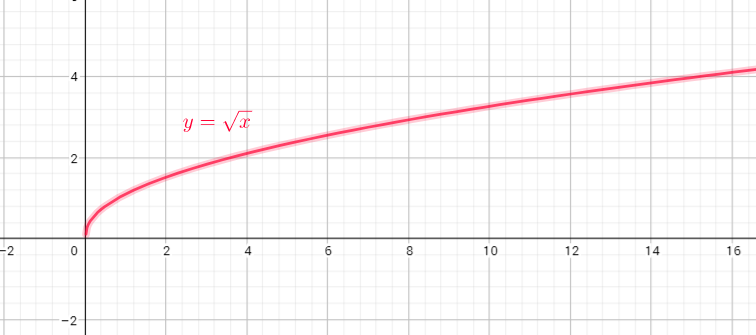
Domain=$left{ xin R| xgeq0right}$

Range=$left{ yin R| ygeq0right}$

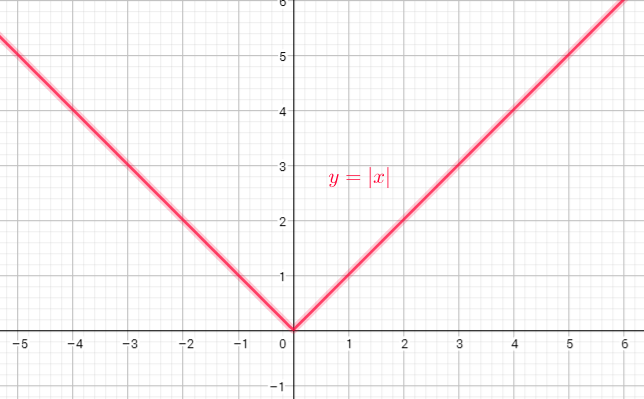
Domain=$left{ xin Rright}$

Range=$left{ yin R| ygeq0right}$

range = ${ yin bold{R};|;ygeq 0}$

b) domain = ${ xinbold{R};|;xneq 0}$

range = ${ yinbold{R};|;yneq 0}$

c) domain = ${ xinbold{R};|;xgeq 0}$

range = ${ yinbold{R};|;ygeq 0}$

d) domain = ${xinbold{R}}$

range = ${ yinbold{R};|;ygeq 0}$

Domain=$left{ 1,2,4right}$

Range=$left{ 2,3,4,5right}$

Domain=$left{ -2,0,3,7right}$

Range=$left{-1,1,3,4 right}$

Domain=$left{ -4,-3,-2,-1,0,1,2,3,4,5right}$

Range=$left{ -4,-3,-2,-1,0,1,2,3,4,5right}$

Domain=$left{ xin R| xgeq-3right}$

Range=$left{yin Rright}$

Domain=$left{xin R right}$

Range=$left{ yin R| yleq5right}$

Domain=$left{ xin R| xgeq4right}$

Range=$left{yin R| ygeq0 right}$

b) domain = ${$ -2, 0, 3, 7 $}$ , range = ${$ $-1$, 1, 3, 4 $}$

c) domain = ${ -4$, $-3$, $-2$, $-1$, 0, 1, 2, 3, 4, 5 $}$

range = ${ -4$, $-3$, $-2$, $-1$, 0, 1, 2, 3, 4, 5 $}$

d) domain = ${ xinbold{R};|;xgeq -3}$ ,
range = ${yinbold{R}}$

e) domain = ${ xinbold{R}}$ , range = ${ yinbold{R};|;yleq 5}$

f) domain = ${ xinbold{R};|;xgeq 4}$, range = ${ yinbold{R};|;ygeq 0}$

$4w+2l=600$

Express $l$ in terms of $w$

$l=dfrac{600-4w}{2}$

The area of a rectangle is $ltimes w$

$A=lw$

$A=left( dfrac{600-4w}{2}right)w$

$$
A=w(300-2w)
$$

We also know that both width $w$ and area $A$ must be more than zero. The upper limit can be obtained from the graph.

domain = ${ winbold{R};|;0<w<150}$

range = ${ Ainbold{R};|;0<Aleq 11,250}$

This corresponds to

$l=dfrac{600-4w}{2}=300-2w=(300-2times 75)=150$ m

b) domain = ${ winbold{R};|;0<w<150}$

range = ${ Ainbold{R};|;0<Aleq 11,250}$

c) $l=150$ m , $w=75$ m

Domain=$left{ xin Rright}$

Range=$left{ yin R| yleq5right}$

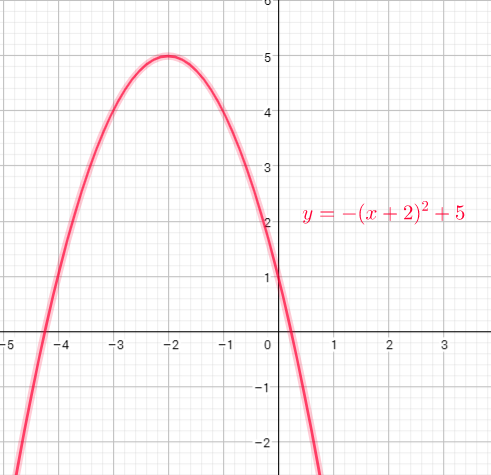
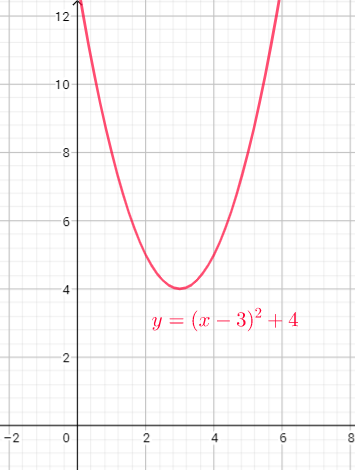
Domain=$left{xin R right}$

Range=$left{ yin R| ygeq4right}$

Range=$left{ yin R|-7leq yleq7 right}$

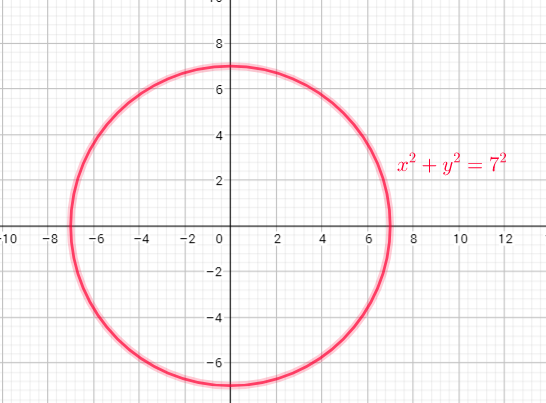
Range=$left{ yin R|1leq yleq9 right}$

b) domain = ${ xinbold{R}}$ , range = ${ yinbold{R};|;ygeq 4}$

c) domain = ${ xinbold{R};|;-7leq xleq 7}$

range = ${ yinbold{R};|;-7leq yleq 7}$

d) domain = ${ xinbold{R};|;-2leq xleq 6}$

range = ${ yinbold{R};|;1leq y leq 9}$