Before this task there is explanation that if $left(x,y right)$ represents a point on the graph of $f$ then $left(y,x right)$ represents a point on the graph of the corresponding $f^{-1}$. So, according to this, we have following solutions:

#### (a)

$left(5,2 right)$

#### (b)

$left(-6,-5 right)$

#### (c)

$left(-8,4 right)$

#### (d)

Here we have that our $textbf{origin point}$ is $left(1,2 right)$, so, the $textbf{inverse point}$ is $left(2,1 right)$.

#### (e)

Here we have that our $textbf{origin point}$ is $left(-3,0 right)$, so, the $textbf{inverse point}$ is $left(0,-3 right)$.

#### (f)

Here we have that our $textbf{origin point}$ is $left(0,7 right)$, so, the $textbf{inverse point}$ is $left(7,0 right)$.

Earlier in this book, before tasks, there is explanation that $textbf{the domain of a function is the range of its inverse and the range of a function is the domain of its inverse}$.

So, according to this, there are following solutions of this task:

#### (a)

$D=Bbb{R}$

$R=Bbb{R}$

#### (b)

$D=Bbb{R}$

$R=left[2,infty right)$

#### (c)

$D=left(-infty,2 right)$

$R=left[-5,infty right)$

#### (d)

$D=left(-5,10 right)$

$R=left(-infty,2 right)$

We have to know that it is $textbf{the graph of the inverse function is a reflection in the line $y=x$}$.

So, according to this, we have following pairs:

$A-D$, $B-F$, $C-E$

#### (a)

To find this point, first we have to find $f(4)$. So, let’s find it!

$f(4)=2cdot4^3+1=2cdot64+1=128+1=129$

$textbf{The required point is $left(4,129 right)$}$.

#### (b)

$textbf{The ordered pair on the inverse relation that corresponds to
the ordered pair $left(4,129 right)$ is point $left(129,4 right)$}$

The explanation is in the task number 1.

#### (c)

$textbf{The doamin and range of $f$ is state $Bbb{R}$}$.

#### (d)

According to explanation in task number 2, $textbf{(the daomain and range of inverse function is also state $Bbb{R}$}$.

#### (e)

$textbf{The inverse relation is a function}$, we can see that by forming table of values, switching the independent and dependent variables in
the table of values.

#### (a)

To find this point, first we have to find $f(4)$. So, let’s find it!

$f(4)=4^4-8=256-8=248$

$textbf{The required point is $left(4,248 right)$}$.

#### (b)

$textbf{The ordered pair on the inverse relation that corresponds to
the ordered pair $left(4,248 right)$ is point $left(248,4 right)$}$

The explanation is in the task number 1.

#### (c)

$textbf{The doamin and range of $f$ are}$:

$D=Bbb{R}$

$R=left[-8,infty right)$

#### (d)

According to explanation in task number 2, $textbf{the domain and range of inverse function are}$:

$D=left[-8,infty right)$

$R=Bbb{R}$

#### (e)

Let’s first find $textbf{inverse relation:}$

$y=x^4-8$

$x=y^4-8$

$y^4=x+8$

$y=sqrt[4]{x+8}$ $wedge$ $y=-sqrt[4]{x+8}$

Here, $textbf{the inverse relation is not a
function}$, but it can be split in
the middle into the two
functions.

#### (a)

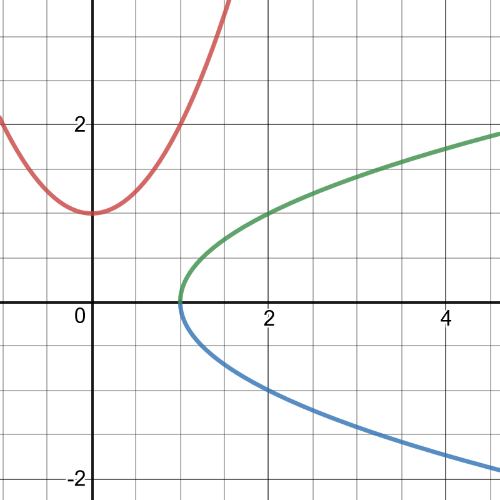
Let’s first find $textbf{inverse relation}$:

$y=x^2+1$

$x=y^2+1$

$y^2=x-1$

$y=-sqrt{x-1}$ $wedge$ $y=sqrt{x-1}$

$textbf{This inverse relation is not a function}$, but it can be split on two functions. On the following graph red one is graph of $y=x^2+1$, blue one is of $y=-sqrt{x-1}$ and green one is of $y=sqrt{x-1}$:

#### (b)

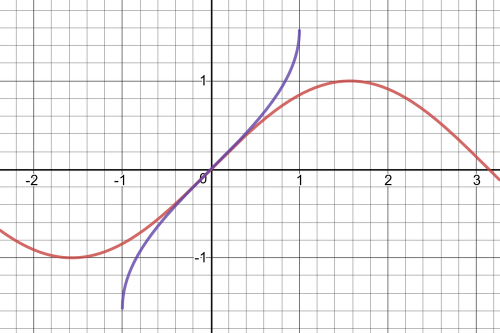
Let’s first find $textbf{inverse relation}$. $textbf{Inverse relation of this function on this domain is also function and it is function}$ $y=arcsin{x}$

On the following graph, red one is graph ofthe function $y=sin{x}$ and purple one is of inverse function, $y=arcsin{x}$:

#### (c)

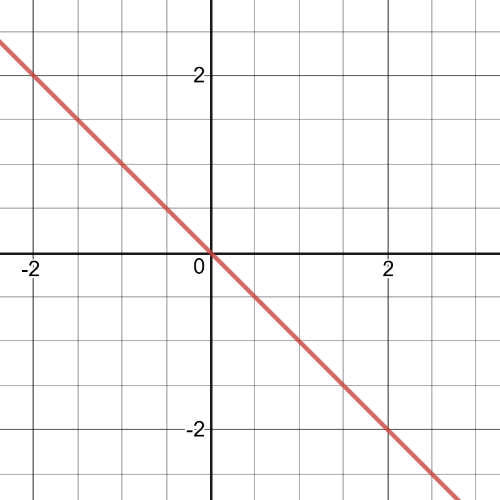
Here, we have:

$y=-x$

$x=-y$

$y=-x$

We can see that $textbf{inverse relation is actually origin function}$, and according to this, it is function. So, the graph of origin and inverse function will bw the graph of the same function, $y=-x$, which follows:

#### (d)

Let’s first find inverse relation:

$y=left|x right|+1$

$x=left|y right|+1$

$left|y right|=x-1$

According to the definition of absolute value, here we have two functions, and also, according to this, we conclude that this $textbf{inverse relation is not a function}$:

$y=x-1$ $wedge$ $y=-(x-1)$

On the following graph, red one is graph of the $y=left|x right|+1$, black one is of $y=x-1$ and green one is of $y=-(x-1)$:

#### (a)

Let’s first find $textbf{inverse relation}$:

$F=dfrac{9}{5}C+32$

$dfrac{9}{5}C=F-32$

$9C=5(F-32)$

$C=dfrac{5}{9}(F-32)$

$textbf{This equation convert Fahrenheit temperature to the equivalent Celsius temperature}$.

#### (b)

First, we will find equiavlent Fahrenheit temperature to 20°C. For this, we will use $textbf{origin relation}$, and we get:

$F=dfrac{9}{5}cdot20+32=9cdot4+32=36+32=68$

So,the answer is $68F$.

To find equivalent Celsius temperature to $68F$, we will use $textbf{transformed relation}$:

$C=dfrac{5}{9}cdotleft(68-32 right)=dfrac{5}{9}cdot36=5cdot4=20$

So,the result is $20°C$.

#### (a)

Let’s first find the $textbf{inverse relation}$:

$A=picdot{r^2}$

$r^2=dfrac{A}{pi}$

$r=sqrt{dfrac{A}{pi}}$

This inverse of the relation we use for $textbf{finding the radius when the area of the circle is known}$.

#### (b)

Because we know that the radius is $r=5$ and do not know the area of the circle, $textbf{we will use origin relation}$:

$A=picdot{r^2}=picdot5^2=25cdotpi$

So, the area of the circle is $A=25pi$

Now, if we know that the area of the circle is $A=25pi$, we will find the radius using $textbf{inverse of the relation}$:

$r=sqrt{dfrac{A}{pi}}=sqrt{dfrac{25pi}{pi}}=sqrt{25}=5$

So, the radius is $r=5$

First, we will find $textbf{inverse relation}$:

$y=kx^3+1$

$x=ky^3+1$

$ky^3=x-1$

$y^3=dfrac{x-1}{k}$

$y=sqrt[3]{dfrac{x-1}{k}}=f^{-1}(x)$

We are given in task that $f^{-1}(15)=2$, we have that:

$2=sqrt[3]{dfrac{15+1}{k}}$

$2^3=dfrac{16}{k}$

$8=dfrac{16}{k}$

$k=dfrac{16}{8}=2$

So,the final result is that $k=2$

#### (a)

$h(3)=2cdot3+7=6+7=13$

#### (b)

$h(9)=2cdot9+=18+7=25$

#### (c)

Here, we will use results from $left( aright)$ and $left( bright)$:

$dfrac{h(9)-h(3)}{9-3}=dfrac{25-13}{6}=dfrac{12}{6}=2$

Now, let’s find first $textbf{inverse relation}$:

$y=2x+7$

$x=2y+7$

$2y=x-7$

$y=dfrac{x-7}{2}=h^{-1}(x)$

#### (d)

$h^{-1}(3)=dfrac{3-7}{2}=-dfrac{4}{2}=2$

#### (e)

$h^{-1}(9)=dfrac{9-7}{2}=-dfrac{2}{2}=1$

#### (f)

Here, we will use results from $left(d right)$ and $left(e right)$:

$dfrac{h^{-1}(9)-h^{-1}(3)}{9-3}=dfrac{1+2}{6}=dfrac{1}{2}$

$textbf{The domain}$ of the inverse relation is state of overall average in all subjects, and $textbf{range}$ is a state of students. $textbf{Inverse relation is not a function}$ because it might be that some students have the same overall average in all their subjects.So, in this case,we will have that element from domain will has two or more correspond elements from range, which actually means that this inverse relation is not a function.

Let’s find $textbf{inverse}$ of each function in this task!

#### (a)

$y=3x+4$

$x=3y+4$

$3y=x-4$

$color{#c34632}{y=dfrac{x-4}{3}}$

#### (b)

$y=-x$

$x=-y$

$color{#c34632}{y=-x}$

#### (c)

$y=x^3-1$

$x=y^3-1$

$y^3=x+1$

$color{#c34632}{y=sqrt[3]{x+1}}$

#### (d)

$y=-2(x+5)=-2x-10$

$x=-2y-10$

$-2y=x+10$

$color{#c34632}{y=-dfrac{x+10}{2}}$

#### (a)

We will find $textbf{an equation for inverserelation}$ of this function:

$y=4(x-3)^2+1$

$x=4(y-3)^2+1$

$4(y-3)^2=x-1$

$(y-3)^2=dfrac{x-1}{4}$

$y-3=pmsqrt{dfrac{x-1}{4}}$

$color{#c34632}{y=pmsqrt{dfrac{x-1}{4}}+3}$

#### (b)

$textbf{Solve for $y$}$ in the equation for the inverse $g$ are:

$y=-sqrt{dfrac{x-1}{4}}$ $vee$ $y=sqrt{dfrac{x-1}{4}}$

#### (c)

Here we have graphs of function $g$ and of its inverse relaation from $left(b right)$. Red one is graph of $g$, blue one is of $y=-sqrt{dfrac{x-1}{4}}$, and green one is of $y=sqrt{dfrac{x-1}{4}}$:

#### (d)

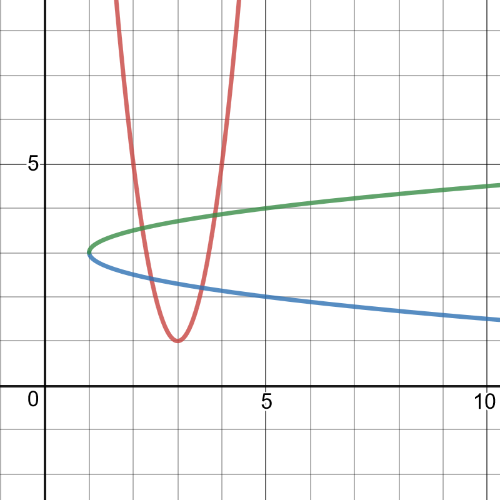
Cross points of the function $g$ and $y=-sqrt{dfrac{x-1}{4}}$,as we can see from the graph are:

$left(2.407,2.407 right)$ $wedge$ $left(3.548,2.202 right)$

Cross points of the function $g$ and $y=sqrt{dfrac{x-1}{4}}$,as we can see from the graph are:

$left(2.202,3.548 right)$ $wedge$ $left(3.843,3.843 right)$

#### (e)

$textbf{We should make restriction od domain}$, then, inverse relation will be a function. This restriction might be any of two following states or any of their subsets:

$D=left(-infty,3 right]$ or $D=left[3,infty right)$

#### (f)

On this domain, $textbf{inverse relation is not a function}$ because only with restrictions on the domain like in $left(e right)$ inverse will be a function.

Let’s first find inverse relation of $y=x^2-2$:

$x=y^2-2$

$y^2=x+2$

$color{#c34632}{y=pmsqrt{x+2}}$

In order for $y$ to be inverse, its inverse must be the starting function. But here inverse of inverse function does not exist, it can only be split into two functions, and one of them is $y=-sqrt{x+2}$, but this does not mean that it is an inverse function.

Let’s firs find inverse of function $y=sqrt{x+2}$
:

$x=sqrt{y+2}$

$x^2=y+2$

$color{#c34632}{y=x^2-2}$

$textbf{Its inverse is a function}$, we should not make a restriction either on a domain or on a range. The simplest way is to see this with the graphics of exactly this inverse function because the test of vertical lines passes.

The function that the John has said is $y=dfrac{x^3}{4}+2$

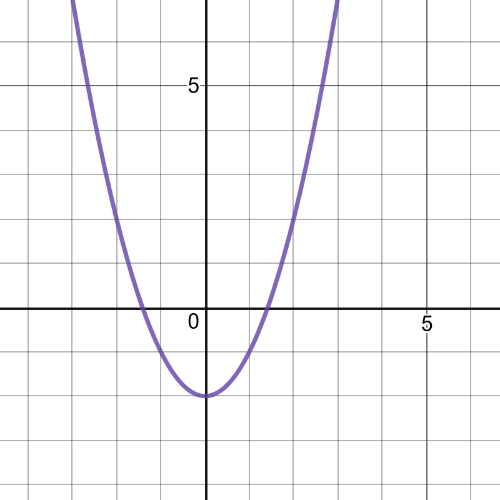
Now, $textbf{we will find its inverse step by step}$:

$x=dfrac{y^3}{4}+2$

$x-2=dfrac{y^3}{4}$

$4(x-2)=y^3$

$color{#c34632}{y=sqrt[3]{4(x-2)}}$

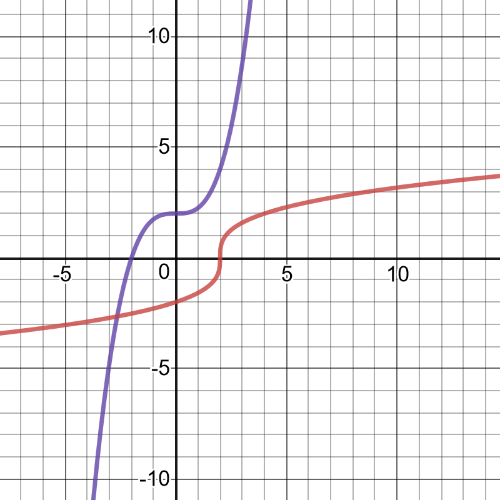
So, the answer is John was correct!

Here we have graphs of those functions, blue one is of $y=dfrac{x^3}{4}+2$ and red one is graph of inverse:

Yes, $textbf{we can find three more functions with this property}$, that would be following functions:

$f(x)=-x$

$f(x)=left|x right|$

$f(x)=-left|x right|$

And no, $textbf{we can’t convince ourselves}$ that there are an infinite number of functions that satisfy property given in this task.

If the original function graph passes the horizontal line test, this means that there are no two different points from the domain that are mapped to the same point from the range. Each point in the domain would have exactly one corresponding point from the range. $textbf{As the inverse relation maps the points from range of the original function to the corresponding points from the domain that is originally captured by the original function}$, in the case that the graph of the starting function passes the test of the horizontal lines, the inverse relation would be a function, since each point from its domain would be mapped to the right one point from the range.