$textbf{The equation of the graph is}$ $6.25pi(dfrac{x}{4})$, and it is on the following picture:

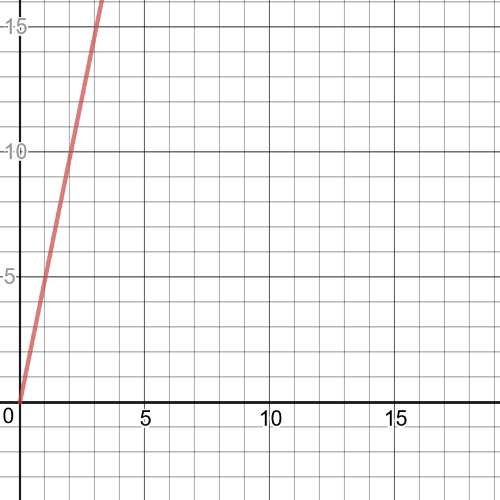
$$
y=6.25pi(dfrac{x}{4})
$$

#### (c)

$8=6.25pi(dfrac{x}{4})$

$8=1.5625pi{x}$

$1.6=x$

$textbf{Ao, it will take about $1.6$ hours}$.

$y=dfrac{6.25 pi}{64}(x-8)^2$

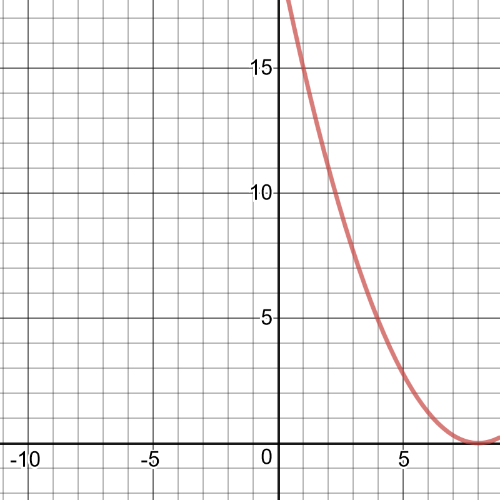
$V(t)=dfrac{6.25pi}{64}(t-8)^2$

#### (c)

$V(2)=dfrac{6.25pi}{64}(2-8)^2=dfrac{6.25pi}{64}(-6)^2=dfrac{6.25}{64}(36)=11m^3$

#### (d)

The initial volume is $19.6 m^3$.

So, the rate of change is $(11-19.6)div 2$ or $-4.3m^3/hr$

#### (e)

As time elapses, the pool is losing less water in the same amount of time.

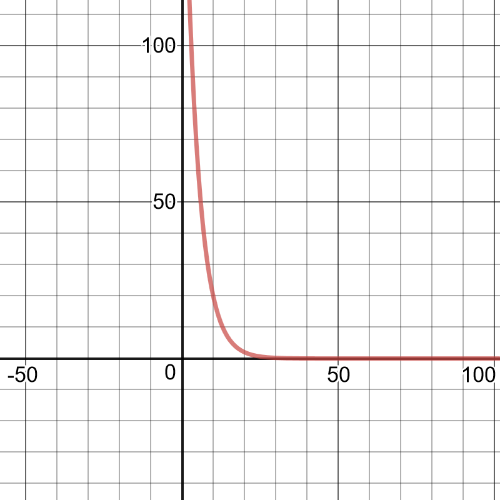
For example, we have two $textbf{following graphs}$:

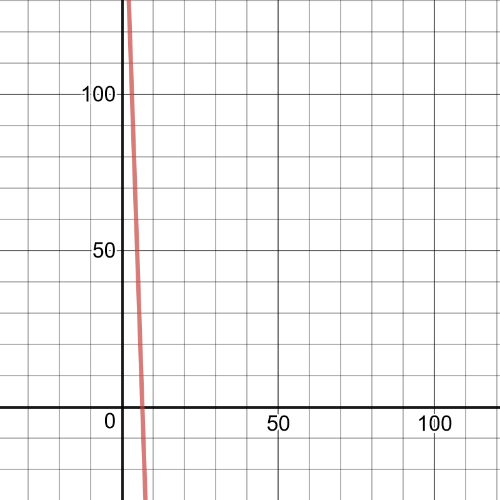
From the linear graph, the $y$-intercept is $(0,200)$ and the slope is $-30$, so, $textbf{the equation is}$:

$V(t)=-30t+200$

$0=-30t+200$

$-200=-30t$

$$
t=6.7
$$

#### (c)

Using a graphing calculator, $textbf{the equation that fits the model is}$

$V(t)=200(0.795)^t.$

$V(t)=200(0.795)^t$

$20=200(0.795)^t$

$0.1=0.765^t$

$log0.1=tlog0.795$

$t=10$.

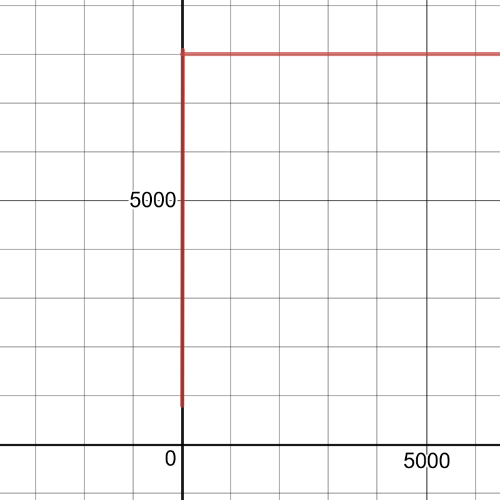
$P(t)=dfrac{8000}{1+9(0.719)^t}$

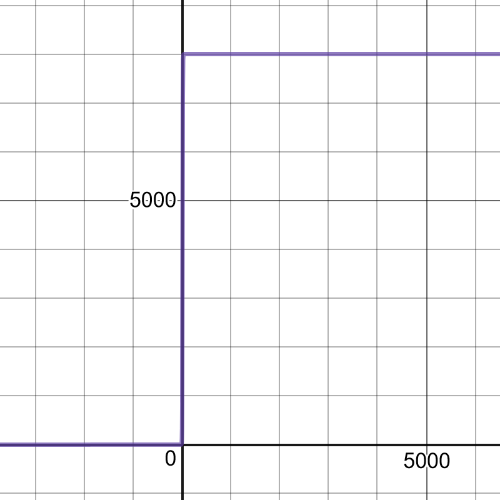
$P(4)=dfrac{8000}{1+9(0.719)^4}=2349$ trout four years after restocking.

#### (d)

The rate of change is $(2349-800)div 4$ or $387.25$ trout per year.

the carrying capacity of the lake; $8000$

#### (b)

Use $(0,800)$ and $(10, 6000)$.

$800=8000-a(b)^0$

$800=8000-a$

$-7200=-a$

$7200=a$

$6000=8000-7200(b)^{10}$

$-2000=-7200(b)^{10}$

$0.278=b^{10}$

$0.278^{dfrac{1}{10}}=b$

$0.88=b$

#### (c)

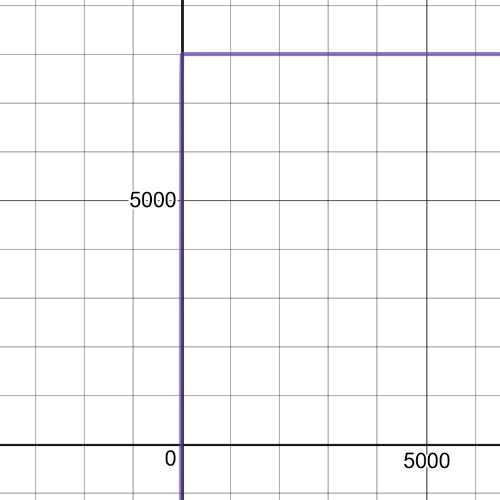
$P(4)=8000-7200(0.88)^4$

$=8000-4317.81$

$=3682$

#### (e)

The rate of change is $(3682-800)div 4$ or $720.5$ trout per year.

#### (f)

In the model in previous problem, the carrying capacity of the lake is divided by a number that gets smaller and smaller, while in this model, a number that gets smaller and smaller is subtracted from the carrying capacity of the lake.

$textbf{The equation is}$ $V(t)=0.85cos(dfrac{pi}{3}(t-1.5))$ and on the following picture there is its $textbf{graph.}$

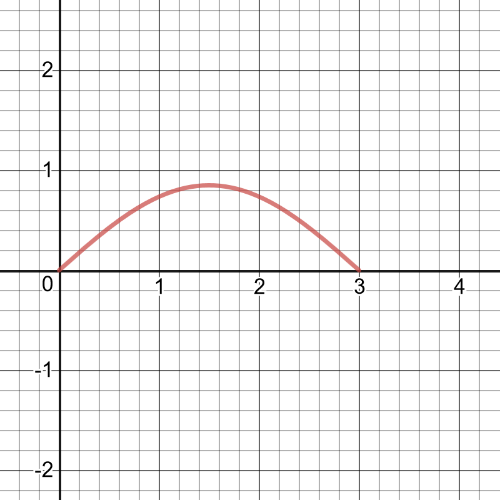
The scatter plot and the graph are very close to being the same, but $textbf{they are not exactly the same.}$
#### (b)

$V(6)=0.85cos(dfrac{pi}{3}(6-1.5))=0.85cos(dfrac{pi}{3}4.5)=0$ L/s
#### (d)

From the graph, the rate of change appears to be at its $textbf{smallest at}$ $t=1.5$s.
#### (e)

It is $textbf{the maximum}$ of the function.
#### (f)

From the graph, the rate of change appears to be at its $textbf{greatest at}$ $t=0$s.

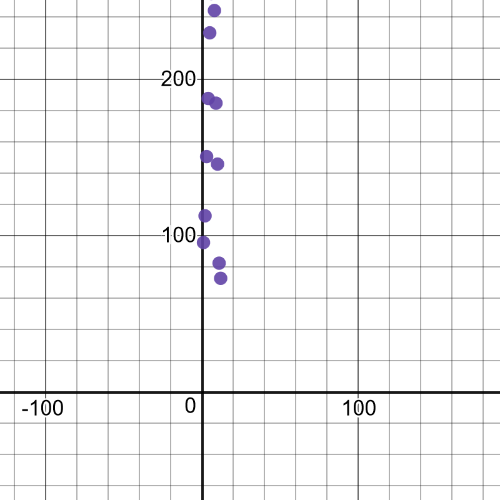
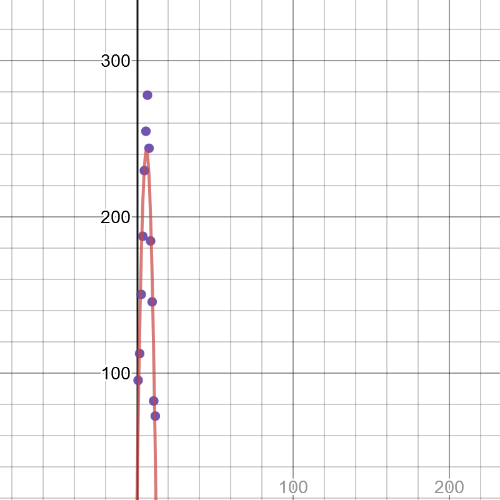
$$
S(t)=-6.07028t^2+77.1402x-2.75455
$$

#### (d)

From the model, $textbf{the maximum}$ will be at $t=7$ an $textbf{the minimum}$ will be at $t=1$.
#### (e)

$textbf{It does not fit it perfectly}$, because, actually, the minimum is not at $t=1$, but at $t=12$.

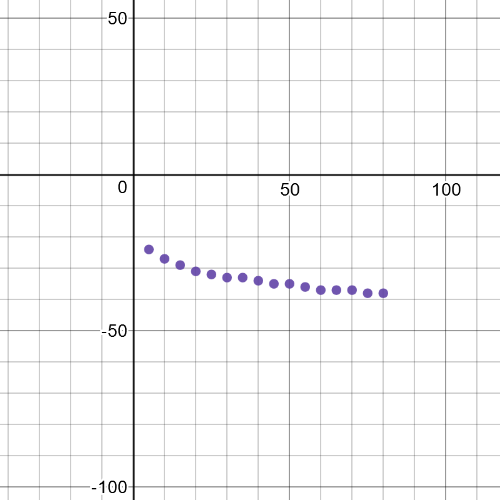
For example:

$C(s)=38+14(0.97)^t$

#### (c)

$C(0)=-38+14(0.97)^0=-38+14=-24^circ$C

$C(100)=-38+14(0.97)^100=-38+0.666=-37.3^circ$C

$C(200)=-38+14(0.97)^200=-38+0.032=-38^circ$C

These answers do not appear to be very resonable, because the wind chill for a wind speed od $0$ km/h should be $-20^circ$C, while the wind chills for wind speeds of $100$ km/h and $200$ km/h should be less than $-38^circ$C.The model only appears to be somewhat accurate for wind speeds of $10$ to $70$ km/h.

Answers will vary; for example, one polynomial model is $P(t)=1.4t^2+3230$, while an exponential model is $P(t)=3230(1.016)^t$. While neither model is perfect, it appears that the polynomial model fits the data better.

#### (b)

$P(155)=1.4(155)^2+3230=36865$

$P(155)=3230(1.016)^{155}=37820$

#### (c)

A case could be made for either model. The polynomial model appears to fit the data better, but population growth is usually exponential.

#### (d)

According to the polynomial model, in $2000$, the population was increasing at a rate of about $389000$ per year, while according to the exponential model, in $2000$, the population was increasing at a rate of about $465000$ per year.

$P(t)=3339.18(1132.25)^t$

#### (b)

$75=3339.18(1132.25)^t$

We can use graphing calculator to solve, and we get that solution is

$t=-31$.So, they were introduced around the year $1924$.
#### (c)

$textbf{rate of growth}$ = $(93100-650)div(35-0)=2641$ rabbits per year.
#### (d)

$$
P(65)=3339.18(1132.25)^65=10712509.96
$$

#### (a)

$V(t)=155.6 sinleft(120pi t+dfrac{pi}{2} right)$

#### (b)

$V(t)=155.6 cos(120 pi t)$

#### (c)

The cosine function was easier to determine.The cosine function is at its maximum when the argument is $0$, so no horizontal translation was necessary.

For example:

$textbf{a linear model is}$ $P(t)=-9t+400$

$textbf{a quadriatic model is}$ $P(t)=dfrac{23}{90}(t-30)^2+170$

$textbf{a exponential model is}$ $P(t)=400(0.972)^t$

The exponential model fits the data far better than the other two models.
#### (b)

$P(60)=-9cdot60+400=-540+400=-140$ kPa

$P(60)=dfrac{23}{90}(60-30)^2+170=dfrac{23}{60}30^2+170=dfrac{23}{90}900+170=230+170=400$ kPa

$P(60)=400(0.972)^60=73$ kPa
#### (c)

$textbf{The exponential model gives the most realistic answer}$, because it fits the data the best.Also, the pressure must be less than $170$ kPa, but it cannot be negative.

$$
T(n)=dfrac{1}{6}n^3+dfrac{1}{2}n^2+dfrac{1}{3}n
$$

#### (b)

$47850=dfrac{1}{6}n^3+dfrac{1}{2}n^2+dfrac{1}{3}n$

So, $n=64.975$.$textbf{So, it is not a tetrahedral number}$ because $n$ must be an integer.

Using a graphing calculator,the equation is $P(t)=30.75(1.008418)^t$

#### (b)

In $2000$, the growth rate of Canada was less than the growth rate of Ontario and Alberta.