The graphic property that least helps us in identifying a function can be $textbf{end behaviour}$, because it does not give us any more detailed information about the flow itself and the appearance of the function, and only on the basis of this feature we can hardly make any conclusions about the analytic form of function.

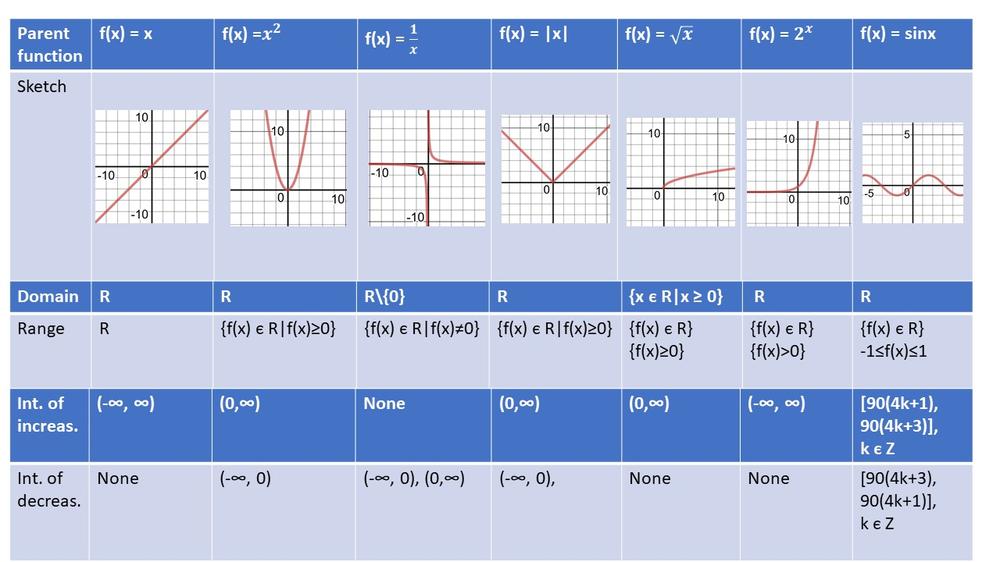
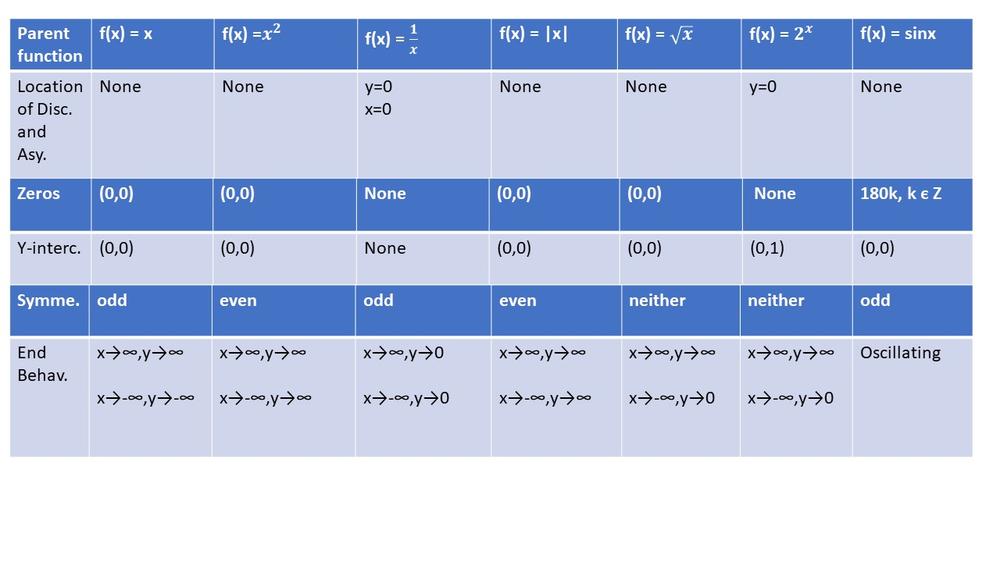
$$
textbf{End behaviour}
$$

The graphical characteristic that most helps us with the differentiation of the parent function is $textbf{domain and range}$, based on this we know from which area the function takes numbers and also in which values ​​it maps them, which greatly narrows the choice of the requested function.

$$
textbf{Domain and range}
$$

According to the previously discussed 7 functions, the requested function could be the transformation of function $2^{x}$, more precisely, the function $textcolor{#c34632}{y=2+2^{x}}$ . It satisfies the property that its domain is a set $R$, and the values ​​from the range are strictly greater than 2, and the property that if $xmapsto-infty$, we have that $ymapsto2$.

$$
textcolor{#c34632}{ y=2+2^{x}}
$$

#### (a)

Here,the characteristic that these two functions have in common is that the both of them are odd functions:

$f(-x)=-dfrac{1}{x}=-f(x)$

$g(-x)=-x=-g(x)$

The characteristic that distinguishes between them is different domain:

$D(f)=R/left{0 right}$

$$
D(f)=R
$$

#### (b)

Here,the characteristic that these two functions have in common is that the both of them have the same domain:

$D(f)=D(g)=R$

The characteristic that distinguishes between them is different range:

$R(f)=left[-1,1 right]$

$R(g)=R$

#### (c)

Here,the characteristic that these two functions have in common is that the both of them have the same domain:

$D(f)=D(g)=R$

The characteristic that distinguishes between them is that function $f$ is odd function and function $g$ is even function:

$f(-x)=-x=-f(x)$

$g(-x)=(-x)^2=x^2=g(x)$

#### (d)

Here,the characteristic that these two functions have in common is that the both of them have the same domain:

$D(f)=D(g)=R$

The characteristic that distinguishes between them is different range:

$R(f)=left{yin R |y>0right}$

$R(g)=left{yin R |ygeq0right}$

#### (a)

$f(-x)=(-x)^2-4=x^2-4=f(x)$

$-f(-x)=-(x^2-4)=-f(x)$

So, the conclusion is that this function is $text{textcolor{#c34632}{even}}$ function.

The function is even if its algebraic form satisfies the relation $textbf{f(-x)=f(x)}$

#### (b)

$f(-x)=sin(-x)+(-x)=-sin{x}-x=\=-(sin{x}+x)=-f(x)$

$-f(-x)=-(-(sin{x}+x))=sin{x}+x=f(x)$

So,the conclusion is that $f$ is $text{textcolor{#c34632}{odd}}$ function.

The function is odd if its algebraic form satisfies the relation $textbf{f(-x)=-f(x)}$

#### (c)

$$
f(-x)=-dfrac{1}{x}-(-x)=-dfrac{1}{x}+x=-(dfrac{1}{x}-x)=-f(x)
$$

$-f(-x)=-(-(dfrac{1}{x}-x))=dfrac{1}{x}-x=f(x)$

So, the conclusion is that $f$ is $text{textcolor{#c34632}{odd}}$ function.

#### (d)

$f(-x)=2(-x)^2+(-x)=2x^2-x$

$Rightarrow(f(-x)ne f(x) wedge f(-x)ne-f(x))$

$-f(-x)=-(2x^2-x)=-2x^2+x$

So,the conclusion is that function $f$ is $text{textcolor{#c34632}{neither}}$ function

The function is neither if its algebraic form does not satisfiy the relations $textbf{f(-x)=f(x)}$ or $textbf{f(-x)=-f(x)}$

#### (e)

$f(-x)=2(-x)^2-(-x)=2x^2+x$

$Rightarrow(f(-x)ne f(x) wedge f(-x)ne-f(x))$

$-f(-x)=-(2x^2+x)=-2x^2-x$

So,the conclusionis that $f$ is $text{textcolor{#c34632}{neither}}$ function.

#### (f)

$f(-x)=|2(-x)+3|=|-2x+3|=|-(2x-3)|=\=|2x-3|$

$-f(-x)=-|2x-3|$

So, the conclusion is that $f$ is $text{textcolor{#c34632}{neither}}$ function.

#### (a)

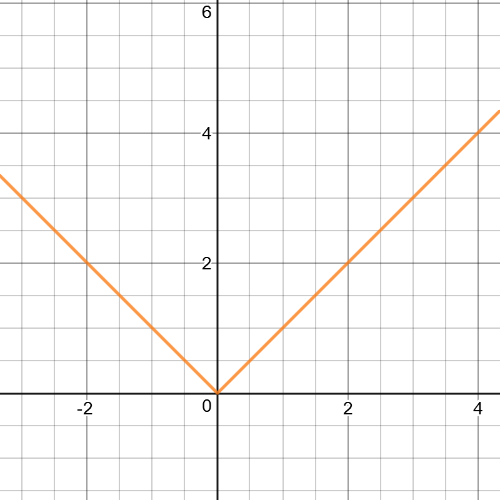
$|x|$,because it is a measure of distance from a number.

#### (b)

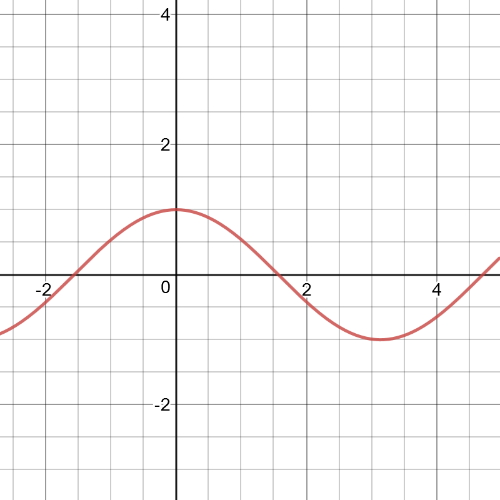
$sin(x)$,because the heights are periodic

#### (c)

$2^x$, because population tends to increase exponentially

#### (d)

$x$,because there is $1 on the first day, $2 on the second, $3 on the third, etc.

#### (a)

It might be the following function:

$f(x)=dfrac{x}{1-x}$

For real, the domain of this function is real numbers without 1, it is because of the denominator, $x-1$. In order for a function to be defined, the denominator must be different from zero, and in this case it is different from zero for $xne1$.

Also, the second condition is satisfied:

$f(0)=dfrac{0}{1-0}=0$

#### (b)

It might be the following function:

$f(x)=sin{x}$

As we could see from some of the previous examples, the graph of this function infinitely many times cuts x axis, so it has infinitely many zeros.

#### (c)

It might be the following function:

$f(x)=cos{x}$

Its graph is even, because this function is even function, and also, its graph is smooth,it has not sharps.

#### (d)

It might be the following function:

$y=x$

Values ​​that take $x$, the same values ​​are taken by $y$.

#### (a)

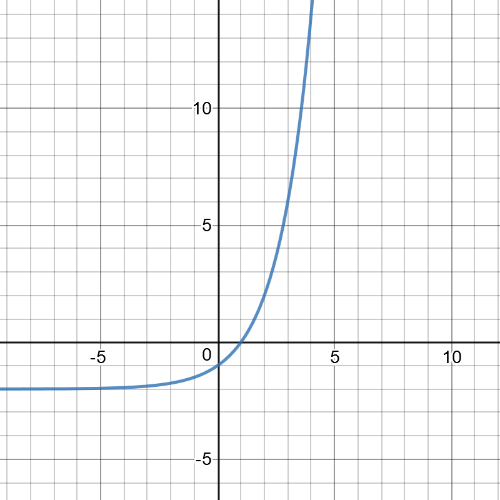
According to all the data given to us about the function, we conclude that the function satisfying all of them can be $f(x)=2^x-2$, which is proved by the following graph of this function:

#### (b)

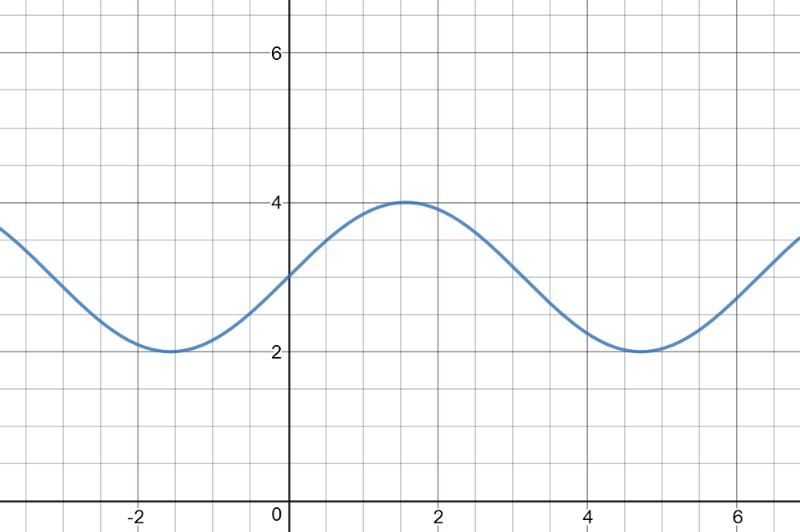
According to all the data given to us about the function, we conclude that the function satisfying all of them can be $f(x)=sin{x}+3$, which is proved by the following graph of this function:

#### (d)

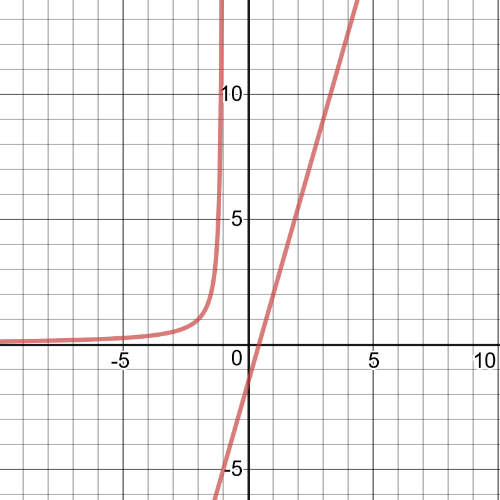
According to all the data given to us about the function, we conclude that the function satisfying all of them can be $f(x)=dfrac{1}{x-5}$, which is proved by the following graph of this function:

#### (a)

According to all the data given to us about the function, we conclude that a function satisfying all of them, and besides being a quadratic function, can be $f(x)=x^2+4$, which is also proved by the following graph of this function:

#### (b)

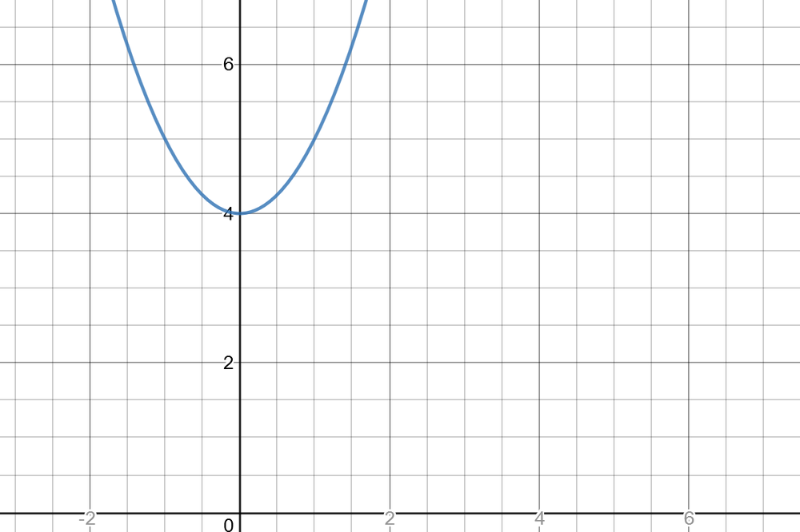
There $textbf{is not}$ only one quadratic function, that has the
characteristics given in part a). Characteristics remain the same with each change of coefficient with positive sign in front of $x^2$.

#### (d)

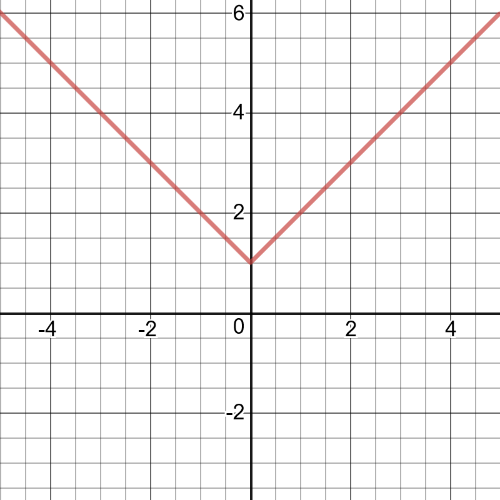
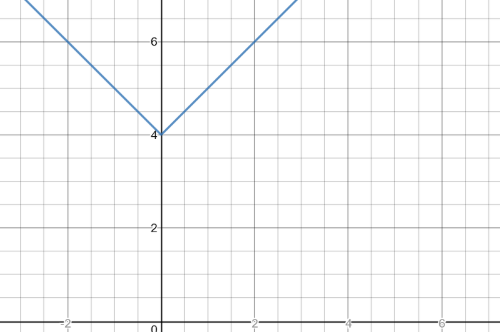
If $f$ is an absolute value function, its transformation satisfying all the conditions given in a) would be a function $f(x)=left|x right|+4$ .Analogously, as a pre-emptive function, the characteristics will not change if we change coefficients with a positive sign in front of $left|x right|$. The appearance of the absolute function that fits all of the above is found in the following graphic:

These two functions have all the same characteristics that are mentioned in the preceding examples and the tasks except one, which is that the function $g(x)=left|x right|$ is sharp at the point 0, and the function $f(x)=x^2$ is rounded. More specifically, for the graph of the function f it is said to have a parabola shape. This proves the graphics of these functions that follow. The yellow is graph of the function $g(x)$ and the blue one is graph of the function $f(x)$:

It is useful to name these functions because they belong to one of the more $textbf{elementary functions}$, and many other functions are based on them. All these other functions can be obtained with $textbf{different transformations}$ or we can get them by looking for the $textbf{inverse functions}$ of these elementary functions so far.

$textbf{Domain and range}$ of this function is an entire set of $R$,just like function $g(x)=x$.Also, this function is an $textbf{odd function}$, like previous function, $g(x)=x$ and like $h(x)=sin{x}$.The function $f(x)=x^3$ is $textbf{increasing}$, like, for example, function $d(x)=2^x$.All these properties can be seen from the graph of the considered function $f(x)=x^3$, which follows:

This function does not need to be considered as a parent function because it has many common properties with function $f(x)=sin{x}$ , such as, for example, periodicity, the same domains and range … And also it can be obtained by transformation of the bass of the considered function $f(x)=sin{x}$.There Is a graph of function $h(x)=cos{x}$, where we can see just discussed similarities with parent function $f(x)=sin{x}$.

$textbf{The graph can have $0$, $1$ or $2$ zeros}$.

$0$ zeros:

$1$ zero:

$2$ zeros:

$0$, $1$ or $2$ zeros