$$
text{color{#4257b2}Draw a graph of a polynomial function that has all the following characteristics.
\ \
** $f(2)=10, f(-3)=0, f(4)=0$
\ \
** the $(y)$ intercept is zero
\ \
** $f(x)>0$ when $x<-3$ and $0<x<4$
\ \
** $f(x)4$ and $-3<x<0$}
$$

The graph has a three zeros are, $(x=0, x=-3, x=4)$ so this graph is represented to the cubic function with two turning points as follows:

$$
f(x)=-x(x+3)(x-4)
$$

$$
text{color{Brown} See the graph
\ \
$f(x)=-x(x+3)(x-4)$}
$$

Describe the end behavior for the function.

This function has an odd degree $text{color{#4257b2}cubic function}$ and the leading coefficient is negative because its downward function, so that the end behavior should be as follows:

The function extend from the second quadrant to the forth quadrant.

$$
xrightarrow-infty, yrightarrowinfty text{and} xrightarrowinfty, yrightarrow-infty
$$

$$
text{color{Brown}$xrightarrow-infty, yrightarrowinfty text{and} xrightarrowinfty, yrightarrow-infty$}
$$

State the possible degree, the sign of leading coefficient and the number of the turning point for each graph in the textbook.

$$
color{#4257b2}text{(a) First graph}
$$

Degree $n=3$

Sign of leading coefficient is positive.

The number of turning point may have $[2, 0]$ turning point.

$$
color{#4257b2}text{(b) Second graph}
$$

Degree $n=4$

Sign of leading coefficient is positive.

The number of turning point may have $[3, 1]$ turning point.

$$
text{color{Brown}(a) Degree $n=3$
\ \
Sign of leading coefficient is positive.
\ \
Number of turning point may have $[2, 0]$ turning point.
\ \
(b) Degree $n=4$
\ \
Sign of leading coefficient is positive.
\ \
Number of turning point may have $[3, 1]$ turning point.}
$$

Write the cubic function for the following given zeros.

$$
color{#4257b2}text{(a)} -3, 6, 4
$$

Standard form of cubic function is $f(x)=a(x-x_{1})(x-x_{2})(x-x_{3})$

$$
f(x)=a (x+3)(x-6)(x-4)
$$

$$
color{#4257b2}text{(b)} -7, 2, 3
$$

Standard form of cubic function is $f(x)=a(x-x_{1})(x-x_{2})(x-x_{3})$

$$
f(x)=a (x+7)(x-2)(x-3)
$$

$$
color{#4257b2}text{(c)} 5, -1, -2
$$

Standard form of cubic function is $f(x)=a(x-x_{1})(x-x_{2})(x-x_{3})$

$$
f(x)=a (x-5)(x+1)(x+2)
$$

$$
color{#4257b2}text{(d)} 9, -5, -4
$$

Standard form of cubic function is $f(x)=a(x-x_{1})(x-x_{2})(x-x_{3})$

$$
f(x)=a (x-9)(x+5)(x+4)
$$

$$
text{color{Brown}(a) $f(x)=a (x+3)(x-6)(x-4)$
\ \
(b) $f(x)=a (x+7)(x-2)(x-3)$
\ \
(c) $f(x)=a (x-5)(x+1)(x+2)$
\ \
(d) $f(x)=a (x-9)(x+5)(x+4)$}
$$

Write the quartic function for the following given zeros.

$$
color{#4257b2}text{(a)} -6, 2, 5, 8
$$

Standard form of cubic function is $f(x)=a(x-x_{1})(x-x_{2})(x-x_{3})(x-x_{4})$

$$
f(x)=a (x+6)(x-2)(x-5)(x-8)
$$

$$
color{#4257b2}text{(b)} 0, -1, 9, 10
$$

Standard form of cubic function is $f(x)=a x (x-x_{1})(x-x_{2})(x-x_{3})$

$$
f(x)=a x (x+1)(x-9)(x-10)
$$

$$
color{#4257b2}text{(c)} 4, -8, 1, 2
$$

Standard form of cubic function is $f(x)=a(x-x_{1})(x-x_{2})(x-x_{3})(x-x_{4})$

$$
f(x)=a (x-4)(x+8)(x-1)(x-2)
$$

$$
color{#4257b2}text{(d)} -3, 3, -6, 6
$$

Standard form of cubic function is $f(x)=a(x-x_{1})(x-x_{2})(x-x_{3})(x-x_{4})$

$$
f(x)=a (x+3)(x-3)(x+6)(x-6)
$$

$$
f(x)=a (x^2-3^2)(x^2-6^2)
$$

$$
text{color{Brown}(a) $f(x)=a (x+6)(x-2)(x-5)(x-8)$
\ \
(b) $f(x)=a x (x+1)(x-9)(x-10)$
\ \
(c) $f(x)=a (x-4)(x+8)(x-1)(x-2)$
\ \
(d) $f(x)=a (x^2-3^2)(x^2-6^2)$}
$$

Sketch the graph:

$$
f(x)=(x-3)(x+2)(x+5)
$$

Use distributive property as follows:

$$
f(x)=(x^2+2x-3x-6)(x+5)
$$

$$
f(x)=(x^2-x-6)(x+5)
$$

$$
f(x)=x^3+5x^2-x^2-5x-6x-30
$$

Rearrange the tiles to group like terms as follows:

$$
f(x)=x^3+(5x^2-x^2)+(-5x-6x)-30
$$

$$
f(x)=x^3+4x^2-11x-30
$$

$$
text{color{Brown}$f(x)=x^3+4x^2-11x-30$}
$$

Write the function that has zeros are $[pm 1, -2]$ and $y$ intercept is $-6$ then sketch the function.

$$
y=(x+x_{1})(x+x_{2})(x+x_{3})
$$

$$
y=(x+1)(x-1)(x-2)-8
$$

$$
(x^2-1)(x-2)-8
$$

$$
x^3-2x^2-x+2-8
$$

$$
x^3-2x^2-x-6
$$

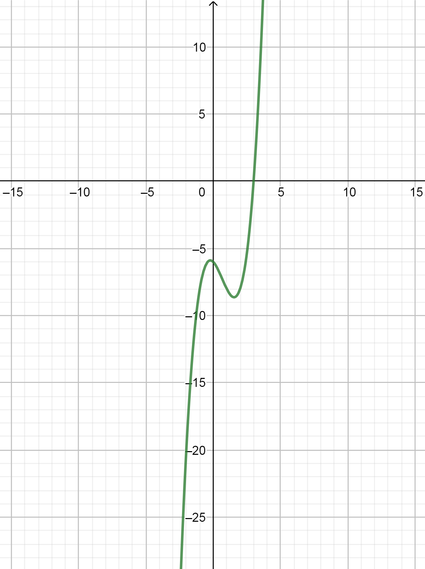
$$
text{color{Brown}$x^3-2x^2-x-6$}
$$

Describe the transformed function for the original equation is $y=x^2$ for the following terms:

$$
color{#4257b2}text{(a)} y=-2(x-1)^2+23
$$

$$
a=-2 k=1 d=1 c=23
$$

$$
color{#4257b2}text{(b)} y=left[dfrac{12}{13}(x+9)right]^2-14
$$

$$
k=dfrac{12}{13} d=-9 c=-14
$$

$$
color{#4257b2}text{(c)} y=x^2-8x+16
$$

$$
=(x-4)^2
$$

$$
d=4
$$

$$
color{#4257b2}text{(d)} y=left(x+dfrac{3}{7}right) left(x+dfrac{3}{7}right)
$$

$$
=left(x+dfrac{3}{7}right)^2
$$

$$
d=-dfrac{3}{7}
$$

$$
color{#4257b2}text{(e)} y=40[-7(x-10)]^2+9
$$

$$
a=40 k=-7 d=10 c=9
$$

$$
text{color{Brown}(a) $a=-2 k=1 d=1 c=23$
\ \
(b) $k=dfrac{12}{13} d=-9 c=-14$
\ \
(c) $d=4$
\ \
(d) $d=-dfrac{3}{7}$
\ \
(e) $a=40 k=-7 d=10 c=9$}
$$

Note that with respect to the parent function $y=x^n$ in $y=a(k(x-d)^n)+c$

* value of $a$ represents vertical stretch for values of $|a|>1$
or compression for values of $|a|1$
or compression for values of $|k|<1$ and possibly a horizontal reflection in $y$ axis for negative values of $k$;

* value of $d$ represents horizontal translation to the left for a negative sign of $d$ and to the right for positive values.

* value of $c$ represents vertical translation downward for a negative sign of $c$ and upward for positive values.

Then each point on the graph of the parent function $(x,y)$ changes to the point
$$left( dfrac{x}{k}+d,ay+cright).tag{1}$$

*(a)* From the given transformations following the transformation properties given in the first step, we can conclude that $a=25$, $k=frac{5}{6}$ and $d=3$. The parent function after the transformations we can rewrite as
$$y=25left(dfrac{5}{6}(x-3) right)^3.$$

To obtain three points on this function, we can choose any point on the graph of the parent function $(x,y)$, and use Eq. $(1)$ to obtain the coordinates of that point on the transformed function. Choosing the $x$ coordinates of the points on the parent function $y=x^3$ we can obtain $y$ coordinates using the equation of the function. If we choose $x$ coordinates to be $x_1=0$, $x_2=-1$ and $x_3=1$, using the parent functino $y=x^3$, we obtain
$$
begin{align*}
y_1&=0^3=0\
y_2&=(-1)^3=-1\
y_3&=1^3=1.
end{align*}
$$
Now using these values and Eq. $(1)$ we obtain three points on the graph of the function obtained after applied transformations.
$$
begin{align*}
left( dfrac{0}{frac{5}{6}}+3,25cdot0+0right)&=(3,0)\
left( dfrac{-1}{frac{5}{6}}+3,25cdot(-1)+0right)&=(1.8,-25)\
left( dfrac{1}{frac{5}{6}}+3,25cdot1+0right)&=(4.2,25).\
end{align*}
$$

*(b)* From the given transformations following the transformation properties given in the first step, we can conclude that $a=1$, $k=-7$, $d=0$ and $c=-19$. The parent function after the transformations we can rewrite as
$$y=left(-7x right)^3-19=-343x^3-19.$$

To obtain three points on this function, we can choose any point on the graph of the parent function $(x,y)$, and use Eq. $(1)$ to obtain the coordinates of that point on the transformed function. Choosing the $x$ coordinates of the points on the parent function $y=x^3$ we can obtain $y$ coordinates using the equation of the function. If we choose $x$ coordinates to be $x_1=0$, $x_2=-1$ and $x_3=1$, using the parent functino $y=x^3$, we obtain
$$
begin{align*}
y_1&=0^3=0\
y_2&=(-1)^3=-1\
y_3&=1^3=1.
end{align*}
$$
Now using these values and Eq. $(1)$ we obtain three points on the graph of the function obtained after applied transformations.
$$
begin{align*}
left( dfrac{0}{-7}+0,-1cdot0-19right)&=(0,-19)\
left( dfrac{-1}{-7}+0,-1cdot(-1)-19right)&=left( dfrac{1}{7},-18right)\
left( dfrac{1}{-7}+0,-1cdot1-19right)&=left( -dfrac{1}{7},-20right).\
end{align*}
$$

*(c)* From the given transformations following the transformation properties given in the first step, we can conclude that $a=-frac{6}{11}$, $k=1$, $d=-5$ and $c=16$. The parent function after the transformations we can rewrite as
$$y=-frac{6}{11}left(x+5 right)^3+16.$$

To obtain three points on this function, we can choose any point on the graph of the parent function $(x,y)$, and use Eq. $(1)$ to obtain the coordinates of that point on the transformed function. Choosing the $x$ coordinates of the points on the parent function $y=x^3$ we can obtain $y$ coordinates using the equation of the function. If we choose $x$ coordinates to be $x_1=0$, $x_2=-1$ and $x_3=1$, using the parent functino $y=x^3$, we obtain
$$
begin{align*}
y_1&=0^3=0\
y_2&=(-1)^3=-1\
y_3&=1^3=1.
end{align*}
$$
Now using these values and Eq. $(1)$ we obtain three points on the graph of the function obtained after applied transformations.
$$
begin{align*}
left( dfrac{0}{1}-5,-frac{6}{11}cdot0+16right)&=(-5,16)\
left( dfrac{-1}{1}-5,-frac{6}{11}cdot(-1)+16right)&=left(-6, dfrac{182}{11}right)\
left( dfrac{1}{1}-5,-frac{6}{11}cdot1+16right)&=left(-4, dfrac{170}{11}right)\
end{align*}
$$

*(d)* From the given transformations following the transformation properties given in the first step, we can conclude that $a=100$, $k=2$, and $c=14$. The parent function after the transformations we can rewrite as
$$y=100left(2x right)^3+14=800x^3+14.$$

To obtain three points on this function, we can choose any point on the graph of the parent function $(x,y)$, and use Eq. $(1)$ to obtain the coordinates of that point on the transformed function. Choosing the $x$ coordinates of the points on the parent function $y=x^3$ we can obtain $y$ coordinates using the equation of the function. If we choose $x$ coordinates to be $x_1=0$, $x_2=-1$ and $x_3=1$, using the parent function $y=x^3$, we obtain
$$
begin{align*}
y_1&=0^3=0\
y_2&=(-1)^3=-1\
y_3&=1^3=1.
end{align*}
$$
Now using these values and Eq. $(1)$ we obtain three points on the graph of the function obtained after applied transformations.
$$
begin{align*}
left( dfrac{0}{2}-0,100cdot0+14right)&=(0,14)\
left( dfrac{-1}{2}-0,100cdot(-1)+14right)&=left( dfrac{-1}{2},-86right)\
left( dfrac{1}{2}-0,100cdot1+14right)&=left( dfrac{1}{2},114right).\
end{align*}
$$

*(e)* From the given transformations following the transformation properties given in the first step, we can conclude that $a=-1$, $k=1$, and $c=-45$. The parent function after the transformations we can rewrite as
$$y=-x^3-45.$$

To obtain three points on this function, we can choose any point on the graph of the parent function $(x,y)$, and use Eq. $(1)$ to obtain the coordinates of that point on the transformed function. Choosing the $x$ coordinates of the points on the parent function $y=x^3$ we can obtain $y$ coordinates using the equation of the function. If we choose $x$ coordinates to be $x_1=0$, $x_2=-1$ and $x_3=1$, using the parent function $y=x^3$, we obtain
$$
begin{align*}
y_1&=0^3=0\
y_2&=(-1)^3=-1\
y_3&=1^3=1.
end{align*}
$$
Now using these values and Eq. $(1)$ we obtain three points on the graph of the function obtained after applied transformations.
$$
begin{align*}
left( dfrac{0}{1}-0,-1cdot0-45right)&=(0,-45)\
left( dfrac{-1}{1}-0,-1cdot(-1)-45right)&=left( -1,-44right)\
left( dfrac{1}{1}-0,-1cdot1-45right)&=left(1,-46right).\
end{align*}
$$

*(f)* From the given transformations following the transformation properties given in the first step, we can conclude that $a=1$, $k=-frac{7}{10}$, $d=12$ and $c=6$. The parent function after the transformations we can rewrite as
$$y=left(-dfrac{7}{10}(x-12) right)^3+6.$$

To obtain three points on this function, we can choose any point on the graph of the parent function $(x,y)$, and use Eq. $(1)$ to obtain the coordinates of that point on the transformed function. Choosing the $x$ coordinates of the points on the parent function $y=x^3$ we can obtain $y$ coordinates using the equation of the function. If we choose $x$ coordinates to be $x_1=0$, $x_2=-1$ and $x_3=1$, using the parent function $y=x^3$, we obtain
$$
begin{align*}
y_1&=0^3=0\
y_2&=(-1)^3=-1\
y_3&=1^3=1.
end{align*}
$$
Now using these values and Eq. $(1)$ we obtain three points on the graph of the function obtained after applied transformations.
$$
begin{align*}
left( dfrac{0}{-frac{7}{10}}+12,1cdot0+6right)&=(12,6)\
left( dfrac{-1}{-frac{7}{10}}+12,1cdot(-1)+6right)&=left( dfrac{94}{
7},5right)\
left( dfrac{1}{-frac{7}{10}}+12,1cdot1+6right)&=left(dfrac{74}{7},7right).\
end{align*}
$$

Divide the following functions:

$$
color{#4257b2}text{(a)} 2x^3+5x^2+3x-4 text{by} (x+5)
$$

Divided the first term for the two question s as follows:

$$
=dfrac{2x^3}{x}=2x^2
$$

Then multiply it $(2x^2)$with $(x+5)$ as follows:

$$
(2x^2)(x+5)=2x^3+10x^2 text{reverse}=-2x^3-10x^2
$$

Subtract the reverse result with $(2x^3+5x^2+3x-4)$ as follows:

$$
cancel 2x^3+5x^2+3x-4cancel-2x^3-10x^2 -5x^2+3x-4
$$

Divided the first term for the two question s as follows:

$$
=dfrac{-5x^2}{x}=-5x
$$

Then multiply it $(-5x)$with $(x+5)$ as follows:

$$
=-5x^2-25x text{reverse}=5x^2+25x
$$

Subtract the reverse result with $(-5x^2+3x-4)$ as follows:

$$
cancel-5x^2+3x-4+cancel5x^2+25x=0 28x-4
$$

Divided the first term for the two question s as follows:

$$
=dfrac{28x}{x}=28
$$

Then multiply it $(28)$with $(x+5)$ as follows:

$$
=28x+140 text{reverse}=-28x+140
$$

Subtract the reverse result with $(28x-4)$ as follows:

$$
cancel28x-4cancel-28x+140=0 =136
$$

$$
(2x^3+5x^2+3x-4)=(x+5)(2x^2-5x+28)+136
$$

$$
color{#4257b2}text{(b)} x^4+4x^3-3x^2-6x-7 text{by} (x^2-8)
$$

Divided the first term for the two question s as follows:

$$
=dfrac{x^4}{x^2}=x^2
$$

Then multiply it $(x^2)$ with $(x^2-8)$ as follows:

$$
(x^2)(x^2-8)=x^4-8x^2 text{reverse}=-x^4+8x^2
$$

Subtract the reverse result with $(x^4+4x^3-3x^2-6x-7)$ as follows:

$$
cancel x^4+4x^3-3x^2-6x-7cancel-x^4+8x^2 4x^3+5x^2-6x-7
$$

Divided the first term for the two question s as follows:

$$
=dfrac{4x^3}{x^2}=4x
$$

Then multiply it $(4x)$ with $(x^2-8)$ as follows:

$$
=4x^3-32x text{reverse}=-4x^3+32x
$$

Subtract the reverse result with $(4x^3+5x^2-6x-7)$ as follows:

$$
cancel4x^3+5x^2-6x-7cancel-4x^3+32x=0 5x^2+26x-7
$$

Divided the first term for the two question s as follows:

$$
=dfrac{5x^2}{x^2}=5
$$

Then multiply it $(5)$ with $(x^2-8)$ as follows:

$$
=5x^2-40 text{reverse}=-5x^2+40
$$

Subtract the reverse result with $(5x^2+26x-7)$ as follows:

$$
cancel5x^2+26x-7cancel-5x^2+40=0 =26x+33
$$

$$
(x^4+4x^3-3x^2-6x-7)=(x^2-8)(x^2+4x+5)+26x+33
$$

$$
color{#4257b2}text{(c)} 2x^4-2x^2+3x-16 text{by} (x^3+3x^2+3x-3)
$$

Divided the first term for the two question s as follows:

$$
=dfrac{2x^4}{x^3}=2x
$$

Then multiply it $(2x)$with $(x^3+3x^2+3x-3)$ as follows:

$$
=2x^4+6x^3+6x^2-6x text{reverse}=-2x^4-6x^3-6x^2+6x
$$

Subtract the reverse result with $(2x^4-2x^2+3x-16)$ as follows:

$$
cancel 2x^4-2x^2+3x-16cancel-2x^4-6x^3-6x^2+6x -6x^3-8x^2+9x-16
$$

Divided the first term for the two question s as follows:

$$
=dfrac{-6x^3}{x^3}=-6
$$

Then multiply it $(-6)$with $(x^3+3x^2+3x-3)$ as follows:

$$
=-6x^3-18x^2-18x+18 text{reverse}=6x^3+18x^2+18x-18
$$

Subtract the reverse result with $(-6x^3-8x^2+9x-16)$ as follows:

$$
cancel-6x^3-8x^2+9x-16cancel6x^3+18x^2+18x-18=0 10x^2+27x-34
$$

$$
(2x^4-2x^2+3x-16)=(x^2-8)(x^3+3x^2+3x-3)+10x^2+27x-34
$$

$$
color{#4257b2}text{(d)} x^5-8x^3-7x-6 text{by} (x^4+4x^3+4x^2-x-3)
$$

Divided the first term for the two question s as follows:

$$
=dfrac{x^5}{x^4}=x
$$

Then multiply it $(x)$with $(x^4+4x^3+4x^2-x-3)$ as follows:

$$
=x^5+4x^4+4x^3-x^2-3x text{reverse}=-x^5-4x^4-4x^3+x^2+3x
$$

Subtract the reverse result with $(x^5-8x^3-7x-6)$ as follows:

$$
cancel x^5-8x^3-7x-6cancel-x^5-4x^4-4x^3+x^2+3x -4x^4-12x^3+x^2-4x-6
$$

Divided the first term for the two question s as follows:

$$
=dfrac{-4x^4}{x^4}=-4
$$

Then multiply it $(-4)$with $(x^4+4x^3+4x^2-x-3)$ as follows:

$$
=-4x^4-16x^3-16x^2+4x+12 text{reverse}=4x^4+16x^3+16x^2-4x-12
$$

Subtract the reverse result with $(-4x^4-12x^3+x^2-4x-6)$ as follows:

$$
cancel-4x^4-12x^3+x^2-4x-6cancel4x^4+16x^3+16x^2-4x-12=0
$$

$$
4x^3+17x^2-8x-18
$$

$$
(x^5-8x^3-7x-6)=(x-4)(x^4+4x^3+4x^2-x-3)+4x^3+17x^2-8x-18
$$

$$
text{color{Brown}(a) $(x+5)(2x^2-5x+28)+136$
\ \
(b) $(x^2-8)(x^2+4x+5)+26x+33$
\ \
(c) $(x^2-8)(x^3+3x^2+3x-3)+10x^2+27x-34$
\ \
(d) $(x-4)(x^4+4x^3+4x^2-x-3)+4x^3+17x^2-8x-18$}
$$

Divide the following terms by $(x+2)$

$$
color{#4257b2}text{(a)} 2x^3+5x^2-x-5
$$

$(x+2)$ is the factor, so use $(x=-2)$ to divide the equation as follows:

$$
begin{align*}
-2| &=2 5 -1 -5
\ \
&= -4 -2 6
\ \
&=2 1 -3 1
end{align*}
$$

$$
2x^3+5x^2-x-5=(x+2)(2x^2+x-3)+1
$$

$$
color{#4257b2}text{(b)} 3x^3+13x^2+17x+3
$$

$(x+2)$ is the factor, so use $(x=-2)$ to divide the equation as follows:

$$
begin{align*}
-2| &=3 13 17 3
\ \
&= -6 -14 -6
\ \
&=3 7 3 -3
end{align*}
$$

$$
3x^3+13x^2+17x+3=(x+2)(3x^2+7x+3)-3
$$

$$
color{#4257b2}text{(c)} 2x^4+5x^3-16x^2-45x-18
$$

$(x+2)$ is the factor, so use $(x=-2)$ to divide the equation as follows:

$$
begin{align*}
-2| &=2 5 -16 -45 -18
\ \
&= -4 -2 36 18
\ \
&=2 1 -18 -9 0
end{align*}
$$

$$
2x^4+5x^3-16x^2-45x-18=(x+2)(2x^3+x^2-18x-9)+1
$$

$$
color{#4257b2}text{(d)} 2x^3+4x^2-5x-4
$$

$(x+2)$ is the factor, so use $(x=-2)$ to divide the equation as follows:

$$
begin{align*}
-2| &=2 4 -5 -4
\ \
&= -4 0 10
\ \
&=2 0 -5 6
end{align*}
$$

$$
2x^3+4x^2-5x-4=(x+2)(2x^2-5)+6
$$

$$
text{color{Brown} (a) $(x+2)(3x^2+7x+3)-3$
\ \
(b) $(x+2)(2x^2+x-3)+1$
\ \
(c) $(x+2)(2x^3+x^2-18x-9)+1$
\ \
(d) $(x+2)(2x^2-5)+6$}
$$

Determine the dividend for the following terms.

$$
color{#4257b2}text{(a)} (x-9)(2x^2+11x-8)+3
$$

Use distributive property as follows:

$$
x(2x^2+11x-8)-9(2x^2+11x-8)+3
$$

$$
2x^3+11x^2-8x-18x^2-99x+72+3
$$

$$
color{Brown}2x^3-7x^2-107x+75
$$

$$
color{#4257b2}text{(b)} (4x+3)(x^3-2x+7)-4
$$

Use distributive property as follows:

$$
4x(x^3-2x+7)+3(x^3-2x+7)-4
$$

$$
4x^4-8x^2+28x+3x^3-6x+21-4
$$

$$
color{Brown}4x^4+3x^3-8x^2+22x+17
$$

$$
color{#4257b2}text{(c)} (3x-4)(x^3+6x^2-6x-7)+5
$$

Use distributive property as follows:

$$
3x(x^3+6x^2-6x-7)-4(x^3+6x^2-6x-7)+5
$$

$$
3x^4+18x^3-18x^2-21x-4x^3-24x^2+24x+28+5
$$

$$
color{Brown}3x^4+14x^3-42x^2+3x+33
$$

$$
color{#4257b2}text{(d)} (3x^2+x-5)(x^4-4x^3+9x-3)+2x-1
$$

Use distributive property as follows:

$$
3x^2(x^4-4x^3+9x-3)+x(x^4-4x^3+9x-3)-5(x^4-4x^3+9x-3)+2x-1
$$

$$
3x^6-12x^5+27x^3-9x^2+x^5-4x^4+9x^2-3x-5x^4+20x^3-45x+15+2x-1
$$

$$
color{Brown}3x^6-11x^5-9x^4+47x^3-46x+14
$$

$$
text{color{Brown}(a) $color{Brown}2x^3-7x^2-107x+75$
\ \
(b) $color{Brown}4x^4+3x^3-8x^2+22x+17$
\ \
(c) $color{Brown}3x^4+14x^3-42x^2+3x+33$
\ \
(d) $color{Brown}3x^6-11x^5-9x^4+47x^3-46x+14$}
$$

Determine the reminder if the equation $(f(x)=x^3+2x^2-6x+1)$ divided by $(x+2)$

$f(x)=$ divisor$cdot$quotient + reminder

$f(x)=(x+2)cdot$quotient + reminder

$f(-2)=(-2+2)cdot$quotient + reminder

$f(-2)=$ reminder

$$
f(-2)=(-2)^3+2(-2)^2-6(-2)+1
$$

$$
f(-2)=-8+8+12+1
$$

$$
f(-2)=13
$$

The reminder is equal $(13)$

$$
text{color{Brown} The reminder is equal $(13)$}
$$

Factor the following expressions

$$
color{#4257b2}text{(a)} x^3-5x^2-22x-16
$$

Use the factor theorem to factor the cubic function as follows:

$$
f(1)=1-5-22-16 f(1)=-42
$$

$$
f(-1)=-1-5+22-16 f(-1)=0
$$

$(x+1)$ is the factor, so use $(x=-1)$ to divide the equation as follows:

$$
begin{align*}
-1| &=1 -5 -22 -16
\ \
&= -1 6 16
\ \
&=1 -6 -16 0
end{align*}
$$

$$
x^3-5x^2-22x-16=(x+1)(x^2-6x-16)
$$

$$
x^3-5x^2-22x-16=(x+1)(x+2)(x-8)
$$

$$
color{#4257b2}text{(b)} 2x^3+x^2-27x-36
$$

Use the factor theorem to factor the cubic function as follows:

$$
f(1)=2+1-27-36 f(1)=-60
$$

$$
f(-1.5)=-6.75+2.25+40.5-36 f(-1.5)=0
$$

$(x+1.5)$ is the factor, so use $(x=-1.5)$ to divide the equation as follows:

$$
begin{align*}
-1.5| &=2 1 -27 -36
\ \
&= -3 3 36
\ \
&=2 -2 -24 0
end{align*}
$$

$$
2x^3+x^2-27x-36=(x+1.5)(2x^2-2x-24)
$$

$$
2x^3+x^2-27x-36=(x+1.5)(2x+6)(x-4)
$$

$$
color{#4257b2}text{(c)} 3x^4-19x^3+38x^2-24x
$$

Use the factor theorem to factor the cubic function as follows:

$$
f(1)=3-19+38-24 f(1)=-2
$$

$$
f(2)=48-152+152-48 f(2)=0
$$

$(x-2)$ is the factor, so use $(x=2)$ to divide the equation as follows:

$$
begin{align*}
2| &=3 -19 38 -24
\ \
&= 6 -26 24
\ \
&=3 -13 12 0
end{align*}
$$

$$
3x^4-19x^3+38x^2-24x=(x-2)(3x^3-13x^2-12x)
$$

$$
3x^4-19x^3+38x^2-24x=x(x-2)(3x^2-13x-12)
$$

$$
color{#4257b2}text{(d)} x^4+11x^3+36x^2+16x-64
$$

Use the factor theorem to factor the cubic function as follows:

$$
f(1)=1+11+36+16-64 f(1)=0
$$

$(x-1)$ is the factor, so use $(x=1)$ to divide the equation as follows:

$$
begin{align*}
1| &=1 11 36 16 -64
\ \
&= 1 12 48 64
\ \
&=1 12 48 64 0
end{align*}
$$

$$
x^4+11x^3+36x^2+16x-64=(x-1)(x^3+12x^2+48x+64)
$$

$$
text{color{Brown}(a) $(x+1)(x+2)(x-8)$ (b) $(x+1.5)(2x+6)(x-4)$
\ \
(c) $x(x-2)(3x^2-13x-12)$ (d) $(x-1)(x^3+12x^2+48x+64)$}
$$

Factor the following expressions

$$
color{#4257b2}text{(a)} 8x^3-10x^2-17x+10
$$

Use the factor theorem to factor the cubic function as follows:

$$
f(1)=8-10-17+10 f(1)=-9
$$

$$
f(2)=64-40-34+10 f(2)=0
$$

$(x-2)$ is the factor, so use $(x=2)$ to divide the equation as follows:

$$
begin{align*}
2| &=8 -10 -17 10
\ \
&= 16 12 -10
\ \
&=8 6 -5 0
end{align*}
$$

$$
8x^3-10x^2-17x+10=(x-2)(8x^2+6x-5)
$$

$$
8x^3-10x^2-17x+10=(x-2)(4x+5)(2x-1)
$$

$$
color{#4257b2}text{(b)} 2x^3+7x^2-7x-30
$$

Use the factor theorem to factor the cubic function as follows:

$$
f(1)=2+7-7-30 f(1)=-28
$$

$$
f(2)=16+28-14-30 f(2)=0
$$

$(x-2)$ is the factor, so use $(x=2)$ to divide the equation as follows:

$$
begin{align*}
2| &=2 7 -7 -30
\ \
&= 4 22 30
\ \
&=2 11 15 0
end{align*}
$$

$$
2x^3+7x^2-7x-30=(x-2)(2x^2+11x+15)
$$

$$
2x^3+7x^2-7x-30=(x-2)(2x+5)(x+3)
$$

$$
color{#4257b2}text{(c)} x^4-7x^3+9x^2+27x-54
$$

Use the factor theorem to factor the cubic function as follows:

$$
f(1)=1-7+9+27-54 f(1)=-24
$$

$$
f(2)=16-56+36+54-54 f(2)=-4
$$

$$
f(3)=81-189+81+81-54 f(3)=0
$$

$(x-3)$ is the factor, so use $(x=3)$ to divide the equation as follows:

$$
begin{align*}
3| &=1 -7 9 27 -54
\ \
&= 3 -12 -9 54
\ \
&=1 -4 -3 18 0
end{align*}
$$

$$
x^4-7x^3+9x^2+27x-54=(x-3)(x^3-4x^2-3x+18)
$$

$$
color{#4257b2}text{(d)} 4x^4+4x^3-35x^2-36x-9
$$

Use greatest common factor as follows:

$$
4x^4+4x^3-35x^2-35x-x-9
$$

$$
4x^3(x+1)-35x(x+1)-x-9
$$

$$
(x+1)(4x^3-35x)-x-9
$$

$$
x(x+1)(4x^2-35)-x-9
$$

$$
text{color{Brown}(a) $(x-2)(4x+5)(2x-1)$ (b) $(x-2)(2x+5)(x+3)$
\ \
(c) $(x-3)(x^3-4x^2-3x+18)$ (d) $x(x+1)(4x^2-35)-x-9$}
$$

Factor the following expression.

$$
color{#4257b2}text{(a)} 64x^3-27
$$

$$
[(4x)^3-3^3]=64x^3-27
$$

Use standard form $a^3-b^3=(a-b)(a^2+ab+b^2)$

$$
(4x)^3-3^3=(4x-3)(16x^2+12x+9)
$$

$$
color{#4257b2}text{(b)} 512x^3-125
$$

$$
[(8x)^3-5^3]=512x^3-125
$$

Use standard form $a^3-b^3=(a-b)(a^2+ab+b^2)$

$$
(8x)^3-5^3=(8x-5)(64x^2+40x+25)
$$

$$
color{#4257b2}text{(c)} 343x^3-1728
$$

$$
[(7x)^3-12^3]=343x^3-1728
$$

Use standard form $a^3-b^3=(a-b)(a^2+ab+b^2)$

$$
(7x)^3-12^3=(7x-12)(49x^2+84x+144)
$$

$$
color{#4257b2}text{(d)} 1331x^3-1
$$

$$
[(11x)^3-1^3]=1331x^3-1
$$

Use standard form $a^3-b^3=(a-b)(a^2+ab+b^2)$

$$
(11x)^3-1^3=(11x-1)(121x^2+11x+1)
$$

$$
text{color{Brown}(a) $(4x-3)(16x^2+12x+9)$
\ \
(b) $(8x-5)(64x^2+40x+25)$
\ \
(c) $(7x-12)(49x^2+84x+144)$
\ \
(d) $(11x-1)(121x^2+11x+1)$}
$$

Factor the following expression.

$$
color{#4257b2}text{(a)} 1000x^3+343
$$

$$
[(10x)^3+7^3]=1000x^3+343
$$

Use standard form $a^3+b^3=(a+b)(a^2-ab+b^2)$

$$
(10x)^3+7^3=(10x+7)(100x^2-70x+49)
$$

$$
color{#4257b2}text{(b)} 1728x^3+125
$$

$$
[(12x)^3+5^3]=1728x^3+125
$$

Use standard form $a^3+b^3=(a+b)(a^2-ab+b^2)$

$$
(12x)^3+5^3=(12x+5)(144x^2-60x+25)
$$

$$
color{#4257b2}text{(c)} 27x^3+1331
$$

$$
[(3x)^3+11^3]=27x^3+1331
$$

Use standard form $a^3+b^3=(a+b)(a^2-ab+b^2)$

$$
(3x)^3+11^3=(3x+11)(9x^2-33x+121)
$$

$$
color{#4257b2}text{(d)} 216x^3+2197
$$

$$
[(6x)^3+13^3]=216x^3+2197
$$

Use standard form $a^3+b^3=(a+b)(a^2-ab+b^2)$

$$
(6x)^3+13^3=(6x+13)(36x^2-78x+169)
$$

$$
text{color{Brown}(a) $(10x+7)(100x^2-70x+49)$
\ \
(b) $(12x+5)(144x^2-60x+25)$
\ \
(c) $(3x+11)(9x^2-33x+121)$
\ \
(d) $(6x+13)(36x^2-78x+169)$}
$$

Factor this equation $(x^6-y^6)$ for the following terms :

$$
text{color{#4257b2}(a) By treating it as difference of squares.}
$$

$$
x^6-y^6=(x^3)^2-(y^3)^2
$$

$$
(x^3)^2-(y^3)^2=(x^3-y^3)(x^3+y^3)
$$

$$
text{color{#4257b2}(b) By treating it as difference of cubes.}
$$

$$
x^6-y^6=(x^2)^3-(y^2)^3
$$

According the standard form $a^3+b^3=(a+b)(a^2-ab+b^2)$

$$
(x^2)^3-(y^2)^3=(x^2-y^2)(x^4+x^2y^2+y^4)
$$

$$
text{color{#4257b2}(c) Explain any similarities or differences in results.}
$$

The two results is different from each other because the difference is is no the same.

$$
text{color{Brown}(a) $(x^3)^2-(y^3)^2=(x^3-y^3)(x^3+y^3)$
\ \
(b) $(x^2)^3-(y^2)^3=(x^2-y^2)(x^4+x^2y^2+y^4)$
\ \
(c) Two results are different.}
$$