$textbf{The solution is}$ $y=sec x$.

$y=sec x$.

$sin dfrac{3pi}{2}=-1$

$cos pi=-1$

$tan dfrac{7pi}{4}=-1$

$csc dfrac{3pi}{2}=-1$

$sec 2pi=1$

$cot dfrac{3pi}{4}=-1$

$sec$ $textbf{has a different value}$.

$sec$ $textbf{has a different value}$.

$y=-12 cos(dfrac{5}{3}(x+dfrac{pi}{6}))+100$

$y=-12 cos(dfrac{5}{3}(dfrac{5pi}{4}+dfrac{pi}{6}))+100$

$y=108.5$

$y=108.5$

For $d=52$ (Feb 21)

$T(52)=-20cos (dfrac{2pi}{365}(52-10))+25$

$T(52)=10$

For $d=128$ (May 8),

$T(128)=-20cos (dfrac{2pi}{365}(128-10))+25$

$T(128)=33.9$

$textbf{average rate}$ = $dfrac{33.9-10}{128-52}=dfrac{23.9}{76}=0.31^circ C$ per day.

$0.31^circ C$ per day.

$dfrac{5pi}{8}$ radians;

$dfrac{5 cancel{pi}}{8} cancel{radians} times (dfrac{180^circ}{cancel{pi radians}})=112.5^circ$

$dfrac{2pi}{3}$ radians;

$dfrac{2 cancel{pi}}{3} cancel{radians} times (dfrac{180^circ}{cancel{pi radians}})=120^circ$

$dfrac{3pi}{5}$ radians;

$dfrac{3 cancel{pi}}{5} cancel{radians} times (dfrac{180^circ}{cancel{pi radians}})=112.5^circ$

So, from smallest to largest, the angles are $dfrac{3pi}{5}$, $110^circ$, $dfrac{5pi}{8}$, $113^circ$ and $dfrac{2pi}{3}$.

$dfrac{3pi}{5}$, $110^circ$, $dfrac{5pi}{8}$, $113^circ$ and $dfrac{2pi}{3}$.

To find the equivalent function we’ll use the following identity of transformation cosine to sine functions
$$sin left(alpha+frac{pi}{2}right)=cos (alpha).$$
We can of course read out $alpha = x + frac{pi}{8}$ as such we can get argument for sine as
$$alpha +frac{pi}{2}= x + frac{pi}{8}+frac{pi}{2} = x + frac{5pi}{8}$$
Thus the equivalent function is
$$y=sinleft(x+dfrac{5pi}{8} right).$$

$y=sinleft(x+dfrac{5pi}{8}right)$

Here we have triangle whose one angle is $90^circ$, and on triangle like this we can calculate value of trigonometric functions of its angles. So, according to definition of $tan$ function, we can calculate required value of $y$.

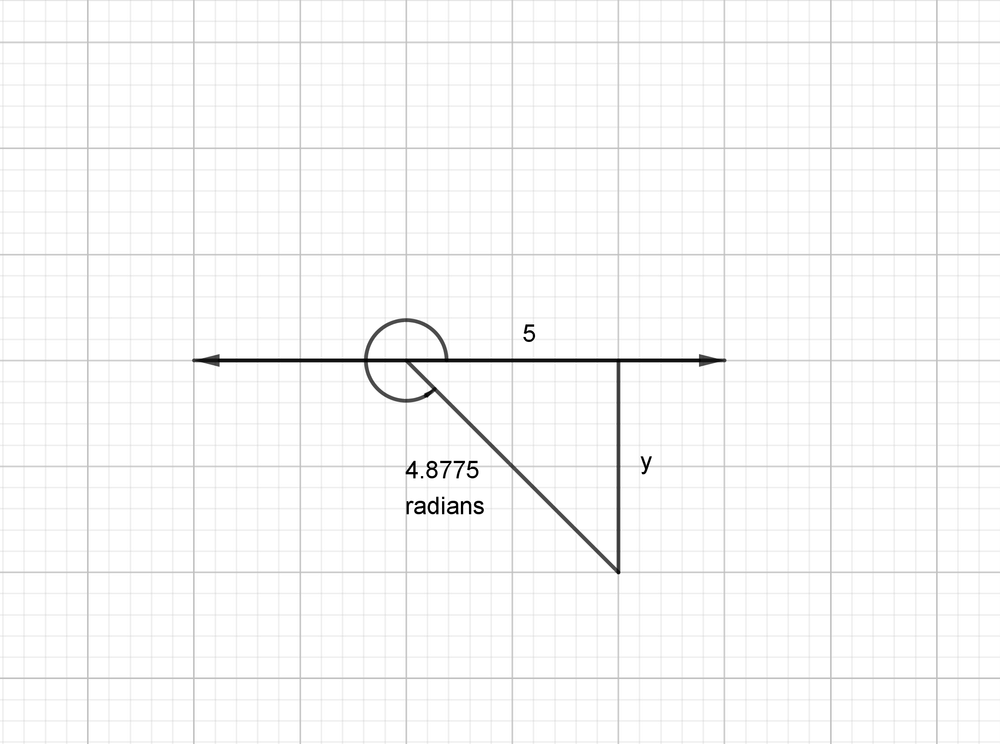
$2pi-4.8755=1.4077$

$tan(1.4077)=dfrac{y}{5}$

$y=5tan(1.4077)$

$y=-30$

$-30$

#### (a)

$$
-3cos(dfrac{pi}{12}x)+22
$$

#### (b)

For $t=0$ (sunrise)

$T(0)=-3cos(dfrac{pi}{12}0)+22$

$T(0)=19$.

For $t=6$:

$T(6)=-3cos(dfrac{pi}{12}6)+22$

$T(6)=22$

$textbf{average rate of change}$ = $dfrac{22-19}{6-0}=dfrac{3}{6}=0.5^circ C$ per hour.
#### (c)

$11 leq t leq 13$

For $t=11$ (5 p.m.)

$T(11)=-3cos(dfrac{pi}{12}11)+22$

$T(11)=24.9$

For $t=13$ (7 p.m.)

$T(13)=-3cos(dfrac{pi}{12}13)+22$

$T(13)=24.9$

$textbf{instaneous rate of change}$ = $dfrac{24.9-24.9}{13-11}=dfrac{0}{2}=0^circ C$ per hour.

(a) $-3cos(dfrac{pi}{12}x)+22$; (b) $0.5^circ C$; (c) $0^circ C$