All Solutions
Page 605: Closure Activity
If we solve quadratic equations which are in this fraction, we will get the following:
$$
dfrac{2x^2-5x-3}{3x^2-11x+6}=dfrac{2left(x+tfrac{1}{2} right)(x-3)}{3left(x-tfrac{2}{3} right)(x-3)}=dfrac{(2x+1)}{(3x-2)}cdotdfrac{x-3}{x-3}=dfrac{2x+1}{3x-2}cdot1=dfrac{2x+1}{3x-2}
$$
#### (b)
We will simplify this expression in the following way:
$$
dfrac{x^3-8}{4x^3-3x^2-10x}=dfrac{(x-2)(x^2+2x+4)}{x(4x^2-3x-10)}=dfrac{(x-2)(x^2+2x+4)}{x(4x+5)(x-2)}=dfrac{x^2+2x+4}{x(4x+5)}
$$
We will simplify the given expression in the following way:
$$
dfrac{x^2-x-6}{x^2-9}cdotdfrac{x^2+5x+6}{x^2+4x+4}=dfrac{(x-3)(x+2)}{(x-3)(x+3)}cdotdfrac{(x+3)(x+2)}{(x+2)^2}=1, x=pm 3, xne -2
$$
#### (b)
We will simplify this expression in the following way:
$$
dfrac{x^2-1}{x}cdotdfrac{2x^2+x}{x^2-2x+1}=dfrac{(x-1)(x+1)}{x}cdotdfrac{x(2x+1)}{(x-1)^2}=dfrac{(x+1)(2x+1)}{x-1}, xne 0, xne 1
$$
A(3,4,2)
$$
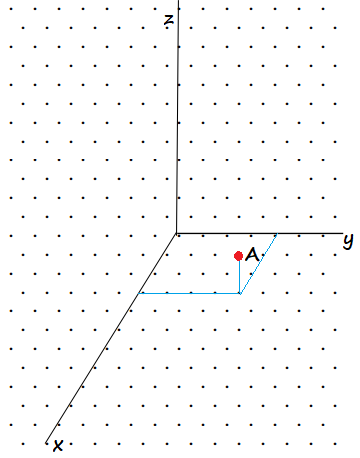
B(-2,3,0)
$$

2x+y-z=6
$$
$z=0,x=0Rightarrow y=6$
$x=0,y=0Rightarrow -z=6Rightarrow z=-6$
left(2m+dfrac{1}{m}right)^4
$$
$a=2m$
$b=dfrac{1}{m}=m^{-1}$
$(2m+m^{-1})^4$
$=_4C_0(2m)^4+_4C_1(2m)^3(m^{-1})^1+_4C_2(2m)^2(m^{-1})^2+_4C_3(2m)^1(m^{-1})^3+_4C_4(m^{-1})^4$
$=16m^4+dfrac{4!}{3!1!}(8m^3)(m^{-1})+dfrac{4!}{2!2!}(4m^2)(m^{-2})+dfrac{4!}{1!3!}(2m)(m^{-3})+m^{-4}$
$=16m^4+dfrac{3!cdot 4}{3!cdot 1}(8m^2)+dfrac{2!cdot 3cdot 4}{2!cdot 1cdot 2}(4)+dfrac{3!cdot 4}{1cdot 3!}(2m^{-2})+m^{-4}$
$=16m^4+32m^2+24+8m^{-2}+m^{-4}$
$=16m^4+32m^2+24+dfrac{8}{m^2}+dfrac{1}{m^4}$
$(a+b)^n=_nC_0a^n+_nC_qa^{n-1}b^1+_nC_2a^{n-2}b^2+…+_nC_{n-1}a^1b^{n-1}+_nC_nb^n$.
16m^4+32m^2+24+dfrac{8}{m^2}+dfrac{1}{m^4}
$$
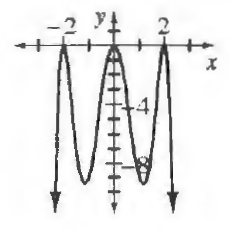
$x_2=0$
$$
x_3=2
$$
f(x)=a(x+2)^2x^2(x-2)^2
$$
$9a=-9$
$$
a=-1
$$
f(x)=-x^2(x-2)^2(x+2)^2
$$
f(x)=-x^2(x-2)^2(x+2)^2
$$
$A=4000$
$$
t=10
$$
$4000=3000e^{10r}$
$e^{10r}=dfrac{4000}{3000}$
$e^{10r}=dfrac{4}{3}$
$ln (e^{10r})=ln (dfrac{4}{3})$
$10r=ln (dfrac{4}{3})$
$r=dfrac{ln (dfrac{4}{3})}{10}$
$rapprox 0.0287=2.87%$
$A=4000$
$r=0.0275$
$$
n=4
$$
$4000=3000left(1+dfrac{0.0275}{4}right)^{4t}$
$dfrac{4000}{3000}=(1.006875)^{4t}$
$dfrac{4}{3}=(1.006875)^{4t}$
$ln (dfrac{4}{3})=ln (1.006875)^{4t}$
$ln (dfrac{4}{3})=4tln (1.006875)$
$t=dfrac{ln (dfrac{4}{3})}{4ln (1.006875)}$
$tapprox 10.5$ years
b) $10.5$ years
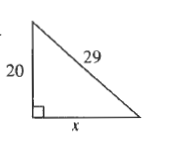
$x^2=841-400$
$x^2=441$
$x=sqrt{441}$
$$
x=21
$$
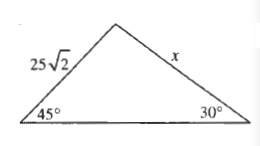
$25sqrt 2sin 45text{textdegree}=xsin 30text{textdegree}$
$x=dfrac{25sqrt 2cdot dfrac{sqrt 2}{2}}{dfrac{1}{2}}$
$x=dfrac{25}{dfrac{1}{2}}$
$x=25cdot 2$
$$
x=50
$$

$1369=400+x^2-40xcdot left(-dfrac{1}{2}right)$
$x^2+20x+400-1369=0$
$(x^2+20x+100)-1069=0$
$(x+10)^2=1069$
$x+10pmsqrt{1069}$
$x+10=pm 32.7$
$x+10=-32.7Rightarrow x_1=-42.7$
$x+10=32.7Rightarrow x_2=22.7$
b) $50$
c) $22.7$

$$
A(7,5)
$$
$$
k=3
$$
$y=a(x-h)^2+k$.
y=a(x-3)^2+3
$$
$16a+3=5$
$16a=2$
$a=dfrac{2}{16}$
$$
a=dfrac{1}{8}
$$
y=dfrac{1}{8}(x-3)^2+3
$$
y=dfrac{1}{8}(x-3)^2+3
$$

