All Solutions
Page 425: Closure Activity
$$
0=(x-2)^2-3
$$
Add 3 to both sides of the equation:
$$
3=(x-2)^2
$$
Take the square root of both sides of the equation:
$$
pm3=x-2
$$
Add 2 to both sides of the equation:
$$
5text{ or }-1=2pm 3 =x
$$
$$
0=(x-19)^2(x+14)
$$
Zero product property:
$$
x-19=0text{ or } x+14=0
$$
Solve each equation to $x$:
$$
x=19text{ or } x=-14
$$
b. $x=-14,19$
{color{#4257b2}text{a)}}
$$
Solution to this example is given below
$$
begin{align*}
&x^2-2xcdot :2+2^2-3&&boxed{text{Square formula}}\
&x^2-4x+4-3&&boxed{text{Multiply, and evaluate}}\
&x^2-4x+1&&boxed{text{Simplify}}\
x^2-4x+1&=0&&boxed{text{Simplify}}\
end{align*}
$$
Solve with the quadratic formula
$$
begin{align*}
x_{1,:2}&=frac{-bpm sqrt{b^2-4ac}}{2a}&&boxed{text{ Use quadratic formula}}\
x_{1,:2}&=frac{-left(-4right)pm sqrt{left(-4right)^2-4cdot :1cdot :1}}{2cdot :1}&&boxed{text{Substitute }1 text{ for }a, -4 text{ for } b, text{ and } 1 text{ for } c.} \
end{align*}
$$
First we solve $x_1$
$$
begin{align*}
x_1&=frac{-left(-4right)+sqrt{left(-4right)^2-4cdot :1cdot :1}}{2cdot :1}&&boxed{text{Simplify}}\
x_1&=frac{4+sqrt{left(-4right)^2-4cdot :1cdot :1}}{2cdot :1}&&boxed{text{Remove parentheses}}\
x_1&=frac{4+sqrt{16-4cdot :1cdot :1}}{2cdot :1}&&boxed{text{Evaluate: }(-4)^2=16}\
x_1&=frac{4+sqrt{16-4cdot :1cdot :1}}{2}&&boxed{text{Multiply the numbers: } 2cdot1=2}\
x_1&=frac{4+sqrt{16-4}}{2}&&boxed{text{Multiply the numbers: }4cdot1cdot1=4}\
x_1&=frac{4+sqrt{12}}{2}&&boxed{text{Subtract the numbers: }16-4=12}\
x_1&=frac{4+sqrt{2^2cdot3}}{2}&&boxed{text{Prime factorization of 12}}\
x_1&=frac{4+sqrt{2^2}sqrt{3}}{2}&&boxed{text{Apply radical rule}}\
end{align*}
$$
begin{align*}
x_1&=frac{4+2sqrt{3}}{2}&&boxed{text{Apply radical rule}}\
x_1&=frac{2cdot :2+2sqrt{3}}{2}&&boxed{text{Rewrite}}\
x_1&=frac{2left(2+sqrt{3}right)}{2}&&boxed{text{Factor out common term: }2}\
x_1&=color{#c34632}{2+sqrt3}&&boxed{text{Simplify}}\
end{align*}
$$
Second we solve $x_2$
$$
begin{align*}
x_2&=frac{-left(-4right)-sqrt{left(-4right)^2-4cdot :1cdot :1}}{2cdot :1}&&boxed{text{Simplify}}\
x_2&=frac{4-sqrt{left(-4right)^2-4cdot :1cdot :1}}{2cdot :1}&&boxed{text{Remove parentheses}}\
x_2&=frac{4-sqrt{16-4cdot :1cdot :1}}{2cdot :1}&&boxed{text{Evaluate: }(-4)^2=16}\
x_2&=frac{4-sqrt{16-4cdot :1cdot :1}}{2}&&boxed{text{Multiply the numbers: } 2cdot1=2}\
x_2&=frac{4-sqrt{16-4}}{2}&&boxed{text{Multiply the numbers: }4cdot1cdot1=4}\
x_2&=frac{4-sqrt{12}}{2}&&boxed{text{Subtract the numbers: }16-4=12}\
x_2&=frac{4-sqrt{2^2cdot3}}{2}&&boxed{text{Prime factorization of 12}}\
end{align*}
$$
begin{align*}
x_2&=frac{4-sqrt{2^2}sqrt{3}}{2}&&boxed{text{Apply radical rule}}\
x_2&=frac{4-2sqrt{3}}{2}&&boxed{text{Apply radical rule}}\
x_2&=frac{2cdot :2-2sqrt{3}}{2}&&boxed{text{Rewrite 4 as }2cdot2}\
x_2&=frac{2left(2-sqrt{3}right)}{2}&&boxed{text{Factor out common term: }2}\
x_2&=color{#c34632}{2-sqrt3}&&boxed{text{Simplify}}\\
&boxed{{color{#c34632}x_1= 2+sqrt3, x_2=2-sqrt3} }&&boxed{text{Final solution}}\
end{align*}
$$
$$
boxed{ color{#c34632} text{ }mathrm{Apply:radical:rule}:quad sqrt[n]{ab}=sqrt[n]{a}sqrt[n]{b} }
$$
$$
boxed{ color{#c34632} text{ }mathrm{Apply:radical:rule}:quad sqrt[n]{a^n}=a }
$$
{color{#4257b2}text{b)}}
$$
Solution to this example is given below
$$
begin{align*}
0&=left(x-19right)^2left(x+14right)&&boxed{text{Substitute 0 for }f(x)}\
left(x-19right)^2left(x+14right)&=0&&boxed{text{Switch sides}}\
end{align*}
$$
Using the zero factor principle
$$
begin{align*}
x-19&=0&&boxed{text{Simplify}}\
x-19+19&=0+19&&boxed{text{Add 19 to both sides}}\
x&=color{#c34632}{19}&&boxed{text{Simplify}}\\
end{align*}
$$
Using the zero factor principle
$$
begin{align*}
x+14&=0&&boxed{text{Simplify}}\
x+14-14&=0-14&&boxed{text{Subtract 14 from both sides}}\
x&=color{#c34632}{-14}&&boxed{text{Simplify}}\\
&boxed{{color{#c34632}x=19, x=-14} }&&boxed{text{Final solution}}\
end{align*}
$$
color{#4257b2} text{ a) }x_1=2+sqrt3, x_2=2-sqrt3
$$
$$
color{#4257b2} text{ b) }x=19, x=-14
$$
$$
f(x)=a(x+3)(x-2)(x-5)
$$
Evaluate at $x=1$ which should equal 56:
$$
56=a(1+3)(1-2)(1-5)
$$
Simplify
$$
56=16a
$$
Divide both sides of the equation by 16:
$$
dfrac{7}{2}=a
$$
Thus the function equation then becomes:
$$
f(x)=dfrac{7}{2}(x+3)(x-2)(x-5)
$$
f(x)=dfrac{7}{2}(x+3)(x-2)(x-5)
$$

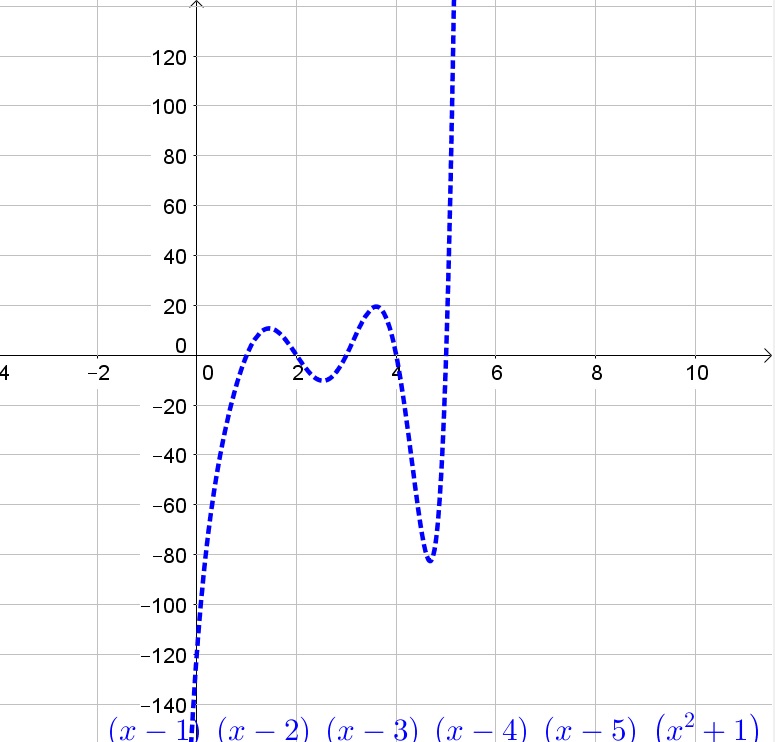
{color{#4257b2}text{a)}}
$$
First we solve $x$-intercepts
$$
begin{align*}
0&=xleft(2x+3right)left(2x-5right)&&boxed{text{Substitute 0 for }y}\
xleft(2x+3right)left(2x-5right)&=0&&boxed{text{Switch sides}}\
end{align*}
$$
Using the zero factor principle
$$
begin{align*}
x&=color{#c34632}{0}&&boxed{text{Simplify}}\
end{align*}
$$
Using the zero factor principle
$$
begin{align*}
2x+3&=0&&boxed{text{Simplify}}\
2x+3-3&=0-3&&boxed{text{Subtract 3 from both sides}}\
2x&=-3&&boxed{text{Simplify}}\
frac{2x}{2}&=frac{-3}{2}&&boxed{text{Divide both sides by 2}}\
x&=color{#c34632}{-frac{3}{2}}&&boxed{text{Simplify}}\
end{align*}
$$
Using the zero factor principle
$$
begin{align*}
2x-5&=0&&boxed{text{Simplify}}\
2x-5+5&=0+5&&boxed{text{Add 5 to both sides}}\
2x&=5&&boxed{text{Simplify}}\
frac{2x}{2}&=frac{5}{2}&&boxed{text{Divide both sides by 2}}\
x&=color{#c34632}{frac{5}{2}}&&boxed{text{Simplify}}\
end{align*}
$$
$$
begin{align*}
y&=0cdot left(2cdot :0+3right)left(2cdot :0-5right)&&boxed{text{Substitute 0 for } x}\
y&=0cdot left(0+3right)left(0-5right)&&boxed{text{Multiply the numbers: }2cdot0=0}\
y&=0cdot3(0-5)&&boxed{text{Calculate within parentheses}}\
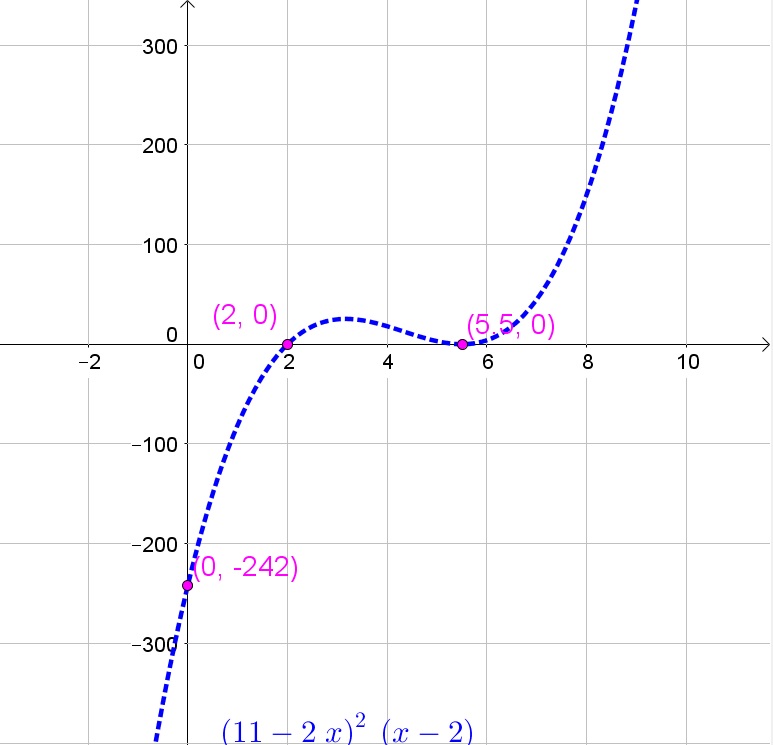
y&=color{#c34632}{0}&&boxed{text{Simplify}}\\
&boxed{{color{#c34632}x=0, x=-frac{3}{2}, x=frac{5}{2}, y=0} }&&boxed{text{Final solution}}\
end{align*}
$$
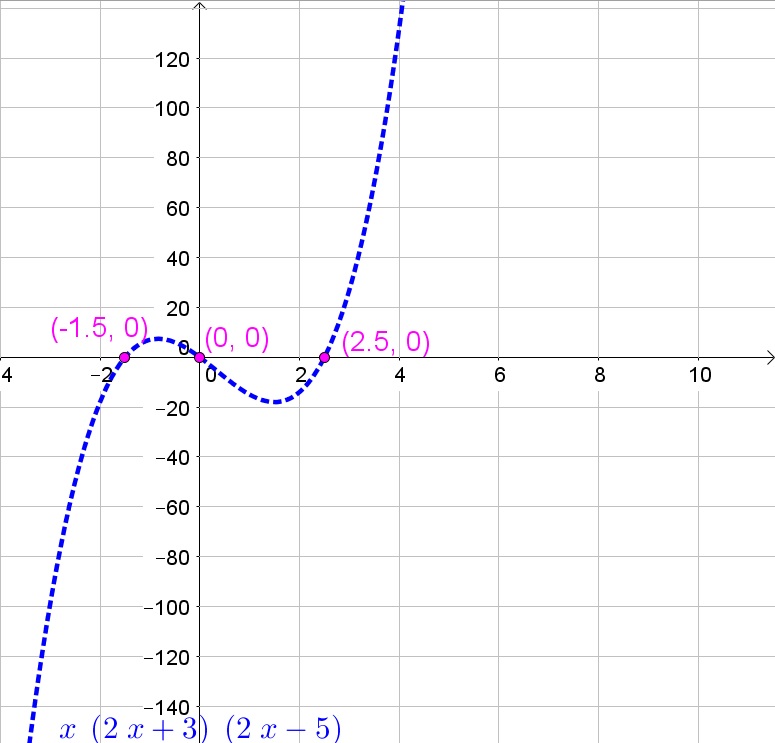
Graph of $boldsymbol{y=x(2x+3)(2x-5)}$
{color{#4257b2}text{b)}}
$$
First we solve $x$-intercepts
$$
begin{align*}
0&=left(11-2xright)^2left(x-2right)&&boxed{text{Substitute 0 for }y}\
left(11-2xright)^2left(x-2right)&=0&&boxed{text{Switch sides}}\
end{align*}
$$
Using the zero factor principle
$$
begin{align*}
11-2x&=0&&boxed{text{Simplify}}\
11-2x-11&=0-11&&boxed{text{Subtract 11 from both sides}}\
-2x&=-11&&boxed{text{Simplify}}\
frac{-2x}{-2}&=frac{-11}{-2}&&boxed{text{Divide both sides by }-2}\
x&=color{#c34632}{frac{11}{2}}&&boxed{text{Simplify}}\
end{align*}
$$
Using the zero factor principle
$$
begin{align*}
x-2&=0&&boxed{text{Simplify}}\
x-2+2&=0+2&&boxed{text{Add 2 to both sides}}\
x&=color{#c34632}{2}&&boxed{text{Simplify}}\
end{align*}
$$
Finally we solve $y$-intercepts
$$
begin{align*}
y&=left(11-2cdot :0right)^2left(0-2right)&&boxed{text{Substitute 0 for } x}\
y&=left(11-0right)^2left(0-2right)&&boxed{text{Multiply the numbers: }2cdot0=0}\
y&=11^2left(0-2right)&&boxed{text{Remove parentheses}}\
y&=121(-2)&&boxed{text{Evaluate: } 11^2=121}\
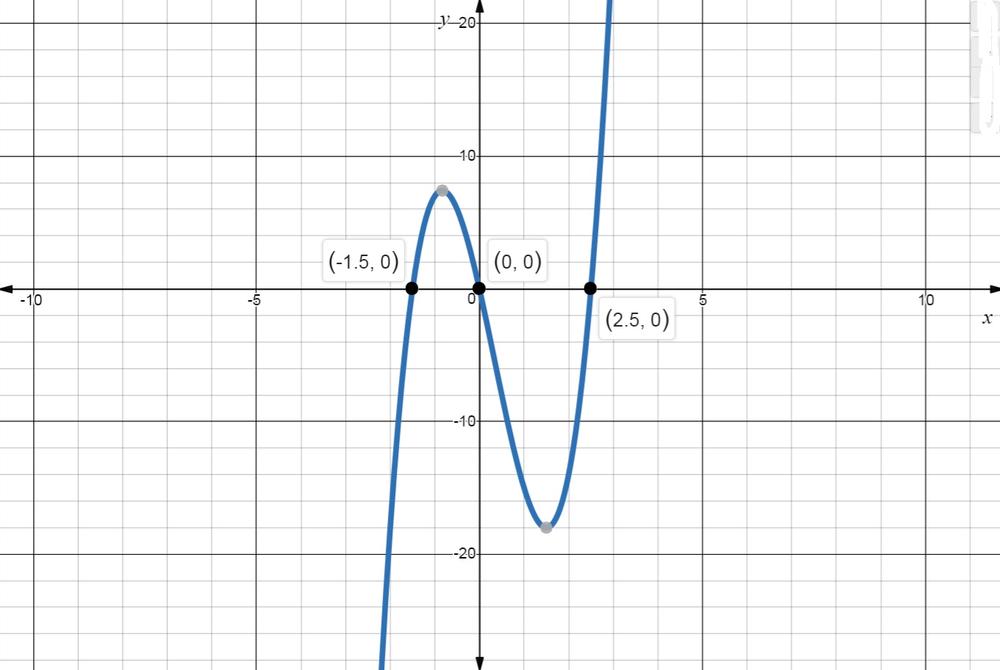
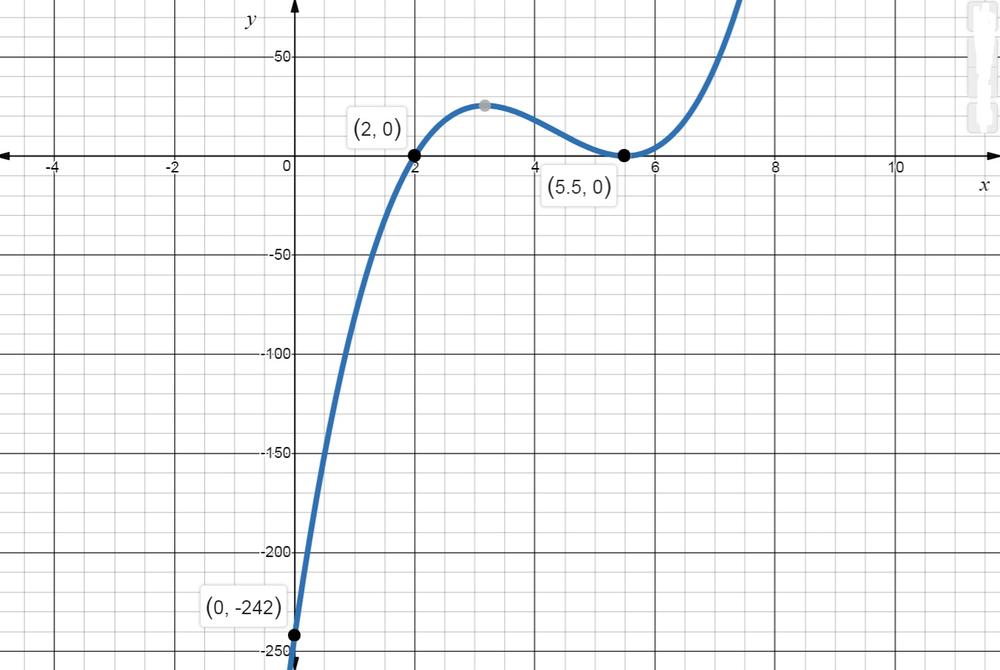
y&=color{#c34632}{-242}&&boxed{text{Simplify}}\
&boxed{{color{#c34632}x=frac{11}{2}, x=2, y=-242} }&&boxed{text{Final solution}}\
end{align*}
$$
Graph of $boldsymbol{y=(11-2x)^2(x-2)}$
color{#4257b2} text{ a) } x=0, x=-frac{3}{2}, x=frac{5}{2}, y=0
$$
$$
color{#4257b2} text{ b) }x=frac{11}{2}, x=2, y=-242
$$
$$
fleft(xright)=ax^2+bx+c
$$
$text{where $a, b$ and $c$ are real numbers and $aneq0$.}$
Quadratic function in the intercept form is:
$$
fleft(xright)=aleft(x-pright)left(x-qright)
$$
$$
begin{align*}
fleft(xright)&=aleft(x-pright)left(x-qright) && text{Intercept form.} \
fleft(xright)&=left(x-3iright)left(x-left(-3iright)right) && text{Substitute} a=1, p=3i, text{and} q=3i. \
fleft(xright)&=left(x-3iright)left(x+3iright) && text{Simplify.} \
fleft(xright)&=x^2+3xi-3xi-9i^2 && text{Distributive Property.} \
fleft(xright)&=x^2-9 cdot left(-1right) && text{Use} i^2=-1. \
fleft(xright)&=x^2+9 && text{Standard form.}
end{align*}
$$
$$
begin{align*}
fleft(xright)&=aleft(x-pright)left(x-qright) && text{Intercept form.} \
fleft(xright)&=left(x-left(2+sqrt{3}right)right)left(x-left(2-sqrt{3}right)right) && text{Substitute} a=1, p=2+sqrt{3}, \
&text{} && text{and} q=2-sqrt{3}. \
fleft(xright)&=left(x-2-sqrt{3}right)left(x-2+sqrt{3}right) && text{Simplify.} \
fleft(xright)&=x^2-2x+sqrt{3}x-2x+4-2sqrt{3}-sqrt{3}x+2sqrt{3}-3 && text{Distributive Property.} \
fleft(xright)&=x^2-4x+1 && text{Standard form.}
end{align*}
$$
$textbf{b.}$ $x^2-4x+1=0$
$$
text{Set $y=left(x+3right)^3-1$, and solve for $x$ in term of $y$.}
$$
$$
begin{align*}
y&=left(x+3right)^3-1 && text{Substitute} y text{for} fleft(xright). \
y+1&=left(x+3right)^3 && text{Add} 1 text{to both sides.} \
sqrt[3]{y+1}&=x+3 && text{cubic root of each side.} \
sqrt[3]{y+1}-3&=x && text{Subtract} 3 text{from both sides.} \
f^{-1}left(yright)&=sqrt[3]{y+1}-3 && text{Substitute} f^{-1}left(yright) text{for} x. \
end{align*}
$$

The inverse is a function because it passes the Vertical Line Test (any vertical line intersects
the graph in at most one point).
f(x)=(x+3)^3-1
$$
$x=(y+3)^3-1$
$x+1=(y+3)^3$
$y+3=sqrt[3]{x+1}$
$y=sqrt[3]{x+1}-3$
$$
f^{-1}(x)=sqrt[3]{x+1}-3
$$
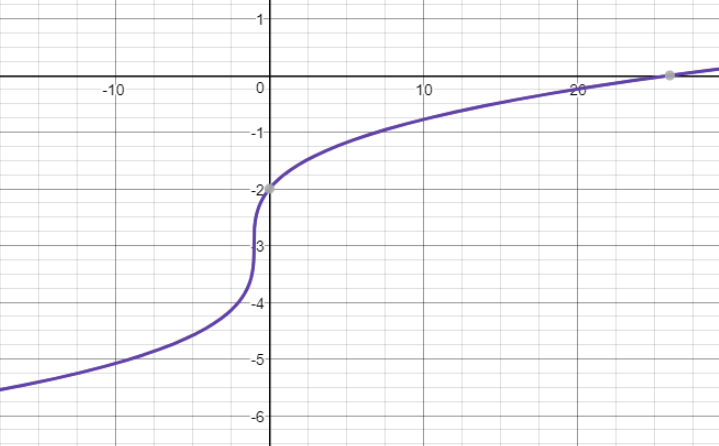
b) See graph
c) Yes
2(3^x)=40.8
$$
$$
3^x=20.4
$$
$xlog (3)=log (20.4)$
$x=dfrac{log (20.4)}{log (3)}$
$$
xapprox 2.745
$$
3x^4=27
$$
$x^4=9$
$x^4-9=0$
$(x^2-3)(x^2+3)=0$
$$
(x-sqrt 3)(x+sqrt 3)(x-sqrt 3i)(x+sqrt 3i)=0
$$
$x+sqrt 3=0Rightarrow x_2=-sqrt 3approx -1.732$
$x-sqrt 3i=0Rightarrow x_3=sqrt 3iapprox1.732i$
$x+sqrt 3i=0Rightarrow x_4=-sqrt 3iapprox-1.732i$
log_5 (2x+1)=3
$$
$$
2x+1=125
$$
$2x=124$
$x=dfrac{124}{2}$
$$
x=62
$$
log (x)+log (2x)=5
$$
$$
log (2x^2)=5
$$
$$
2x^2=100,000
$$
$x^2=50,000$
$x=pmsqrt{50,000}$
$$
xapprox pm223.607
$$
x=223.607
$$
b) $-sqrt 3, sqrt 3, -sqrt 3i, sqrt 3i$
c) $62$
d) $223.607$
$B(3,20.125)$
$$
y=20
$$
y=ab^x+k
$$
k=20
$$
begin{cases}
ab^1+20=22\
ab^3+20=20.125
end{cases}
$$
begin{cases}
ab=22-20\
ab^3=20.125-20
end{cases}
$$
$$
begin{cases}
ab=2\
ab^3=0.125
end{cases}
$$
$dfrac{ab^3}{ab}=dfrac{0.125}{2}$
$b^2=0.0625$
$b=0.25$
$a(0.25)=2$
$a=dfrac{2}{0.25}$
$$
a=8
$$
y=8(0.25)^x+20
$$
y=8(0.25)^x+20
$$

$dfrac{12}{sin 50text{textdegree}}=dfrac{8}{sin Z}$
$12sin Z=8sin 50text{textdegree}$
$sin Z=dfrac{8sin 50text{textdegree}}{12}=dfrac{8cdot 0.76604444}{12}$
$approx0.5107$
$sin^{-1} 0.5107approx 30.71text{textdegree}$
$$
Z=30.71text{textdegree}
$$
Z=30.71text{textdegree}
$$

