#### (b)

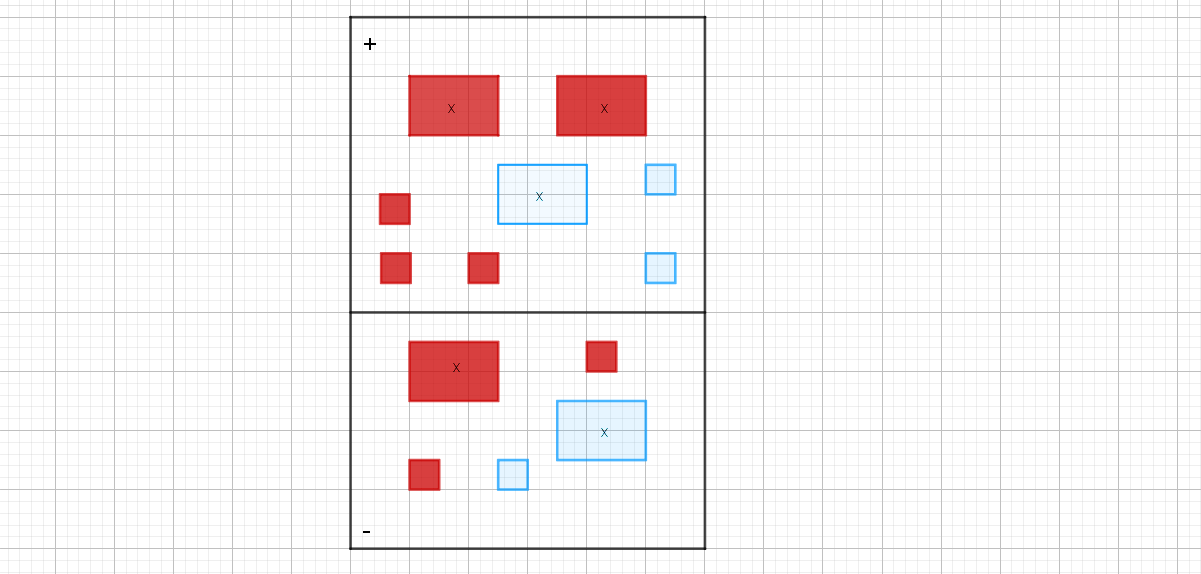
An expression for the tiles as they appear is following:

$$
x+x-x+1+1+1-1-(x-x+1+1-1)
$$

#### (c)

Circled all of the zeos that we can find to simplify the expression are:

$$
x+boxed{x-x}+1+1+boxed{1-1}-(boxed{x-x}+1+boxed{1-1})
$$

#### (d)

The completely simplified expression is following:

$$
x+1
$$

(a) You can use online tile algebra tools; (b) $x+x-x+1+1+1-1-(x-x+1+1-1)$; (c) $4$ groups; (d) $x+1$

Expression on the left side is:

$$
x+x+1+1+1-1-(x+x-1-1)=4
$$

Expression on the right side is:

$$
x+x-x+1+1-(x+x-x+1-1-1)=3
$$

Conclusion is that $4>3$, so, expression on the left side is greater.

$$
{color{#4257b2}text{a)}}
$$

$$
begin{align*}
3+7x-left(2+9xright)& {=}quad : 3+7x-2-9x\
&=7x-9x-2+3\
&=-2x-2+3\
&={color{#c34632}-2x+1}
end{align*}
$$

$$
{color{#4257b2}text{b)}}
$$

$$
begin{align*}
6-left(3x-4right)+7x-11& {=}quad : 6-3x+4+7x-11\
&=-3x+7x+4+6-11\
&=4x+4+6-11\
&={color{#c34632}4x-1}
end{align*}
$$

$$
color{#4257b2} text{ a) }-2x+1
$$

$$
color{#4257b2} text{ b) }4x-1
$$

$$
text{ }
$$

$$
text{ }
$$

$$
text{ }
$$

$$
text{ }
$$

$$
text{ }
$$

We need to simplify each expression below:

$$
color{#4257b2}text{(a)} 6^2-(5-4)+2(8-2^2)+8
$$

Evaluate the exponent as follows:

$$
36-(5-4)+2(8-4)+8
$$

Simplify the expression as follows:

$$
36-1+(2cdot4)+8
$$

Multiply from the left the the right as follows:

$$
36-1+8+8 text{Simplify} =51
$$

$$
color{#4257b2}text{(b)} dfrac{2(9-6)^2}{18}
$$

Evaluate the exponent as follows:

$$
dfrac{2(3)^2}{18}=dfrac{2cdot9}{18}
$$

Multiply from the left the the right as follows:

$$
dfrac{18}{18}=1
$$

$$
text{color{#4257b2}(a) $51$ (b) $1$}
$$

We need to evaluate the following expression.

$$
color{#4257b2}6x-(3y+7)-xy text{When} x=5, y=3
$$

Substitute the values of $x$ and $y$ as follows:

$$
(6 cdot 5)-[(3 cdot3)+7]-(5 cdot3)
$$

Multiply from the left to the right to simplify as follows:

$$
30-(9+7)-15=30-16-15
$$

Simplify as follows:

$$
30-31=-1
$$

$$
text{color{#4257b2}Large$-1$}
$$

$$
begin{align*}
& text{Group like terms}\
&3x^2-8x^2+4x-y^2+y^2-5y+10-8+3\\
&= -5x^2+4x-y^2+y^2-5y+10-8+3 tag{Add similar elements} \
&= -5x^2+4x-5y+10-8+3 tag{Add similar elements} \
&=boxed{{color{#c34632} -5x^2+4x+5-5y } }
end{align*}
$$

$$
color{#4257b2} text{}-5x^2+4x+5-5y
$$

We need to Simplify the following expression an evaluate it at the given values.

$$
color{#4257b2}3x^2-5x-4+xy-(2xy+2x^2) text{When} x=-1, y=6
$$

$$
3x^2-5x-4+xy-2xy-2x^2
$$

Add tiles to groups like terms as follows:

$$
(3x^2-2x^2)+-5x+(xy-2xy)-4
$$

$$
x^2-5x-xy-4
$$

Substitute the values of $x=-1, y=6$ in the function as follows:

$$
(-1)^2-(5cdot-1)-(-1cdot6)-4=1-(-5)-(-6)-4=1+5+6-4=8
$$

$x^2-5x-xy-4=8$

$$
begin{align*}
&text{Expand}\
&-3x+6=2x-9\\
&-3x+6-6=2x-9-6 tag{Subtract 6 from both sides} \
&-3x=2x-15 tag{Simplify}\
&-3x-2x=2x-15-2x tag{Subtract 2x from both sides}\
&-5x=-15 tag{Simplify}\
&frac{-5x}{-5}=frac{-15}{-5} tag{Divide both sides by -5}\\
&boxed{{color{#c34632} x=3 } }
end{align*}
$$

$$
begin{align*}
&text{We check the given equation}\
&2-(3* 3-4)=2*3-9\\
&2-5=6-9 tag{Simplify} \
&-3=-3 tag{Simplify}\
end{align*}
$$

$$
color{#965501} text{This equation is correct}
$$

$$
color{#4257b2} text{} x=3
$$

We need to solve the following equations as follows:

$$
color{#4257b2}text{(a)} 1-2-(-2x)=-3-x-(2+x)
$$

Change subtraction to adding the opposite as follows:

$$
1-2+2x=-3-x-2-x
$$

Isolate the variables on the left side as follows:

$$
2x+x+x=-3-2+2-1 4x=-4
$$

$$
x=dfrac{-4}{4} x=-1
$$

$text{color{#4257b2}Check:}$ substitute the value of $x=dfrac{1}{2}$ in the equation as follows:

$$
1-2-(-2cdot-1)=-3-(-1)-(2+(-1)) 1-2-(2)=-3+1-(2-1)
$$

$$
1-2-2=-3+1-2+1 -3=-3
$$

Since the left side is equal the right side, so the answer is correct.

$$
color{#4257b2}text{(b)} 1-3-x-(3-1)=x-1-x-(3+x)
$$

Change subtraction to adding the opposite as follows:

$$
1-3-x-3+1=x-1-x-3-x
$$

Isolate the variables on the left side as follows:

$$
-x-x+x+x=-1-3-1+3+3-1 -2x+2x=-6+6
$$

$$
0=0 text{No solution}
$$

(a) $x=-1$ (b) No solution

We need to solve the following equations as follows:

$$
color{#4257b2}text{(a)} -2x+2=-8
$$

Isolate the variables on the left side as follows:

$$
-2x=-8-2 -2x=-10
$$

Divided both sides of equation by $-2$ as follows.

$$
left(dfrac{-2}{-2}right) x=dfrac{-10}{-2} x=5
$$

$text{color{#4257b2}Check:}$ substitute the value of $x=5$ in the equation as follows:

$$
(-2cdot5)+2=-8 -10+2=-8 -8=-8
$$

Since the left side is equal the right side, so the answer is correct.

$$
color{#4257b2}text{(b)} 4x-2+x=2x+8+3x
$$

Isolate the variables on the left side as follows:

$$
4x+x-2x-3x=8+2 5x-5x=10
$$

$$
0=10 text{No solution}
$$

(a) $x=5$ (b) No solution